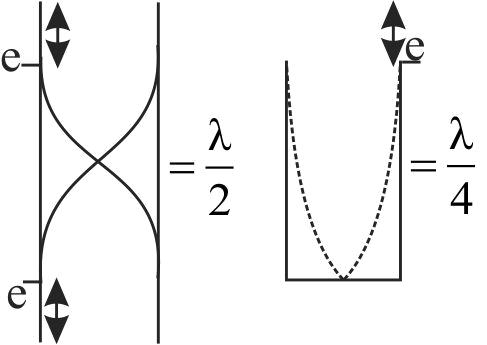
354879 A tube of diameter \(d\) and of length \(l\) unit is open at both ends. Its fundamental frequency of resonance is found to be \(f_{1}\). The velocity of sound in air is \(330\;m/\sec \). One endof tube is now closed. The lowest frequency of resonance of tube is \(f_{2}\). Taking into consideration of end correction, \(\dfrac{f_{2}}{f_{1}}\) is :
354880 In the experiment for the determination of the speed of sound in air using the resonance column method, the length of the air column that resonates in the fundamental mode, with a tuning fork is \(0.1\;m\). When this length is changed to\(0.35\;m\), the same tuning fork resonates with the first overnote. Calculate the end correction
354879 A tube of diameter \(d\) and of length \(l\) unit is open at both ends. Its fundamental frequency of resonance is found to be \(f_{1}\). The velocity of sound in air is \(330\;m/\sec \). One endof tube is now closed. The lowest frequency of resonance of tube is \(f_{2}\). Taking into consideration of end correction, \(\dfrac{f_{2}}{f_{1}}\) is :
354880 In the experiment for the determination of the speed of sound in air using the resonance column method, the length of the air column that resonates in the fundamental mode, with a tuning fork is \(0.1\;m\). When this length is changed to\(0.35\;m\), the same tuning fork resonates with the first overnote. Calculate the end correction
354879 A tube of diameter \(d\) and of length \(l\) unit is open at both ends. Its fundamental frequency of resonance is found to be \(f_{1}\). The velocity of sound in air is \(330\;m/\sec \). One endof tube is now closed. The lowest frequency of resonance of tube is \(f_{2}\). Taking into consideration of end correction, \(\dfrac{f_{2}}{f_{1}}\) is :
354880 In the experiment for the determination of the speed of sound in air using the resonance column method, the length of the air column that resonates in the fundamental mode, with a tuning fork is \(0.1\;m\). When this length is changed to\(0.35\;m\), the same tuning fork resonates with the first overnote. Calculate the end correction
354879 A tube of diameter \(d\) and of length \(l\) unit is open at both ends. Its fundamental frequency of resonance is found to be \(f_{1}\). The velocity of sound in air is \(330\;m/\sec \). One endof tube is now closed. The lowest frequency of resonance of tube is \(f_{2}\). Taking into consideration of end correction, \(\dfrac{f_{2}}{f_{1}}\) is :
354880 In the experiment for the determination of the speed of sound in air using the resonance column method, the length of the air column that resonates in the fundamental mode, with a tuning fork is \(0.1\;m\). When this length is changed to\(0.35\;m\), the same tuning fork resonates with the first overnote. Calculate the end correction
354879 A tube of diameter \(d\) and of length \(l\) unit is open at both ends. Its fundamental frequency of resonance is found to be \(f_{1}\). The velocity of sound in air is \(330\;m/\sec \). One endof tube is now closed. The lowest frequency of resonance of tube is \(f_{2}\). Taking into consideration of end correction, \(\dfrac{f_{2}}{f_{1}}\) is :
354880 In the experiment for the determination of the speed of sound in air using the resonance column method, the length of the air column that resonates in the fundamental mode, with a tuning fork is \(0.1\;m\). When this length is changed to\(0.35\;m\), the same tuning fork resonates with the first overnote. Calculate the end correction