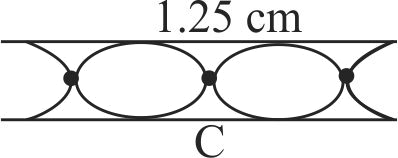
354882 A massless rod is suspended by two identical strings \(AB\) and \(CD\) of equal length. A block of mass \(m\) is suspended from point \(O\) such that \(BO\) is equal to ' \(x\) 'Further, it is observed that the frequency of \(1^{\text {st }}\) harmonic (fundamental frequency) in \(AB\) is equal to \(2^{\text {nd }}\) harmonic frequency in \(CD\). Then, length of \(BO\) is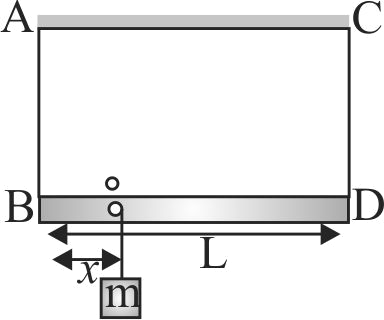

354883 If two antinodes are observed on either side of \(C\), the frequency of the mode in which the rod is vibrating will be
\(\left( {Y = 2 \times {{10}^{11}}\;N/{m^2},d = 8 \times {{10}^3}\;kg/{m^2}} \right)\)

354884 A \(100\,cm\) long steel rod is clamped at its middle. The fundamental frequency of longitudinal vibrations of the rod is \(2.53\,kHz\). What is the speed of sound in steel?

354885 Assertion :
The speed of sound in solids is maximum though their density is large.
Reason :
The coefficient of elasticity of solid is large.

354882 A massless rod is suspended by two identical strings \(AB\) and \(CD\) of equal length. A block of mass \(m\) is suspended from point \(O\) such that \(BO\) is equal to ' \(x\) 'Further, it is observed that the frequency of \(1^{\text {st }}\) harmonic (fundamental frequency) in \(AB\) is equal to \(2^{\text {nd }}\) harmonic frequency in \(CD\). Then, length of \(BO\) is

354883 If two antinodes are observed on either side of \(C\), the frequency of the mode in which the rod is vibrating will be
\(\left( {Y = 2 \times {{10}^{11}}\;N/{m^2},d = 8 \times {{10}^3}\;kg/{m^2}} \right)\)

354884 A \(100\,cm\) long steel rod is clamped at its middle. The fundamental frequency of longitudinal vibrations of the rod is \(2.53\,kHz\). What is the speed of sound in steel?

354885 Assertion :
The speed of sound in solids is maximum though their density is large.
Reason :
The coefficient of elasticity of solid is large.

354882 A massless rod is suspended by two identical strings \(AB\) and \(CD\) of equal length. A block of mass \(m\) is suspended from point \(O\) such that \(BO\) is equal to ' \(x\) 'Further, it is observed that the frequency of \(1^{\text {st }}\) harmonic (fundamental frequency) in \(AB\) is equal to \(2^{\text {nd }}\) harmonic frequency in \(CD\). Then, length of \(BO\) is

354883 If two antinodes are observed on either side of \(C\), the frequency of the mode in which the rod is vibrating will be
\(\left( {Y = 2 \times {{10}^{11}}\;N/{m^2},d = 8 \times {{10}^3}\;kg/{m^2}} \right)\)

354884 A \(100\,cm\) long steel rod is clamped at its middle. The fundamental frequency of longitudinal vibrations of the rod is \(2.53\,kHz\). What is the speed of sound in steel?

354885 Assertion :
The speed of sound in solids is maximum though their density is large.
Reason :
The coefficient of elasticity of solid is large.

354882 A massless rod is suspended by two identical strings \(AB\) and \(CD\) of equal length. A block of mass \(m\) is suspended from point \(O\) such that \(BO\) is equal to ' \(x\) 'Further, it is observed that the frequency of \(1^{\text {st }}\) harmonic (fundamental frequency) in \(AB\) is equal to \(2^{\text {nd }}\) harmonic frequency in \(CD\). Then, length of \(BO\) is

354883 If two antinodes are observed on either side of \(C\), the frequency of the mode in which the rod is vibrating will be
\(\left( {Y = 2 \times {{10}^{11}}\;N/{m^2},d = 8 \times {{10}^3}\;kg/{m^2}} \right)\)

354884 A \(100\,cm\) long steel rod is clamped at its middle. The fundamental frequency of longitudinal vibrations of the rod is \(2.53\,kHz\). What is the speed of sound in steel?

354885 Assertion :
The speed of sound in solids is maximum though their density is large.
Reason :
The coefficient of elasticity of solid is large.