368099
Two ideal slits \(S_{1}\) and \(S_{2}\) are at a distance \(d\) apart, and illuminated by light of wavelength \(\lambda\) passing through an ideal source slit \(S\) placed on the line through \(S_{2}\) as shown. The distance between the planes of slits and the source slit is \(D\). A screen is held at a distance \(D\) from the plane of the slits. The minimum value of \(d\) for which there is darkness at \(O\) is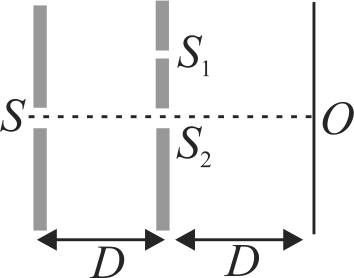
368099
Two ideal slits \(S_{1}\) and \(S_{2}\) are at a distance \(d\) apart, and illuminated by light of wavelength \(\lambda\) passing through an ideal source slit \(S\) placed on the line through \(S_{2}\) as shown. The distance between the planes of slits and the source slit is \(D\). A screen is held at a distance \(D\) from the plane of the slits. The minimum value of \(d\) for which there is darkness at \(O\) is
368099
Two ideal slits \(S_{1}\) and \(S_{2}\) are at a distance \(d\) apart, and illuminated by light of wavelength \(\lambda\) passing through an ideal source slit \(S\) placed on the line through \(S_{2}\) as shown. The distance between the planes of slits and the source slit is \(D\). A screen is held at a distance \(D\) from the plane of the slits. The minimum value of \(d\) for which there is darkness at \(O\) is
368099
Two ideal slits \(S_{1}\) and \(S_{2}\) are at a distance \(d\) apart, and illuminated by light of wavelength \(\lambda\) passing through an ideal source slit \(S\) placed on the line through \(S_{2}\) as shown. The distance between the planes of slits and the source slit is \(D\). A screen is held at a distance \(D\) from the plane of the slits. The minimum value of \(d\) for which there is darkness at \(O\) is