367794 Three waves of equal frequency having amplitudes \(10\mu m,4\mu m,7\mu m\) arrive at a given point with successive phase difference of \(\frac{\pi }{2}\), the amplitude of the resulting wave \(\left( {in\,\,\mu m} \right)\) s given by

367795 Two identical light waves, propagating in the same direction, have a phase difference \(\delta \). After they superimpose, the intensity of the resulting wave will be proportional to

367796 Two coherent light sources \({A}\) and \({B}\) are at a distance \(3 \lambda\) from each other \((\lambda=\) wavelength \()\). The distance from \({A}\) on the \(+{X}\)-axis at which the first constructive interference is found to be \({N} \lambda\). What is the value of \({N}\) ?

367797 The coherent waves each of intensity \(I_{0}\) produce interference pattern. The resultant intensity at the point of observation will be: (given \(\phi\) is the phase difference at the instant of arriving at that point)

367798 Two beams of light having intensities \(I\) and \(4I\) interfere to produce a fringe pattern on a screen.
The phase difference between the beams is \(\frac{\pi }{2}\) at point \(A\) and \(\pi \) at point \(B\). Then the difference between the resulting intensities at \(A\) and \(B\) is

367794 Three waves of equal frequency having amplitudes \(10\mu m,4\mu m,7\mu m\) arrive at a given point with successive phase difference of \(\frac{\pi }{2}\), the amplitude of the resulting wave \(\left( {in\,\,\mu m} \right)\) s given by

367795 Two identical light waves, propagating in the same direction, have a phase difference \(\delta \). After they superimpose, the intensity of the resulting wave will be proportional to

367796 Two coherent light sources \({A}\) and \({B}\) are at a distance \(3 \lambda\) from each other \((\lambda=\) wavelength \()\). The distance from \({A}\) on the \(+{X}\)-axis at which the first constructive interference is found to be \({N} \lambda\). What is the value of \({N}\) ?

367797 The coherent waves each of intensity \(I_{0}\) produce interference pattern. The resultant intensity at the point of observation will be: (given \(\phi\) is the phase difference at the instant of arriving at that point)

367798 Two beams of light having intensities \(I\) and \(4I\) interfere to produce a fringe pattern on a screen.
The phase difference between the beams is \(\frac{\pi }{2}\) at point \(A\) and \(\pi \) at point \(B\). Then the difference between the resulting intensities at \(A\) and \(B\) is

367794 Three waves of equal frequency having amplitudes \(10\mu m,4\mu m,7\mu m\) arrive at a given point with successive phase difference of \(\frac{\pi }{2}\), the amplitude of the resulting wave \(\left( {in\,\,\mu m} \right)\) s given by

367795 Two identical light waves, propagating in the same direction, have a phase difference \(\delta \). After they superimpose, the intensity of the resulting wave will be proportional to

367796 Two coherent light sources \({A}\) and \({B}\) are at a distance \(3 \lambda\) from each other \((\lambda=\) wavelength \()\). The distance from \({A}\) on the \(+{X}\)-axis at which the first constructive interference is found to be \({N} \lambda\). What is the value of \({N}\) ?

367797 The coherent waves each of intensity \(I_{0}\) produce interference pattern. The resultant intensity at the point of observation will be: (given \(\phi\) is the phase difference at the instant of arriving at that point)

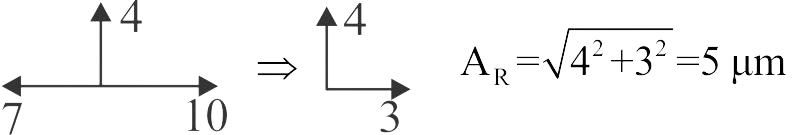
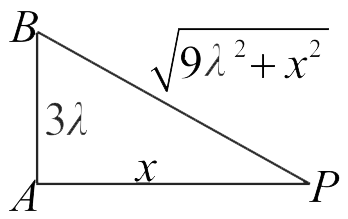
367798 Two beams of light having intensities \(I\) and \(4I\) interfere to produce a fringe pattern on a screen.
The phase difference between the beams is \(\frac{\pi }{2}\) at point \(A\) and \(\pi \) at point \(B\). Then the difference between the resulting intensities at \(A\) and \(B\) is

367794 Three waves of equal frequency having amplitudes \(10\mu m,4\mu m,7\mu m\) arrive at a given point with successive phase difference of \(\frac{\pi }{2}\), the amplitude of the resulting wave \(\left( {in\,\,\mu m} \right)\) s given by

367795 Two identical light waves, propagating in the same direction, have a phase difference \(\delta \). After they superimpose, the intensity of the resulting wave will be proportional to

367796 Two coherent light sources \({A}\) and \({B}\) are at a distance \(3 \lambda\) from each other \((\lambda=\) wavelength \()\). The distance from \({A}\) on the \(+{X}\)-axis at which the first constructive interference is found to be \({N} \lambda\). What is the value of \({N}\) ?

367797 The coherent waves each of intensity \(I_{0}\) produce interference pattern. The resultant intensity at the point of observation will be: (given \(\phi\) is the phase difference at the instant of arriving at that point)

367798 Two beams of light having intensities \(I\) and \(4I\) interfere to produce a fringe pattern on a screen.
The phase difference between the beams is \(\frac{\pi }{2}\) at point \(A\) and \(\pi \) at point \(B\). Then the difference between the resulting intensities at \(A\) and \(B\) is

367794 Three waves of equal frequency having amplitudes \(10\mu m,4\mu m,7\mu m\) arrive at a given point with successive phase difference of \(\frac{\pi }{2}\), the amplitude of the resulting wave \(\left( {in\,\,\mu m} \right)\) s given by

367795 Two identical light waves, propagating in the same direction, have a phase difference \(\delta \). After they superimpose, the intensity of the resulting wave will be proportional to

367796 Two coherent light sources \({A}\) and \({B}\) are at a distance \(3 \lambda\) from each other \((\lambda=\) wavelength \()\). The distance from \({A}\) on the \(+{X}\)-axis at which the first constructive interference is found to be \({N} \lambda\). What is the value of \({N}\) ?

367797 The coherent waves each of intensity \(I_{0}\) produce interference pattern. The resultant intensity at the point of observation will be: (given \(\phi\) is the phase difference at the instant of arriving at that point)

367798 Two beams of light having intensities \(I\) and \(4I\) interfere to produce a fringe pattern on a screen.
The phase difference between the beams is \(\frac{\pi }{2}\) at point \(A\) and \(\pi \) at point \(B\). Then the difference between the resulting intensities at \(A\) and \(B\) is