366381 \(1\;kg\) of water at \(100^\circ C\) is converted into steam at \(100^\circ C\) by boiling at atmospheric pressure. The volume of water changes from \(1.00 \times {10^{ - 3}}\;{m^3}\) as a liquid to \(1.671\;{m^3}\) as steam. The change in internal energy of the system during the process will be (Given latent heat of vaporisation \( = 2257\;kJ/kg\), Atmospheric pressure \( = 1 \times {10^5}\;Pa\))
366382
In a calorimeter there is 5 \(kg\) of water at \(0^{\circ} {C}\) mixed to an unknown mass of ice and the mixture is in thermal equilibrium. The water equivalent of the calorimeter is 250 \(g\) . At time \(t=0\), a heater is switched on which supplies heat at a constant rate to the calorimeter. The temperature of the mixture is measured at various times and the result has been plotted in the given figure. Neglect any heat loss from the mixture calorimeter system to the surrounding and calculate the initial mass of the ice.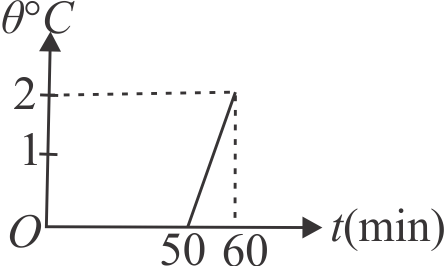
Given: Specific latent heat of fusion of ice is \(L_{f}=80\,\, {cal}\,\, {g}^{-1}\) Specific heat capacity of water \(=1\,\, {cal}\,\, {g}^{-1}{ }^{\circ} {C}^{-1}\)
366383 A bullet of mass \(10 \times {10^{ - 3}}\;kg\) moving with a speed of \(40\;m{s^{ - 1}}\) hits an ice block \(\left( {0^\circ C} \right)\) of \(990\;g\) kept at rest on a frictionless floor and gets embedded in it. If ice takes \(50 \%\) of \(K.E\) lost by the system, the amount of ice melted (in gram) approximately is: \((1\,cal = 4.2\;J)\) Latent heat of ice \( = 80\,cal/g)\)
366381 \(1\;kg\) of water at \(100^\circ C\) is converted into steam at \(100^\circ C\) by boiling at atmospheric pressure. The volume of water changes from \(1.00 \times {10^{ - 3}}\;{m^3}\) as a liquid to \(1.671\;{m^3}\) as steam. The change in internal energy of the system during the process will be (Given latent heat of vaporisation \( = 2257\;kJ/kg\), Atmospheric pressure \( = 1 \times {10^5}\;Pa\))
366382
In a calorimeter there is 5 \(kg\) of water at \(0^{\circ} {C}\) mixed to an unknown mass of ice and the mixture is in thermal equilibrium. The water equivalent of the calorimeter is 250 \(g\) . At time \(t=0\), a heater is switched on which supplies heat at a constant rate to the calorimeter. The temperature of the mixture is measured at various times and the result has been plotted in the given figure. Neglect any heat loss from the mixture calorimeter system to the surrounding and calculate the initial mass of the ice.
Given: Specific latent heat of fusion of ice is \(L_{f}=80\,\, {cal}\,\, {g}^{-1}\) Specific heat capacity of water \(=1\,\, {cal}\,\, {g}^{-1}{ }^{\circ} {C}^{-1}\)
366383 A bullet of mass \(10 \times {10^{ - 3}}\;kg\) moving with a speed of \(40\;m{s^{ - 1}}\) hits an ice block \(\left( {0^\circ C} \right)\) of \(990\;g\) kept at rest on a frictionless floor and gets embedded in it. If ice takes \(50 \%\) of \(K.E\) lost by the system, the amount of ice melted (in gram) approximately is: \((1\,cal = 4.2\;J)\) Latent heat of ice \( = 80\,cal/g)\)
366381 \(1\;kg\) of water at \(100^\circ C\) is converted into steam at \(100^\circ C\) by boiling at atmospheric pressure. The volume of water changes from \(1.00 \times {10^{ - 3}}\;{m^3}\) as a liquid to \(1.671\;{m^3}\) as steam. The change in internal energy of the system during the process will be (Given latent heat of vaporisation \( = 2257\;kJ/kg\), Atmospheric pressure \( = 1 \times {10^5}\;Pa\))
366382
In a calorimeter there is 5 \(kg\) of water at \(0^{\circ} {C}\) mixed to an unknown mass of ice and the mixture is in thermal equilibrium. The water equivalent of the calorimeter is 250 \(g\) . At time \(t=0\), a heater is switched on which supplies heat at a constant rate to the calorimeter. The temperature of the mixture is measured at various times and the result has been plotted in the given figure. Neglect any heat loss from the mixture calorimeter system to the surrounding and calculate the initial mass of the ice.
Given: Specific latent heat of fusion of ice is \(L_{f}=80\,\, {cal}\,\, {g}^{-1}\) Specific heat capacity of water \(=1\,\, {cal}\,\, {g}^{-1}{ }^{\circ} {C}^{-1}\)
366383 A bullet of mass \(10 \times {10^{ - 3}}\;kg\) moving with a speed of \(40\;m{s^{ - 1}}\) hits an ice block \(\left( {0^\circ C} \right)\) of \(990\;g\) kept at rest on a frictionless floor and gets embedded in it. If ice takes \(50 \%\) of \(K.E\) lost by the system, the amount of ice melted (in gram) approximately is: \((1\,cal = 4.2\;J)\) Latent heat of ice \( = 80\,cal/g)\)
366381 \(1\;kg\) of water at \(100^\circ C\) is converted into steam at \(100^\circ C\) by boiling at atmospheric pressure. The volume of water changes from \(1.00 \times {10^{ - 3}}\;{m^3}\) as a liquid to \(1.671\;{m^3}\) as steam. The change in internal energy of the system during the process will be (Given latent heat of vaporisation \( = 2257\;kJ/kg\), Atmospheric pressure \( = 1 \times {10^5}\;Pa\))
366382
In a calorimeter there is 5 \(kg\) of water at \(0^{\circ} {C}\) mixed to an unknown mass of ice and the mixture is in thermal equilibrium. The water equivalent of the calorimeter is 250 \(g\) . At time \(t=0\), a heater is switched on which supplies heat at a constant rate to the calorimeter. The temperature of the mixture is measured at various times and the result has been plotted in the given figure. Neglect any heat loss from the mixture calorimeter system to the surrounding and calculate the initial mass of the ice.
Given: Specific latent heat of fusion of ice is \(L_{f}=80\,\, {cal}\,\, {g}^{-1}\) Specific heat capacity of water \(=1\,\, {cal}\,\, {g}^{-1}{ }^{\circ} {C}^{-1}\)
366383 A bullet of mass \(10 \times {10^{ - 3}}\;kg\) moving with a speed of \(40\;m{s^{ - 1}}\) hits an ice block \(\left( {0^\circ C} \right)\) of \(990\;g\) kept at rest on a frictionless floor and gets embedded in it. If ice takes \(50 \%\) of \(K.E\) lost by the system, the amount of ice melted (in gram) approximately is: \((1\,cal = 4.2\;J)\) Latent heat of ice \( = 80\,cal/g)\)
