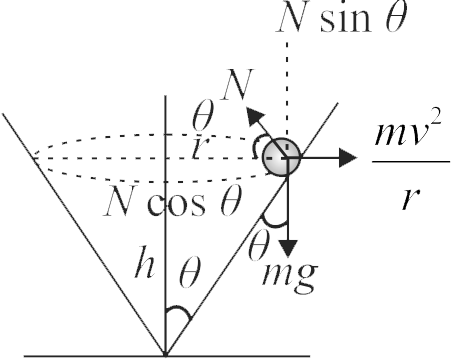
363201 A right circular cone is fixed with its axis vertical and vertex down. A bead is in contact with its smooth inside surface and describes circular motion in a horizontal plane at the height of \(10\,cm\) above the vertex. Find its velocity in \({{m} / {s}}\). (Take \({\left.g=10 {~m} / {s}^{2}\right)}\)

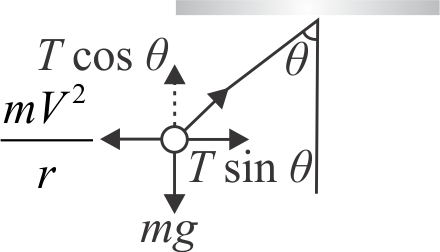
363202 A car is moving in a circular horizontal track of radius \(10.0\;m\) with a constant speed of \(10.0\;m{s^{ - 1}}.\) A plumb bob is suspended from the roof of the car by a light rigid rod of length \(10.0\;m.\) The angle made by the rod with the track is (take, \(g = 10\;m{s^{ - 2}}\) )

363203 A car is moving on a horizontal curved road with radius \(50\;m\). The approximate maximum speed of car will be, if friction between tyres and road is 0.34. [ take \({g = 10\;m{s^{ - 2}}}\)]

363204 A car is moving with a constant speed of \(20\;m{\rm{/}}s\) in a circular horizontal track of radius \(40\;m\). A bob is suspended from the roof of the car by a massless string. The angle made by the string with the vertical will be (Take \({g = 10\;m{\rm{/}}{s^2}}\))

363205 A small block of mass \(100\;g\) is tied to a spring of spring constant \(7.5\;N{\rm{/}}m\) and length \(20\;cm\). The other end of spring fixed at a particular point \(A\). If the block moves in a circular path on a smooth horizontal surface with constant angular velocity \(5\,rad{\rm{/}}s\) about point \(A\), then tension in the spring is

363201 A right circular cone is fixed with its axis vertical and vertex down. A bead is in contact with its smooth inside surface and describes circular motion in a horizontal plane at the height of \(10\,cm\) above the vertex. Find its velocity in \({{m} / {s}}\). (Take \({\left.g=10 {~m} / {s}^{2}\right)}\)

363202 A car is moving in a circular horizontal track of radius \(10.0\;m\) with a constant speed of \(10.0\;m{s^{ - 1}}.\) A plumb bob is suspended from the roof of the car by a light rigid rod of length \(10.0\;m.\) The angle made by the rod with the track is (take, \(g = 10\;m{s^{ - 2}}\) )

363203 A car is moving on a horizontal curved road with radius \(50\;m\). The approximate maximum speed of car will be, if friction between tyres and road is 0.34. [ take \({g = 10\;m{s^{ - 2}}}\)]

363204 A car is moving with a constant speed of \(20\;m{\rm{/}}s\) in a circular horizontal track of radius \(40\;m\). A bob is suspended from the roof of the car by a massless string. The angle made by the string with the vertical will be (Take \({g = 10\;m{\rm{/}}{s^2}}\))

363205 A small block of mass \(100\;g\) is tied to a spring of spring constant \(7.5\;N{\rm{/}}m\) and length \(20\;cm\). The other end of spring fixed at a particular point \(A\). If the block moves in a circular path on a smooth horizontal surface with constant angular velocity \(5\,rad{\rm{/}}s\) about point \(A\), then tension in the spring is

363201 A right circular cone is fixed with its axis vertical and vertex down. A bead is in contact with its smooth inside surface and describes circular motion in a horizontal plane at the height of \(10\,cm\) above the vertex. Find its velocity in \({{m} / {s}}\). (Take \({\left.g=10 {~m} / {s}^{2}\right)}\)

363202 A car is moving in a circular horizontal track of radius \(10.0\;m\) with a constant speed of \(10.0\;m{s^{ - 1}}.\) A plumb bob is suspended from the roof of the car by a light rigid rod of length \(10.0\;m.\) The angle made by the rod with the track is (take, \(g = 10\;m{s^{ - 2}}\) )

363203 A car is moving on a horizontal curved road with radius \(50\;m\). The approximate maximum speed of car will be, if friction between tyres and road is 0.34. [ take \({g = 10\;m{s^{ - 2}}}\)]

363204 A car is moving with a constant speed of \(20\;m{\rm{/}}s\) in a circular horizontal track of radius \(40\;m\). A bob is suspended from the roof of the car by a massless string. The angle made by the string with the vertical will be (Take \({g = 10\;m{\rm{/}}{s^2}}\))

363205 A small block of mass \(100\;g\) is tied to a spring of spring constant \(7.5\;N{\rm{/}}m\) and length \(20\;cm\). The other end of spring fixed at a particular point \(A\). If the block moves in a circular path on a smooth horizontal surface with constant angular velocity \(5\,rad{\rm{/}}s\) about point \(A\), then tension in the spring is

363201 A right circular cone is fixed with its axis vertical and vertex down. A bead is in contact with its smooth inside surface and describes circular motion in a horizontal plane at the height of \(10\,cm\) above the vertex. Find its velocity in \({{m} / {s}}\). (Take \({\left.g=10 {~m} / {s}^{2}\right)}\)

363202 A car is moving in a circular horizontal track of radius \(10.0\;m\) with a constant speed of \(10.0\;m{s^{ - 1}}.\) A plumb bob is suspended from the roof of the car by a light rigid rod of length \(10.0\;m.\) The angle made by the rod with the track is (take, \(g = 10\;m{s^{ - 2}}\) )

363203 A car is moving on a horizontal curved road with radius \(50\;m\). The approximate maximum speed of car will be, if friction between tyres and road is 0.34. [ take \({g = 10\;m{s^{ - 2}}}\)]

363204 A car is moving with a constant speed of \(20\;m{\rm{/}}s\) in a circular horizontal track of radius \(40\;m\). A bob is suspended from the roof of the car by a massless string. The angle made by the string with the vertical will be (Take \({g = 10\;m{\rm{/}}{s^2}}\))

363205 A small block of mass \(100\;g\) is tied to a spring of spring constant \(7.5\;N{\rm{/}}m\) and length \(20\;cm\). The other end of spring fixed at a particular point \(A\). If the block moves in a circular path on a smooth horizontal surface with constant angular velocity \(5\,rad{\rm{/}}s\) about point \(A\), then tension in the spring is

363201 A right circular cone is fixed with its axis vertical and vertex down. A bead is in contact with its smooth inside surface and describes circular motion in a horizontal plane at the height of \(10\,cm\) above the vertex. Find its velocity in \({{m} / {s}}\). (Take \({\left.g=10 {~m} / {s}^{2}\right)}\)

363202 A car is moving in a circular horizontal track of radius \(10.0\;m\) with a constant speed of \(10.0\;m{s^{ - 1}}.\) A plumb bob is suspended from the roof of the car by a light rigid rod of length \(10.0\;m.\) The angle made by the rod with the track is (take, \(g = 10\;m{s^{ - 2}}\) )

363203 A car is moving on a horizontal curved road with radius \(50\;m\). The approximate maximum speed of car will be, if friction between tyres and road is 0.34. [ take \({g = 10\;m{s^{ - 2}}}\)]

363204 A car is moving with a constant speed of \(20\;m{\rm{/}}s\) in a circular horizontal track of radius \(40\;m\). A bob is suspended from the roof of the car by a massless string. The angle made by the string with the vertical will be (Take \({g = 10\;m{\rm{/}}{s^2}}\))

363205 A small block of mass \(100\;g\) is tied to a spring of spring constant \(7.5\;N{\rm{/}}m\) and length \(20\;cm\). The other end of spring fixed at a particular point \(A\). If the block moves in a circular path on a smooth horizontal surface with constant angular velocity \(5\,rad{\rm{/}}s\) about point \(A\), then tension in the spring is