361839
A point \(P\) moves in counter-clockwise direction on a circular path as shown in the figure. The movement of \('P'\) is such that it sweeps out a length \(s=t^{3}+5\), where \(s\) is in metres and \(t\) is in seconds. The radius of the path is \(27\;m.\) The acceleration of ' \(P\) ' when \(t = 3\;s\) is
(Take \(\sqrt{13}=3.6\) )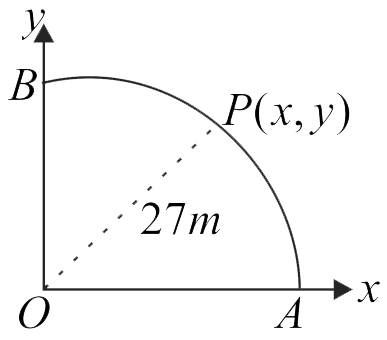
361839
A point \(P\) moves in counter-clockwise direction on a circular path as shown in the figure. The movement of \('P'\) is such that it sweeps out a length \(s=t^{3}+5\), where \(s\) is in metres and \(t\) is in seconds. The radius of the path is \(27\;m.\) The acceleration of ' \(P\) ' when \(t = 3\;s\) is
(Take \(\sqrt{13}=3.6\) )
361839
A point \(P\) moves in counter-clockwise direction on a circular path as shown in the figure. The movement of \('P'\) is such that it sweeps out a length \(s=t^{3}+5\), where \(s\) is in metres and \(t\) is in seconds. The radius of the path is \(27\;m.\) The acceleration of ' \(P\) ' when \(t = 3\;s\) is
(Take \(\sqrt{13}=3.6\) )
361839
A point \(P\) moves in counter-clockwise direction on a circular path as shown in the figure. The movement of \('P'\) is such that it sweeps out a length \(s=t^{3}+5\), where \(s\) is in metres and \(t\) is in seconds. The radius of the path is \(27\;m.\) The acceleration of ' \(P\) ' when \(t = 3\;s\) is
(Take \(\sqrt{13}=3.6\) )
361839
A point \(P\) moves in counter-clockwise direction on a circular path as shown in the figure. The movement of \('P'\) is such that it sweeps out a length \(s=t^{3}+5\), where \(s\) is in metres and \(t\) is in seconds. The radius of the path is \(27\;m.\) The acceleration of ' \(P\) ' when \(t = 3\;s\) is
(Take \(\sqrt{13}=3.6\) )