355066 \(S_{1}\) and \(S_{2}\) are two coherent sources of sound of frequency \(110\;Hz\) each. they have no initial phase difference. The intensity at a point \(P\) due to \(S_{1}\) is \(I_{0}\) and due to \(S_{2}\) is \(4 I_{0}\). If the velocity of sound is \(330\;m/s\) then the resultant intensity at \(P\) is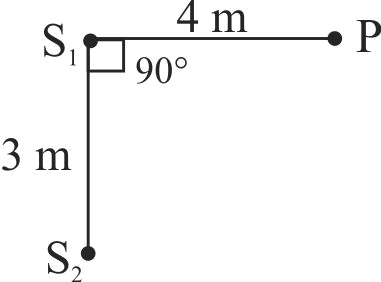

355067 two waves are propogating to the point p along a straight line produces by two sources \(A\) and \(B\) of sample harmonic and of equal frequency. The amplitude of every wave at \(P\) is '\(a\)' and phase of \(A\) is ahead \(\frac{\pi }{3}\) than that of \(B\) and the distance \(AP\) is greater than \(BP\) by \(50\,cm\). Then the resultant amplitude at the point \(P\) will be, If the wavelength is 1 meter

355068 Assertion :
The phenomenon of interference is based on conservation of energy.
Reason :
For interference of two waves the amplitude must be nearly equal.

355069 Statement A :
The interference of two identical waves moving in same direction produces standing waves.
Statement B :
Various particles of standing waves do not remain in constant phase.

355066 \(S_{1}\) and \(S_{2}\) are two coherent sources of sound of frequency \(110\;Hz\) each. they have no initial phase difference. The intensity at a point \(P\) due to \(S_{1}\) is \(I_{0}\) and due to \(S_{2}\) is \(4 I_{0}\). If the velocity of sound is \(330\;m/s\) then the resultant intensity at \(P\) is

355067 two waves are propogating to the point p along a straight line produces by two sources \(A\) and \(B\) of sample harmonic and of equal frequency. The amplitude of every wave at \(P\) is '\(a\)' and phase of \(A\) is ahead \(\frac{\pi }{3}\) than that of \(B\) and the distance \(AP\) is greater than \(BP\) by \(50\,cm\). Then the resultant amplitude at the point \(P\) will be, If the wavelength is 1 meter

355068 Assertion :
The phenomenon of interference is based on conservation of energy.
Reason :
For interference of two waves the amplitude must be nearly equal.

355069 Statement A :
The interference of two identical waves moving in same direction produces standing waves.
Statement B :
Various particles of standing waves do not remain in constant phase.

355066 \(S_{1}\) and \(S_{2}\) are two coherent sources of sound of frequency \(110\;Hz\) each. they have no initial phase difference. The intensity at a point \(P\) due to \(S_{1}\) is \(I_{0}\) and due to \(S_{2}\) is \(4 I_{0}\). If the velocity of sound is \(330\;m/s\) then the resultant intensity at \(P\) is

355067 two waves are propogating to the point p along a straight line produces by two sources \(A\) and \(B\) of sample harmonic and of equal frequency. The amplitude of every wave at \(P\) is '\(a\)' and phase of \(A\) is ahead \(\frac{\pi }{3}\) than that of \(B\) and the distance \(AP\) is greater than \(BP\) by \(50\,cm\). Then the resultant amplitude at the point \(P\) will be, If the wavelength is 1 meter

355068 Assertion :
The phenomenon of interference is based on conservation of energy.
Reason :
For interference of two waves the amplitude must be nearly equal.

355069 Statement A :
The interference of two identical waves moving in same direction produces standing waves.
Statement B :
Various particles of standing waves do not remain in constant phase.

355066 \(S_{1}\) and \(S_{2}\) are two coherent sources of sound of frequency \(110\;Hz\) each. they have no initial phase difference. The intensity at a point \(P\) due to \(S_{1}\) is \(I_{0}\) and due to \(S_{2}\) is \(4 I_{0}\). If the velocity of sound is \(330\;m/s\) then the resultant intensity at \(P\) is

355067 two waves are propogating to the point p along a straight line produces by two sources \(A\) and \(B\) of sample harmonic and of equal frequency. The amplitude of every wave at \(P\) is '\(a\)' and phase of \(A\) is ahead \(\frac{\pi }{3}\) than that of \(B\) and the distance \(AP\) is greater than \(BP\) by \(50\,cm\). Then the resultant amplitude at the point \(P\) will be, If the wavelength is 1 meter

355068 Assertion :
The phenomenon of interference is based on conservation of energy.
Reason :
For interference of two waves the amplitude must be nearly equal.

355069 Statement A :
The interference of two identical waves moving in same direction produces standing waves.
Statement B :
Various particles of standing waves do not remain in constant phase.