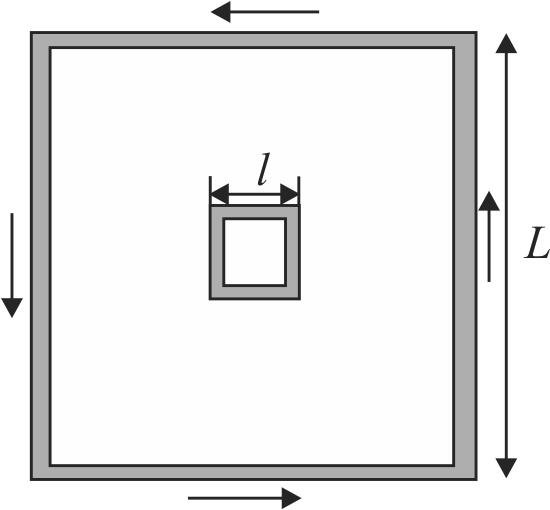
358529 A small square loop of wire of side \({l}\) is placed inside a large square loop of wire of side \({L(>>l)}\). The loops are coplanar and their centres coincide. If the mutual inductance of the system is found to be \({N \sqrt{2} \dfrac{\mu_{0} l^{2}}{\pi L}}\), find the value of \({N}\).

358530 A pair of adjacent coils has a mutual inductance of \(2.5\,H\). If the current in one coil changes from 0 to \(40\;A\) in \(0.8\,s\), then the change in flux linked with the other coil is

358531 Two coils have a mutual inductance \(0.005\,H.\) the current changes in the first coil according to the equation \(i = {i_m}\sin \omega t\) where \({i_m} = 10\;A\) and \(\omega = 100\,\pi rad{s^{ - 1}}.\) The maximum value of the emf induced (V) in the second coil is]

358532 A circuit contains two inductors of selfinductance \(L_{1}\) and \(L_{2}\) in series. If \(M\) is the mutual inductance then the effective inductance of the circuit shown will be

358529 A small square loop of wire of side \({l}\) is placed inside a large square loop of wire of side \({L(>>l)}\). The loops are coplanar and their centres coincide. If the mutual inductance of the system is found to be \({N \sqrt{2} \dfrac{\mu_{0} l^{2}}{\pi L}}\), find the value of \({N}\).

358530 A pair of adjacent coils has a mutual inductance of \(2.5\,H\). If the current in one coil changes from 0 to \(40\;A\) in \(0.8\,s\), then the change in flux linked with the other coil is

358531 Two coils have a mutual inductance \(0.005\,H.\) the current changes in the first coil according to the equation \(i = {i_m}\sin \omega t\) where \({i_m} = 10\;A\) and \(\omega = 100\,\pi rad{s^{ - 1}}.\) The maximum value of the emf induced (V) in the second coil is]

358532 A circuit contains two inductors of selfinductance \(L_{1}\) and \(L_{2}\) in series. If \(M\) is the mutual inductance then the effective inductance of the circuit shown will be

358529 A small square loop of wire of side \({l}\) is placed inside a large square loop of wire of side \({L(>>l)}\). The loops are coplanar and their centres coincide. If the mutual inductance of the system is found to be \({N \sqrt{2} \dfrac{\mu_{0} l^{2}}{\pi L}}\), find the value of \({N}\).

358530 A pair of adjacent coils has a mutual inductance of \(2.5\,H\). If the current in one coil changes from 0 to \(40\;A\) in \(0.8\,s\), then the change in flux linked with the other coil is

358531 Two coils have a mutual inductance \(0.005\,H.\) the current changes in the first coil according to the equation \(i = {i_m}\sin \omega t\) where \({i_m} = 10\;A\) and \(\omega = 100\,\pi rad{s^{ - 1}}.\) The maximum value of the emf induced (V) in the second coil is]

358532 A circuit contains two inductors of selfinductance \(L_{1}\) and \(L_{2}\) in series. If \(M\) is the mutual inductance then the effective inductance of the circuit shown will be

358529 A small square loop of wire of side \({l}\) is placed inside a large square loop of wire of side \({L(>>l)}\). The loops are coplanar and their centres coincide. If the mutual inductance of the system is found to be \({N \sqrt{2} \dfrac{\mu_{0} l^{2}}{\pi L}}\), find the value of \({N}\).

358530 A pair of adjacent coils has a mutual inductance of \(2.5\,H\). If the current in one coil changes from 0 to \(40\;A\) in \(0.8\,s\), then the change in flux linked with the other coil is

358531 Two coils have a mutual inductance \(0.005\,H.\) the current changes in the first coil according to the equation \(i = {i_m}\sin \omega t\) where \({i_m} = 10\;A\) and \(\omega = 100\,\pi rad{s^{ - 1}}.\) The maximum value of the emf induced (V) in the second coil is]

358532 A circuit contains two inductors of selfinductance \(L_{1}\) and \(L_{2}\) in series. If \(M\) is the mutual inductance then the effective inductance of the circuit shown will be