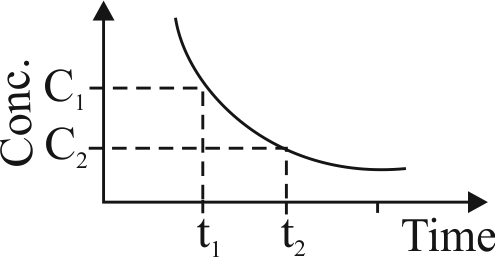
320579 In an experiment to study the reaction \({\text{A + 2B}} \to {\text{C + 2D}}\), the initial rate \(\frac{{{\text{ - d[A]}}}}{{{\text{dt}}}}\) at \(\mathrm{t}=\) 0 was found to be \({\rm{2}}{\rm{.6 \times 1}}{{\rm{0}}^{{\rm{ - 2}}}}{\rm{M}}{{\rm{s}}^{{\rm{ - 1}}}}\). What is the value of \(\frac{{{\text{ - d[B]}}}}{{{\text{dt}}}}{\mkern 1mu} {\mkern 1mu} {\text{at t = 0}}\,\,{\text{in M}}{{\text{s}}^{{\text{ - 1}}}}\) ?
320580 For the reaction \({{\rm{N}}_{\rm{2}}}{\rm{(g) + 3}}{{\rm{H}}_{\rm{2}}}{\rm{(g)}} \to {\rm{2N}}{{\rm{H}}_{\rm{3}}}{\rm{(g)}}\) under certain conditions of temperature and partial pressure of the reactants, the rate of formation of \({\text{N}}{{\text{H}}_{\text{3}}}{\mkern 1mu} {\mkern 1mu} {\text{is}}{\mkern 1mu} \,\,{\text{0}}{\text{.001}}\;{\text{mole}}{\mkern 1mu} {\mkern 1mu} {\text{h}}{{\text{r}}^{{\text{ - 1}}}}\). The rate of conversion of \(\mathrm{H}_{2}\) under the same conditions is
320579 In an experiment to study the reaction \({\text{A + 2B}} \to {\text{C + 2D}}\), the initial rate \(\frac{{{\text{ - d[A]}}}}{{{\text{dt}}}}\) at \(\mathrm{t}=\) 0 was found to be \({\rm{2}}{\rm{.6 \times 1}}{{\rm{0}}^{{\rm{ - 2}}}}{\rm{M}}{{\rm{s}}^{{\rm{ - 1}}}}\). What is the value of \(\frac{{{\text{ - d[B]}}}}{{{\text{dt}}}}{\mkern 1mu} {\mkern 1mu} {\text{at t = 0}}\,\,{\text{in M}}{{\text{s}}^{{\text{ - 1}}}}\) ?
320580 For the reaction \({{\rm{N}}_{\rm{2}}}{\rm{(g) + 3}}{{\rm{H}}_{\rm{2}}}{\rm{(g)}} \to {\rm{2N}}{{\rm{H}}_{\rm{3}}}{\rm{(g)}}\) under certain conditions of temperature and partial pressure of the reactants, the rate of formation of \({\text{N}}{{\text{H}}_{\text{3}}}{\mkern 1mu} {\mkern 1mu} {\text{is}}{\mkern 1mu} \,\,{\text{0}}{\text{.001}}\;{\text{mole}}{\mkern 1mu} {\mkern 1mu} {\text{h}}{{\text{r}}^{{\text{ - 1}}}}\). The rate of conversion of \(\mathrm{H}_{2}\) under the same conditions is
320579 In an experiment to study the reaction \({\text{A + 2B}} \to {\text{C + 2D}}\), the initial rate \(\frac{{{\text{ - d[A]}}}}{{{\text{dt}}}}\) at \(\mathrm{t}=\) 0 was found to be \({\rm{2}}{\rm{.6 \times 1}}{{\rm{0}}^{{\rm{ - 2}}}}{\rm{M}}{{\rm{s}}^{{\rm{ - 1}}}}\). What is the value of \(\frac{{{\text{ - d[B]}}}}{{{\text{dt}}}}{\mkern 1mu} {\mkern 1mu} {\text{at t = 0}}\,\,{\text{in M}}{{\text{s}}^{{\text{ - 1}}}}\) ?
320580 For the reaction \({{\rm{N}}_{\rm{2}}}{\rm{(g) + 3}}{{\rm{H}}_{\rm{2}}}{\rm{(g)}} \to {\rm{2N}}{{\rm{H}}_{\rm{3}}}{\rm{(g)}}\) under certain conditions of temperature and partial pressure of the reactants, the rate of formation of \({\text{N}}{{\text{H}}_{\text{3}}}{\mkern 1mu} {\mkern 1mu} {\text{is}}{\mkern 1mu} \,\,{\text{0}}{\text{.001}}\;{\text{mole}}{\mkern 1mu} {\mkern 1mu} {\text{h}}{{\text{r}}^{{\text{ - 1}}}}\). The rate of conversion of \(\mathrm{H}_{2}\) under the same conditions is
320579 In an experiment to study the reaction \({\text{A + 2B}} \to {\text{C + 2D}}\), the initial rate \(\frac{{{\text{ - d[A]}}}}{{{\text{dt}}}}\) at \(\mathrm{t}=\) 0 was found to be \({\rm{2}}{\rm{.6 \times 1}}{{\rm{0}}^{{\rm{ - 2}}}}{\rm{M}}{{\rm{s}}^{{\rm{ - 1}}}}\). What is the value of \(\frac{{{\text{ - d[B]}}}}{{{\text{dt}}}}{\mkern 1mu} {\mkern 1mu} {\text{at t = 0}}\,\,{\text{in M}}{{\text{s}}^{{\text{ - 1}}}}\) ?
320580 For the reaction \({{\rm{N}}_{\rm{2}}}{\rm{(g) + 3}}{{\rm{H}}_{\rm{2}}}{\rm{(g)}} \to {\rm{2N}}{{\rm{H}}_{\rm{3}}}{\rm{(g)}}\) under certain conditions of temperature and partial pressure of the reactants, the rate of formation of \({\text{N}}{{\text{H}}_{\text{3}}}{\mkern 1mu} {\mkern 1mu} {\text{is}}{\mkern 1mu} \,\,{\text{0}}{\text{.001}}\;{\text{mole}}{\mkern 1mu} {\mkern 1mu} {\text{h}}{{\text{r}}^{{\text{ - 1}}}}\). The rate of conversion of \(\mathrm{H}_{2}\) under the same conditions is