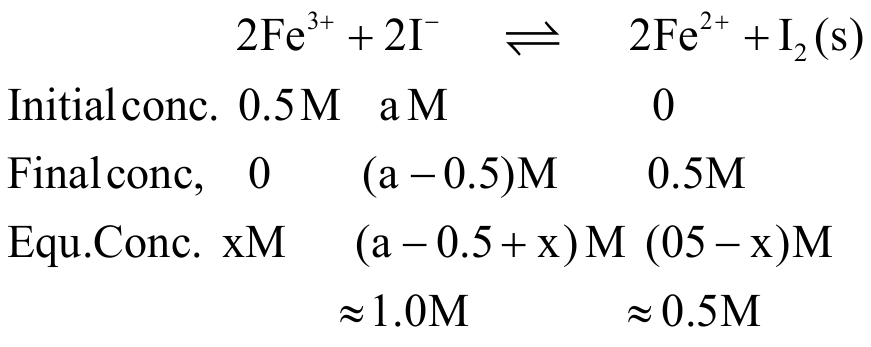329956 Two concentration cells of \({\rm{Ag}}\) with \({\rm{Ag}}\) electrode are dipped in solution of \(\mathrm{AgNO}_{3}\). In first cell, concentration of one electrode is \(1 \mathrm{M}\) and other electrode is 0.1 and emf is \(0.06 \mathrm{~V}\). In second cell, concentration of one electrode is \(1 \mathrm{M}\) and other electrode is \(0.01 \mathrm{M}\). Calculate the emf of second cell.
329957
The Nernst equation is written as
\({{\rm{E}}_{{\rm{cell}}}}{\rm{ = E}}_{{\rm{cell}}}^{\rm{o}}{\rm{ - }}\frac{{{\rm{R}}\,{\rm{T}}}}{{{\rm{nF}}}}{\rm{ln}}\frac{{\left[ {{\rm{N}}{{\rm{i}}^{{\rm{2 + }}}}} \right]}}{{{{\left[ {{\rm{A}}{{\rm{g}}^{\rm{ + }}}} \right]}^{\rm{2}}}}}\)
Select the correct cell representation for the above equation.
329958 The concentration of \(\left[\mathrm{Fe}^{3+}\right]\) at equilibrium when potassium iodide is added to a solution of \(\mathrm{Fe}^{3+}\) initially \(0.50\,{\rm{M}}\) until \([{{\rm{I}}^ - }] = 1.0{\mkern 1mu} \,{\rm{M}}\) is ____\(({\rm{Given}}\,{\rm{E}}_{{\rm{F}}{{\rm{e}}^{3 + }}\mid {\rm{F}}{{\rm{e}}^{2 + }}}^0 = 0.770\;{\rm{V}},{\rm{E}}{^\circ _{{{\rm{I}}_2}{\rm{/}}{{\rm{I}}^ - }}} = 0.535\;{\rm{V}})\)
329959
Calculate E.M.F. of following cell at \({\rm{298K}}\)
\(\left. {\,{\rm{Zn}}\left( {\rm{s}} \right)} \right \vert \left. {{\rm{ZnSO4}}({\rm{0}}.{\rm{01M}})} \right \vert \left. {\left \vert {{\rm{CuSO4}}({\rm{1}}.{\rm{0M}})} \right.} \right \vert {\rm{Cu}}({\rm{s}})\,\,\)
\({\rm{if}}\,\,{{\rm{E}}^{\rm{o}}}{\rm{cell}}\,{\rm{ = }}\,{\rm{2}}.{\rm{0V}}\)
329956 Two concentration cells of \({\rm{Ag}}\) with \({\rm{Ag}}\) electrode are dipped in solution of \(\mathrm{AgNO}_{3}\). In first cell, concentration of one electrode is \(1 \mathrm{M}\) and other electrode is 0.1 and emf is \(0.06 \mathrm{~V}\). In second cell, concentration of one electrode is \(1 \mathrm{M}\) and other electrode is \(0.01 \mathrm{M}\). Calculate the emf of second cell.
329957
The Nernst equation is written as
\({{\rm{E}}_{{\rm{cell}}}}{\rm{ = E}}_{{\rm{cell}}}^{\rm{o}}{\rm{ - }}\frac{{{\rm{R}}\,{\rm{T}}}}{{{\rm{nF}}}}{\rm{ln}}\frac{{\left[ {{\rm{N}}{{\rm{i}}^{{\rm{2 + }}}}} \right]}}{{{{\left[ {{\rm{A}}{{\rm{g}}^{\rm{ + }}}} \right]}^{\rm{2}}}}}\)
Select the correct cell representation for the above equation.
329958 The concentration of \(\left[\mathrm{Fe}^{3+}\right]\) at equilibrium when potassium iodide is added to a solution of \(\mathrm{Fe}^{3+}\) initially \(0.50\,{\rm{M}}\) until \([{{\rm{I}}^ - }] = 1.0{\mkern 1mu} \,{\rm{M}}\) is ____\(({\rm{Given}}\,{\rm{E}}_{{\rm{F}}{{\rm{e}}^{3 + }}\mid {\rm{F}}{{\rm{e}}^{2 + }}}^0 = 0.770\;{\rm{V}},{\rm{E}}{^\circ _{{{\rm{I}}_2}{\rm{/}}{{\rm{I}}^ - }}} = 0.535\;{\rm{V}})\)
329959
Calculate E.M.F. of following cell at \({\rm{298K}}\)
\(\left. {\,{\rm{Zn}}\left( {\rm{s}} \right)} \right \vert \left. {{\rm{ZnSO4}}({\rm{0}}.{\rm{01M}})} \right \vert \left. {\left \vert {{\rm{CuSO4}}({\rm{1}}.{\rm{0M}})} \right.} \right \vert {\rm{Cu}}({\rm{s}})\,\,\)
\({\rm{if}}\,\,{{\rm{E}}^{\rm{o}}}{\rm{cell}}\,{\rm{ = }}\,{\rm{2}}.{\rm{0V}}\)
329956 Two concentration cells of \({\rm{Ag}}\) with \({\rm{Ag}}\) electrode are dipped in solution of \(\mathrm{AgNO}_{3}\). In first cell, concentration of one electrode is \(1 \mathrm{M}\) and other electrode is 0.1 and emf is \(0.06 \mathrm{~V}\). In second cell, concentration of one electrode is \(1 \mathrm{M}\) and other electrode is \(0.01 \mathrm{M}\). Calculate the emf of second cell.
329957
The Nernst equation is written as
\({{\rm{E}}_{{\rm{cell}}}}{\rm{ = E}}_{{\rm{cell}}}^{\rm{o}}{\rm{ - }}\frac{{{\rm{R}}\,{\rm{T}}}}{{{\rm{nF}}}}{\rm{ln}}\frac{{\left[ {{\rm{N}}{{\rm{i}}^{{\rm{2 + }}}}} \right]}}{{{{\left[ {{\rm{A}}{{\rm{g}}^{\rm{ + }}}} \right]}^{\rm{2}}}}}\)
Select the correct cell representation for the above equation.
329958 The concentration of \(\left[\mathrm{Fe}^{3+}\right]\) at equilibrium when potassium iodide is added to a solution of \(\mathrm{Fe}^{3+}\) initially \(0.50\,{\rm{M}}\) until \([{{\rm{I}}^ - }] = 1.0{\mkern 1mu} \,{\rm{M}}\) is ____\(({\rm{Given}}\,{\rm{E}}_{{\rm{F}}{{\rm{e}}^{3 + }}\mid {\rm{F}}{{\rm{e}}^{2 + }}}^0 = 0.770\;{\rm{V}},{\rm{E}}{^\circ _{{{\rm{I}}_2}{\rm{/}}{{\rm{I}}^ - }}} = 0.535\;{\rm{V}})\)
329959
Calculate E.M.F. of following cell at \({\rm{298K}}\)
\(\left. {\,{\rm{Zn}}\left( {\rm{s}} \right)} \right \vert \left. {{\rm{ZnSO4}}({\rm{0}}.{\rm{01M}})} \right \vert \left. {\left \vert {{\rm{CuSO4}}({\rm{1}}.{\rm{0M}})} \right.} \right \vert {\rm{Cu}}({\rm{s}})\,\,\)
\({\rm{if}}\,\,{{\rm{E}}^{\rm{o}}}{\rm{cell}}\,{\rm{ = }}\,{\rm{2}}.{\rm{0V}}\)
329956 Two concentration cells of \({\rm{Ag}}\) with \({\rm{Ag}}\) electrode are dipped in solution of \(\mathrm{AgNO}_{3}\). In first cell, concentration of one electrode is \(1 \mathrm{M}\) and other electrode is 0.1 and emf is \(0.06 \mathrm{~V}\). In second cell, concentration of one electrode is \(1 \mathrm{M}\) and other electrode is \(0.01 \mathrm{M}\). Calculate the emf of second cell.
329957
The Nernst equation is written as
\({{\rm{E}}_{{\rm{cell}}}}{\rm{ = E}}_{{\rm{cell}}}^{\rm{o}}{\rm{ - }}\frac{{{\rm{R}}\,{\rm{T}}}}{{{\rm{nF}}}}{\rm{ln}}\frac{{\left[ {{\rm{N}}{{\rm{i}}^{{\rm{2 + }}}}} \right]}}{{{{\left[ {{\rm{A}}{{\rm{g}}^{\rm{ + }}}} \right]}^{\rm{2}}}}}\)
Select the correct cell representation for the above equation.
329958 The concentration of \(\left[\mathrm{Fe}^{3+}\right]\) at equilibrium when potassium iodide is added to a solution of \(\mathrm{Fe}^{3+}\) initially \(0.50\,{\rm{M}}\) until \([{{\rm{I}}^ - }] = 1.0{\mkern 1mu} \,{\rm{M}}\) is ____\(({\rm{Given}}\,{\rm{E}}_{{\rm{F}}{{\rm{e}}^{3 + }}\mid {\rm{F}}{{\rm{e}}^{2 + }}}^0 = 0.770\;{\rm{V}},{\rm{E}}{^\circ _{{{\rm{I}}_2}{\rm{/}}{{\rm{I}}^ - }}} = 0.535\;{\rm{V}})\)
329959
Calculate E.M.F. of following cell at \({\rm{298K}}\)
\(\left. {\,{\rm{Zn}}\left( {\rm{s}} \right)} \right \vert \left. {{\rm{ZnSO4}}({\rm{0}}.{\rm{01M}})} \right \vert \left. {\left \vert {{\rm{CuSO4}}({\rm{1}}.{\rm{0M}})} \right.} \right \vert {\rm{Cu}}({\rm{s}})\,\,\)
\({\rm{if}}\,\,{{\rm{E}}^{\rm{o}}}{\rm{cell}}\,{\rm{ = }}\,{\rm{2}}.{\rm{0V}}\)
329956 Two concentration cells of \({\rm{Ag}}\) with \({\rm{Ag}}\) electrode are dipped in solution of \(\mathrm{AgNO}_{3}\). In first cell, concentration of one electrode is \(1 \mathrm{M}\) and other electrode is 0.1 and emf is \(0.06 \mathrm{~V}\). In second cell, concentration of one electrode is \(1 \mathrm{M}\) and other electrode is \(0.01 \mathrm{M}\). Calculate the emf of second cell.
329957
The Nernst equation is written as
\({{\rm{E}}_{{\rm{cell}}}}{\rm{ = E}}_{{\rm{cell}}}^{\rm{o}}{\rm{ - }}\frac{{{\rm{R}}\,{\rm{T}}}}{{{\rm{nF}}}}{\rm{ln}}\frac{{\left[ {{\rm{N}}{{\rm{i}}^{{\rm{2 + }}}}} \right]}}{{{{\left[ {{\rm{A}}{{\rm{g}}^{\rm{ + }}}} \right]}^{\rm{2}}}}}\)
Select the correct cell representation for the above equation.
329958 The concentration of \(\left[\mathrm{Fe}^{3+}\right]\) at equilibrium when potassium iodide is added to a solution of \(\mathrm{Fe}^{3+}\) initially \(0.50\,{\rm{M}}\) until \([{{\rm{I}}^ - }] = 1.0{\mkern 1mu} \,{\rm{M}}\) is ____\(({\rm{Given}}\,{\rm{E}}_{{\rm{F}}{{\rm{e}}^{3 + }}\mid {\rm{F}}{{\rm{e}}^{2 + }}}^0 = 0.770\;{\rm{V}},{\rm{E}}{^\circ _{{{\rm{I}}_2}{\rm{/}}{{\rm{I}}^ - }}} = 0.535\;{\rm{V}})\)
329959
Calculate E.M.F. of following cell at \({\rm{298K}}\)
\(\left. {\,{\rm{Zn}}\left( {\rm{s}} \right)} \right \vert \left. {{\rm{ZnSO4}}({\rm{0}}.{\rm{01M}})} \right \vert \left. {\left \vert {{\rm{CuSO4}}({\rm{1}}.{\rm{0M}})} \right.} \right \vert {\rm{Cu}}({\rm{s}})\,\,\)
\({\rm{if}}\,\,{{\rm{E}}^{\rm{o}}}{\rm{cell}}\,{\rm{ = }}\,{\rm{2}}.{\rm{0V}}\)