270274 A man of mass\(65 \mathrm{~kg}\). is standing stationary with respect to a conveyor belt which is accel- erating with \(1 \mathrm{~m} / \mathrm{s}^{2}\). If \(\mu_{\mathrm{s}}\) is \(\mathbf{0 . 2}\), the net force on the man and the maximum acceleration of the belt so that the man is stationary relative to the belt are \(\left(g=10 \mathrm{~m} / \mathrm{s}^{2}\right)\)

270275 A man of mass\(60 \mathrm{~kg}\) sitting on ice pushes a block of mass of \(12 \mathrm{~kg}\) on ice horizontally with a speed of \(5 \mathrm{~ms}^{-1}\). The coefficient of friction between the man and ice and between block and ice is 0.2 . If \(g=10 \mathrm{~ms}^{-2}\), the distance between man and the block, when they come to rest is

270276 A vehicle of mass\(M\) is moving on a rough horizontal road with a momentum \(P\). If the coefficient of friction between the tyres and the road is \(\mu\), then the stopping distance is (EAM-2012)

270277 The rear side of a truck is open and a box of\(40 \mathrm{~kg}\) mass is placed \(5 \mathrm{~m}\) away from the open end as shown in figure. The coefficient of friction between the box the surface below it is 0.15. On a straight road, the truck starts from rest and accelerates with \(2 \mathrm{~ms}^{-2}\). At what distance from the starting point does the box fall from the truck? (Ignore the size of the box.)

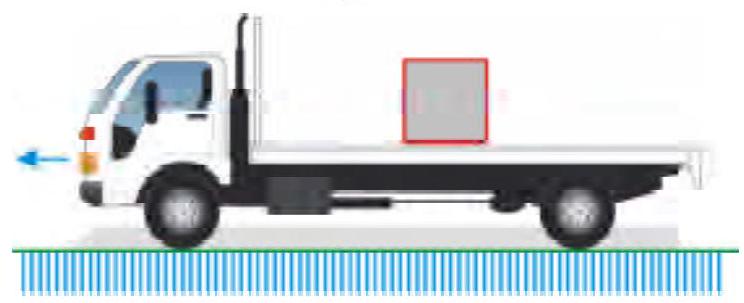
270274 A man of mass\(65 \mathrm{~kg}\). is standing stationary with respect to a conveyor belt which is accel- erating with \(1 \mathrm{~m} / \mathrm{s}^{2}\). If \(\mu_{\mathrm{s}}\) is \(\mathbf{0 . 2}\), the net force on the man and the maximum acceleration of the belt so that the man is stationary relative to the belt are \(\left(g=10 \mathrm{~m} / \mathrm{s}^{2}\right)\)

270275 A man of mass\(60 \mathrm{~kg}\) sitting on ice pushes a block of mass of \(12 \mathrm{~kg}\) on ice horizontally with a speed of \(5 \mathrm{~ms}^{-1}\). The coefficient of friction between the man and ice and between block and ice is 0.2 . If \(g=10 \mathrm{~ms}^{-2}\), the distance between man and the block, when they come to rest is

270276 A vehicle of mass\(M\) is moving on a rough horizontal road with a momentum \(P\). If the coefficient of friction between the tyres and the road is \(\mu\), then the stopping distance is (EAM-2012)

270277 The rear side of a truck is open and a box of\(40 \mathrm{~kg}\) mass is placed \(5 \mathrm{~m}\) away from the open end as shown in figure. The coefficient of friction between the box the surface below it is 0.15. On a straight road, the truck starts from rest and accelerates with \(2 \mathrm{~ms}^{-2}\). At what distance from the starting point does the box fall from the truck? (Ignore the size of the box.)

270274 A man of mass\(65 \mathrm{~kg}\). is standing stationary with respect to a conveyor belt which is accel- erating with \(1 \mathrm{~m} / \mathrm{s}^{2}\). If \(\mu_{\mathrm{s}}\) is \(\mathbf{0 . 2}\), the net force on the man and the maximum acceleration of the belt so that the man is stationary relative to the belt are \(\left(g=10 \mathrm{~m} / \mathrm{s}^{2}\right)\)

270275 A man of mass\(60 \mathrm{~kg}\) sitting on ice pushes a block of mass of \(12 \mathrm{~kg}\) on ice horizontally with a speed of \(5 \mathrm{~ms}^{-1}\). The coefficient of friction between the man and ice and between block and ice is 0.2 . If \(g=10 \mathrm{~ms}^{-2}\), the distance between man and the block, when they come to rest is

270276 A vehicle of mass\(M\) is moving on a rough horizontal road with a momentum \(P\). If the coefficient of friction between the tyres and the road is \(\mu\), then the stopping distance is (EAM-2012)

270277 The rear side of a truck is open and a box of\(40 \mathrm{~kg}\) mass is placed \(5 \mathrm{~m}\) away from the open end as shown in figure. The coefficient of friction between the box the surface below it is 0.15. On a straight road, the truck starts from rest and accelerates with \(2 \mathrm{~ms}^{-2}\). At what distance from the starting point does the box fall from the truck? (Ignore the size of the box.)

270274 A man of mass\(65 \mathrm{~kg}\). is standing stationary with respect to a conveyor belt which is accel- erating with \(1 \mathrm{~m} / \mathrm{s}^{2}\). If \(\mu_{\mathrm{s}}\) is \(\mathbf{0 . 2}\), the net force on the man and the maximum acceleration of the belt so that the man is stationary relative to the belt are \(\left(g=10 \mathrm{~m} / \mathrm{s}^{2}\right)\)

270275 A man of mass\(60 \mathrm{~kg}\) sitting on ice pushes a block of mass of \(12 \mathrm{~kg}\) on ice horizontally with a speed of \(5 \mathrm{~ms}^{-1}\). The coefficient of friction between the man and ice and between block and ice is 0.2 . If \(g=10 \mathrm{~ms}^{-2}\), the distance between man and the block, when they come to rest is

270276 A vehicle of mass\(M\) is moving on a rough horizontal road with a momentum \(P\). If the coefficient of friction between the tyres and the road is \(\mu\), then the stopping distance is (EAM-2012)

270277 The rear side of a truck is open and a box of\(40 \mathrm{~kg}\) mass is placed \(5 \mathrm{~m}\) away from the open end as shown in figure. The coefficient of friction between the box the surface below it is 0.15. On a straight road, the truck starts from rest and accelerates with \(2 \mathrm{~ms}^{-2}\). At what distance from the starting point does the box fall from the truck? (Ignore the size of the box.)