371406 Two Carnot engines are operated in series between three reservoirs at temperatures \({T_1},\;{T_2}\) and \({T_3}\) respectively as shown in figure. First engine receives heat from first reservoir and rejects some amount of heat to the second reservoir. Second engine absorbs the heat rejected from first engine and rejects remaining amount of heat to the third reservoir at \({T_3}.\) Considering the work outputs of the two engines to be equal, calculate the temperature \({T_2}\) .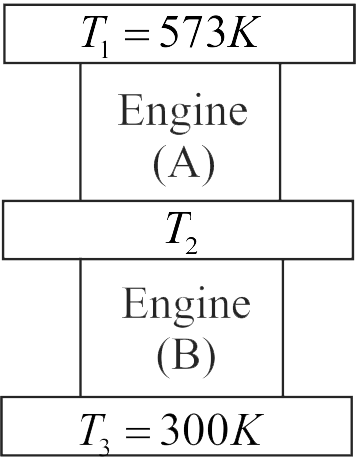

371407 The first operation involved in a carnot cycle is

371408 A Carnot engine operating between temperatures \(T_{1}\) and \(T_{2}\) has efficiency \(\dfrac{1}{6}\) when \(T_{2}\) is lowered by \(62\;K\) its efficiency increases to \(\dfrac{1}{3}\). Then \(T_{1}\) and \(T_{2}\) are, respectively

371409 A Carnot engine working between \({27^{\circ} {C}}\) and \({127^{\circ} {C}}\), draws 600 \(J\) of heat from the reservoir in one cycle. The work done by the engine

371406 Two Carnot engines are operated in series between three reservoirs at temperatures \({T_1},\;{T_2}\) and \({T_3}\) respectively as shown in figure. First engine receives heat from first reservoir and rejects some amount of heat to the second reservoir. Second engine absorbs the heat rejected from first engine and rejects remaining amount of heat to the third reservoir at \({T_3}.\) Considering the work outputs of the two engines to be equal, calculate the temperature \({T_2}\) .

371407 The first operation involved in a carnot cycle is

371408 A Carnot engine operating between temperatures \(T_{1}\) and \(T_{2}\) has efficiency \(\dfrac{1}{6}\) when \(T_{2}\) is lowered by \(62\;K\) its efficiency increases to \(\dfrac{1}{3}\). Then \(T_{1}\) and \(T_{2}\) are, respectively

371409 A Carnot engine working between \({27^{\circ} {C}}\) and \({127^{\circ} {C}}\), draws 600 \(J\) of heat from the reservoir in one cycle. The work done by the engine

371406 Two Carnot engines are operated in series between three reservoirs at temperatures \({T_1},\;{T_2}\) and \({T_3}\) respectively as shown in figure. First engine receives heat from first reservoir and rejects some amount of heat to the second reservoir. Second engine absorbs the heat rejected from first engine and rejects remaining amount of heat to the third reservoir at \({T_3}.\) Considering the work outputs of the two engines to be equal, calculate the temperature \({T_2}\) .

371407 The first operation involved in a carnot cycle is

371408 A Carnot engine operating between temperatures \(T_{1}\) and \(T_{2}\) has efficiency \(\dfrac{1}{6}\) when \(T_{2}\) is lowered by \(62\;K\) its efficiency increases to \(\dfrac{1}{3}\). Then \(T_{1}\) and \(T_{2}\) are, respectively

371409 A Carnot engine working between \({27^{\circ} {C}}\) and \({127^{\circ} {C}}\), draws 600 \(J\) of heat from the reservoir in one cycle. The work done by the engine

371406 Two Carnot engines are operated in series between three reservoirs at temperatures \({T_1},\;{T_2}\) and \({T_3}\) respectively as shown in figure. First engine receives heat from first reservoir and rejects some amount of heat to the second reservoir. Second engine absorbs the heat rejected from first engine and rejects remaining amount of heat to the third reservoir at \({T_3}.\) Considering the work outputs of the two engines to be equal, calculate the temperature \({T_2}\) .

371407 The first operation involved in a carnot cycle is

371408 A Carnot engine operating between temperatures \(T_{1}\) and \(T_{2}\) has efficiency \(\dfrac{1}{6}\) when \(T_{2}\) is lowered by \(62\;K\) its efficiency increases to \(\dfrac{1}{3}\). Then \(T_{1}\) and \(T_{2}\) are, respectively

371409 A Carnot engine working between \({27^{\circ} {C}}\) and \({127^{\circ} {C}}\), draws 600 \(J\) of heat from the reservoir in one cycle. The work done by the engine