367997 Assertion :
The pattern and position of fringes always remains same even after the introduction of transparent medium like glass plate in a path of one of the slit.
Reason :
The central fringe is bright or dark is depends upon the initial phase difference between the two coherence source.

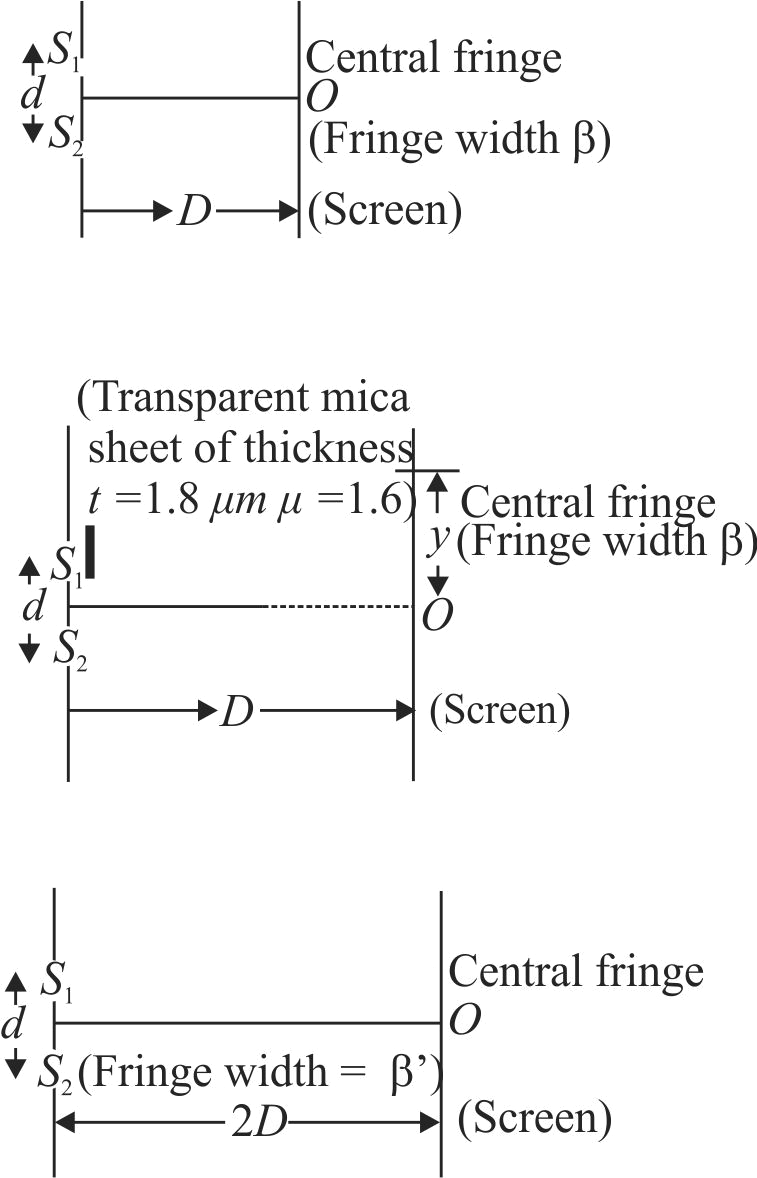
367998 On introducing a thin mica sheet of thickness \({2 \times 10^{-6} {~m}}\) and refractive index 1.5 in the path of one of the waves, central bright maxima shifts by \({n}\) fringes. Wavelength of the wave used is
\(5000\) \( \mathop A^{~~\circ} \), then the value of \({n}\) is

367999 Using monochromatic light of wavelength \(\lambda\), an experimentalist sets up the Young's double slit experiment in three ways as shown. If she observes that \(y=\beta\), the wavelength of light used is

368000 A monochromatic beam of light falls on \(YDSE\) apparatus at some angle (say \(\theta \)) as shown in figure. A thin sheet of glass is inserted infront of the lower slit \({S_2}.\) The central bright fringe (path difference \( = 0)\) will be obtained

368001 In Young’s double slit experiment the distance between the slits and the screen is \(1.2\,m\) and the distance between the two slits is \(2.4\,mm\) If a thin transparent mica sheet of thickness \(1\,\mu m\) and \(R.I.{\rm{ }}1.5\) is introduced between one of the interfering beams, the shift in the position of central bright fringe is

367997 Assertion :
The pattern and position of fringes always remains same even after the introduction of transparent medium like glass plate in a path of one of the slit.
Reason :
The central fringe is bright or dark is depends upon the initial phase difference between the two coherence source.

367998 On introducing a thin mica sheet of thickness \({2 \times 10^{-6} {~m}}\) and refractive index 1.5 in the path of one of the waves, central bright maxima shifts by \({n}\) fringes. Wavelength of the wave used is
\(5000\) \( \mathop A^{~~\circ} \), then the value of \({n}\) is

367999 Using monochromatic light of wavelength \(\lambda\), an experimentalist sets up the Young's double slit experiment in three ways as shown. If she observes that \(y=\beta\), the wavelength of light used is

368000 A monochromatic beam of light falls on \(YDSE\) apparatus at some angle (say \(\theta \)) as shown in figure. A thin sheet of glass is inserted infront of the lower slit \({S_2}.\) The central bright fringe (path difference \( = 0)\) will be obtained

368001 In Young’s double slit experiment the distance between the slits and the screen is \(1.2\,m\) and the distance between the two slits is \(2.4\,mm\) If a thin transparent mica sheet of thickness \(1\,\mu m\) and \(R.I.{\rm{ }}1.5\) is introduced between one of the interfering beams, the shift in the position of central bright fringe is

367997 Assertion :
The pattern and position of fringes always remains same even after the introduction of transparent medium like glass plate in a path of one of the slit.
Reason :
The central fringe is bright or dark is depends upon the initial phase difference between the two coherence source.

367998 On introducing a thin mica sheet of thickness \({2 \times 10^{-6} {~m}}\) and refractive index 1.5 in the path of one of the waves, central bright maxima shifts by \({n}\) fringes. Wavelength of the wave used is
\(5000\) \( \mathop A^{~~\circ} \), then the value of \({n}\) is

367999 Using monochromatic light of wavelength \(\lambda\), an experimentalist sets up the Young's double slit experiment in three ways as shown. If she observes that \(y=\beta\), the wavelength of light used is

368000 A monochromatic beam of light falls on \(YDSE\) apparatus at some angle (say \(\theta \)) as shown in figure. A thin sheet of glass is inserted infront of the lower slit \({S_2}.\) The central bright fringe (path difference \( = 0)\) will be obtained

368001 In Young’s double slit experiment the distance between the slits and the screen is \(1.2\,m\) and the distance between the two slits is \(2.4\,mm\) If a thin transparent mica sheet of thickness \(1\,\mu m\) and \(R.I.{\rm{ }}1.5\) is introduced between one of the interfering beams, the shift in the position of central bright fringe is

367997 Assertion :
The pattern and position of fringes always remains same even after the introduction of transparent medium like glass plate in a path of one of the slit.
Reason :
The central fringe is bright or dark is depends upon the initial phase difference between the two coherence source.

367998 On introducing a thin mica sheet of thickness \({2 \times 10^{-6} {~m}}\) and refractive index 1.5 in the path of one of the waves, central bright maxima shifts by \({n}\) fringes. Wavelength of the wave used is
\(5000\) \( \mathop A^{~~\circ} \), then the value of \({n}\) is

367999 Using monochromatic light of wavelength \(\lambda\), an experimentalist sets up the Young's double slit experiment in three ways as shown. If she observes that \(y=\beta\), the wavelength of light used is

368000 A monochromatic beam of light falls on \(YDSE\) apparatus at some angle (say \(\theta \)) as shown in figure. A thin sheet of glass is inserted infront of the lower slit \({S_2}.\) The central bright fringe (path difference \( = 0)\) will be obtained

368001 In Young’s double slit experiment the distance between the slits and the screen is \(1.2\,m\) and the distance between the two slits is \(2.4\,mm\) If a thin transparent mica sheet of thickness \(1\,\mu m\) and \(R.I.{\rm{ }}1.5\) is introduced between one of the interfering beams, the shift in the position of central bright fringe is

367997 Assertion :
The pattern and position of fringes always remains same even after the introduction of transparent medium like glass plate in a path of one of the slit.
Reason :
The central fringe is bright or dark is depends upon the initial phase difference between the two coherence source.

367998 On introducing a thin mica sheet of thickness \({2 \times 10^{-6} {~m}}\) and refractive index 1.5 in the path of one of the waves, central bright maxima shifts by \({n}\) fringes. Wavelength of the wave used is
\(5000\) \( \mathop A^{~~\circ} \), then the value of \({n}\) is

367999 Using monochromatic light of wavelength \(\lambda\), an experimentalist sets up the Young's double slit experiment in three ways as shown. If she observes that \(y=\beta\), the wavelength of light used is

368000 A monochromatic beam of light falls on \(YDSE\) apparatus at some angle (say \(\theta \)) as shown in figure. A thin sheet of glass is inserted infront of the lower slit \({S_2}.\) The central bright fringe (path difference \( = 0)\) will be obtained

368001 In Young’s double slit experiment the distance between the slits and the screen is \(1.2\,m\) and the distance between the two slits is \(2.4\,mm\) If a thin transparent mica sheet of thickness \(1\,\mu m\) and \(R.I.{\rm{ }}1.5\) is introduced between one of the interfering beams, the shift in the position of central bright fringe is