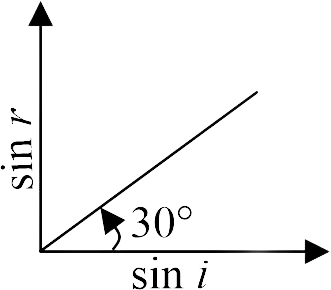
367894 Unpolarised light is incident from air on a plane surface of a material of refractive index \('\mu '\). At a particular angle of incidence ‘i’, it is found that the reflected and refracted rays are perpendicular to each other. Which of the following options is correct for this situation?
367894 Unpolarised light is incident from air on a plane surface of a material of refractive index \('\mu '\). At a particular angle of incidence ‘i’, it is found that the reflected and refracted rays are perpendicular to each other. Which of the following options is correct for this situation?
367894 Unpolarised light is incident from air on a plane surface of a material of refractive index \('\mu '\). At a particular angle of incidence ‘i’, it is found that the reflected and refracted rays are perpendicular to each other. Which of the following options is correct for this situation?
367894 Unpolarised light is incident from air on a plane surface of a material of refractive index \('\mu '\). At a particular angle of incidence ‘i’, it is found that the reflected and refracted rays are perpendicular to each other. Which of the following options is correct for this situation?