367772 White light reflected from a soap film (Refractive index \( = {\rm{ }}1.5)\) has a maximum at \(600\,nm\) and a minimum at \(450\,nm\) with no minimum in between. Then the thickness of the film is \(x \times {10^7}m\). The value x is

367773 The light of wavelength 5890 \( \mathop A^{~~\circ} \) falls normally on a thin air film. The minimum thickness of the film such that the film appears dark in reflected light is:

367774 The path difference between two waves \(y_{1}=A_{1} \sin \omega t \quad\) and \(\quad y_{2}=A_{2} \cos (\omega t+\phi)\) when they arrive at a given point will be

367775 Two light rays initially in same phase travel through two media of equal length \(L\) having refractive index \({\mu _1}\) and \({\mu _2}\) \(\left( {{\mu _1} > {\mu _2}} \right)\) as shown in the figure. If the wavelength of light ray in air is \(\lambda \), the phase difference of the emerging rays is given by

367772 White light reflected from a soap film (Refractive index \( = {\rm{ }}1.5)\) has a maximum at \(600\,nm\) and a minimum at \(450\,nm\) with no minimum in between. Then the thickness of the film is \(x \times {10^7}m\). The value x is

367773 The light of wavelength 5890 \( \mathop A^{~~\circ} \) falls normally on a thin air film. The minimum thickness of the film such that the film appears dark in reflected light is:

367774 The path difference between two waves \(y_{1}=A_{1} \sin \omega t \quad\) and \(\quad y_{2}=A_{2} \cos (\omega t+\phi)\) when they arrive at a given point will be

367775 Two light rays initially in same phase travel through two media of equal length \(L\) having refractive index \({\mu _1}\) and \({\mu _2}\) \(\left( {{\mu _1} > {\mu _2}} \right)\) as shown in the figure. If the wavelength of light ray in air is \(\lambda \), the phase difference of the emerging rays is given by

367772 White light reflected from a soap film (Refractive index \( = {\rm{ }}1.5)\) has a maximum at \(600\,nm\) and a minimum at \(450\,nm\) with no minimum in between. Then the thickness of the film is \(x \times {10^7}m\). The value x is

367773 The light of wavelength 5890 \( \mathop A^{~~\circ} \) falls normally on a thin air film. The minimum thickness of the film such that the film appears dark in reflected light is:

367774 The path difference between two waves \(y_{1}=A_{1} \sin \omega t \quad\) and \(\quad y_{2}=A_{2} \cos (\omega t+\phi)\) when they arrive at a given point will be

367775 Two light rays initially in same phase travel through two media of equal length \(L\) having refractive index \({\mu _1}\) and \({\mu _2}\) \(\left( {{\mu _1} > {\mu _2}} \right)\) as shown in the figure. If the wavelength of light ray in air is \(\lambda \), the phase difference of the emerging rays is given by

367772 White light reflected from a soap film (Refractive index \( = {\rm{ }}1.5)\) has a maximum at \(600\,nm\) and a minimum at \(450\,nm\) with no minimum in between. Then the thickness of the film is \(x \times {10^7}m\). The value x is

367773 The light of wavelength 5890 \( \mathop A^{~~\circ} \) falls normally on a thin air film. The minimum thickness of the film such that the film appears dark in reflected light is:

367774 The path difference between two waves \(y_{1}=A_{1} \sin \omega t \quad\) and \(\quad y_{2}=A_{2} \cos (\omega t+\phi)\) when they arrive at a given point will be

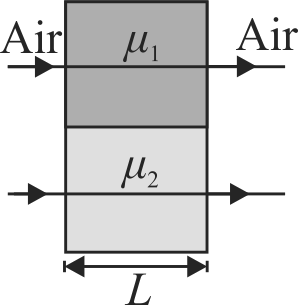
367775 Two light rays initially in same phase travel through two media of equal length \(L\) having refractive index \({\mu _1}\) and \({\mu _2}\) \(\left( {{\mu _1} > {\mu _2}} \right)\) as shown in the figure. If the wavelength of light ray in air is \(\lambda \), the phase difference of the emerging rays is given by