363277
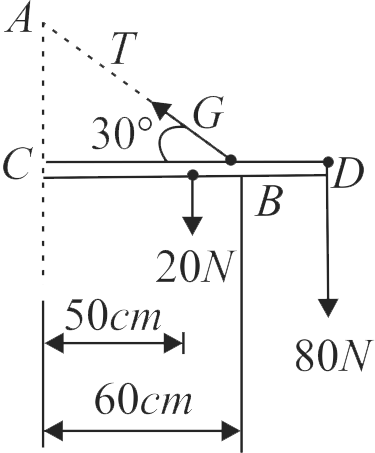
An object of mass \(8\;kg\) is hanging from one end of a uniform rod \(C D\) of mass \(2\;kg\) and length \(1\;m\) pivoted at its end \(C\) on a vertical wall as shown in figure. It is supported by a cable \(A B\) such that the system is in equilibrium. The tension in the cable is (Take \({g = 10\;m{\rm{/}}{s^2}}\))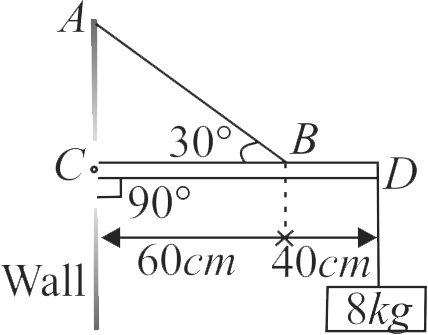
363279
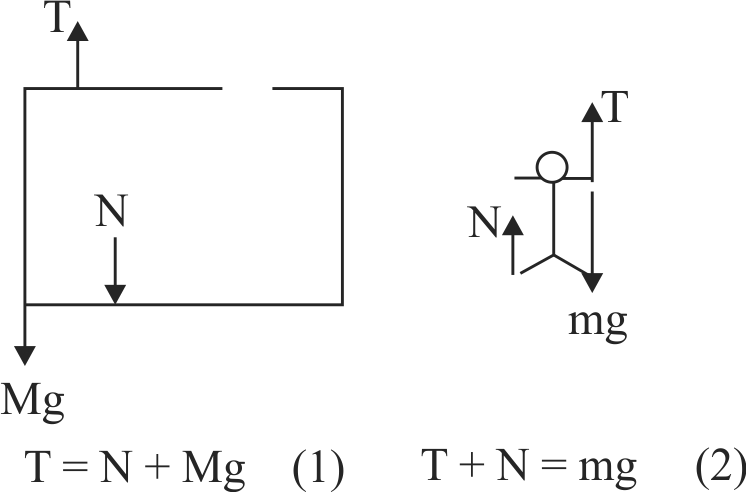
Figure shows a man of mass 50 \(kg\) standing on a light weighing machine kept in a box of mass 30 \(kg\). The box is hanging from a pulley fixed to the ceiling through a light rope, the other end of which is held by the man himself. If the man manages to keep the box at rest, the weight shown by the machine is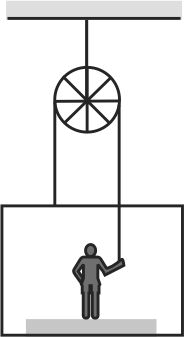
363277
An object of mass \(8\;kg\) is hanging from one end of a uniform rod \(C D\) of mass \(2\;kg\) and length \(1\;m\) pivoted at its end \(C\) on a vertical wall as shown in figure. It is supported by a cable \(A B\) such that the system is in equilibrium. The tension in the cable is (Take \({g = 10\;m{\rm{/}}{s^2}}\))
363279
Figure shows a man of mass 50 \(kg\) standing on a light weighing machine kept in a box of mass 30 \(kg\). The box is hanging from a pulley fixed to the ceiling through a light rope, the other end of which is held by the man himself. If the man manages to keep the box at rest, the weight shown by the machine is
363277
An object of mass \(8\;kg\) is hanging from one end of a uniform rod \(C D\) of mass \(2\;kg\) and length \(1\;m\) pivoted at its end \(C\) on a vertical wall as shown in figure. It is supported by a cable \(A B\) such that the system is in equilibrium. The tension in the cable is (Take \({g = 10\;m{\rm{/}}{s^2}}\))
363279
Figure shows a man of mass 50 \(kg\) standing on a light weighing machine kept in a box of mass 30 \(kg\). The box is hanging from a pulley fixed to the ceiling through a light rope, the other end of which is held by the man himself. If the man manages to keep the box at rest, the weight shown by the machine is
363277
An object of mass \(8\;kg\) is hanging from one end of a uniform rod \(C D\) of mass \(2\;kg\) and length \(1\;m\) pivoted at its end \(C\) on a vertical wall as shown in figure. It is supported by a cable \(A B\) such that the system is in equilibrium. The tension in the cable is (Take \({g = 10\;m{\rm{/}}{s^2}}\))
363279
Figure shows a man of mass 50 \(kg\) standing on a light weighing machine kept in a box of mass 30 \(kg\). The box is hanging from a pulley fixed to the ceiling through a light rope, the other end of which is held by the man himself. If the man manages to keep the box at rest, the weight shown by the machine is