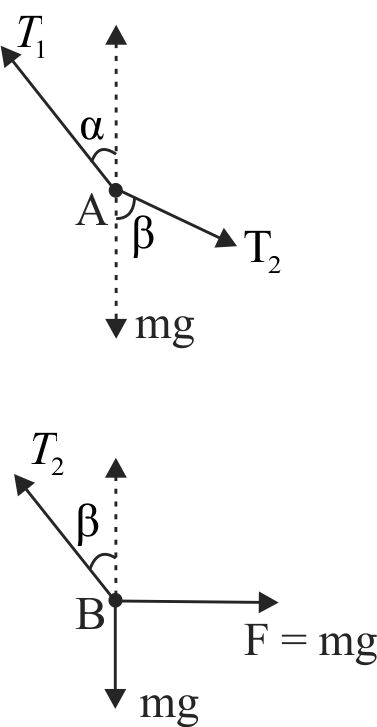
Explanation:
\({T_2}\cos \beta = mg\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,(1)\)
\({T_2}\sin \beta = F = mg\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,(2)\)
From equations (1) and (2), \(\beta = 45^\circ \)
\({T_2}\cos \beta + mg = {T_1}\cos \alpha \,\,\,\,\,(3)\)
\({T_2}\sin \beta = {T_1}\sin \alpha \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,(4)\)
Dividing equation (4) and (3)
\(\tan \alpha = 1/2\)
\( \Rightarrow \sin \alpha = 1/\sqrt 5 \)
From equation (4), \({T_2}\frac{1}{{\sqrt 2 }} = {T_1}\frac{1}{{\sqrt 5 }}\)
\( \Rightarrow \,\,\,\,\sqrt 5 {T_2} = \sqrt 2 {T_1}\)