363013 The dipole moment of a circular loop carrying a current \(I\), is \(m\) and the magnetic field at the centre of the loop is \(B_{1}\). Where the dipole moment is doubled by keeping the current constant, the magnetic field at the centre of the loop is \(B_{2}\). The ratio \(\dfrac{B_{1}}{B_{2}}\) is:
363014
A uniform conducting wire of length \(12 a\) and resistance ' \(R\) ' is wound up as a current carrying coil in the shape of
(i) an equilateral triangle of side ' \(a\) '
(ii) a square of side ' \(a\) 'The magnetic dipole moments of the coil in each case respectively are
363013 The dipole moment of a circular loop carrying a current \(I\), is \(m\) and the magnetic field at the centre of the loop is \(B_{1}\). Where the dipole moment is doubled by keeping the current constant, the magnetic field at the centre of the loop is \(B_{2}\). The ratio \(\dfrac{B_{1}}{B_{2}}\) is:
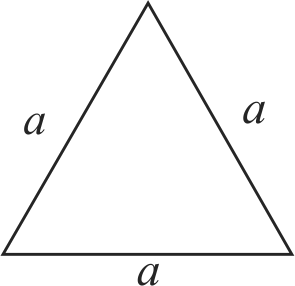
363014
A uniform conducting wire of length \(12 a\) and resistance ' \(R\) ' is wound up as a current carrying coil in the shape of
(i) an equilateral triangle of side ' \(a\) '
(ii) a square of side ' \(a\) 'The magnetic dipole moments of the coil in each case respectively are
363013 The dipole moment of a circular loop carrying a current \(I\), is \(m\) and the magnetic field at the centre of the loop is \(B_{1}\). Where the dipole moment is doubled by keeping the current constant, the magnetic field at the centre of the loop is \(B_{2}\). The ratio \(\dfrac{B_{1}}{B_{2}}\) is:
363014
A uniform conducting wire of length \(12 a\) and resistance ' \(R\) ' is wound up as a current carrying coil in the shape of
(i) an equilateral triangle of side ' \(a\) '
(ii) a square of side ' \(a\) 'The magnetic dipole moments of the coil in each case respectively are
363013 The dipole moment of a circular loop carrying a current \(I\), is \(m\) and the magnetic field at the centre of the loop is \(B_{1}\). Where the dipole moment is doubled by keeping the current constant, the magnetic field at the centre of the loop is \(B_{2}\). The ratio \(\dfrac{B_{1}}{B_{2}}\) is:
363014
A uniform conducting wire of length \(12 a\) and resistance ' \(R\) ' is wound up as a current carrying coil in the shape of
(i) an equilateral triangle of side ' \(a\) '
(ii) a square of side ' \(a\) 'The magnetic dipole moments of the coil in each case respectively are