363049 A circular coil of 16 turns and radius 10\(cm\) carrying a current of 0.75\(A\) rests with its plane normal to an external field of magnitude \(5.0 \times {10^{ - 2}}\;T\). The coil is free to turn about an axis in its plane perpendicular to the field direction. When the coil is turned slightly and released, it oscillates about its stable equilibrium with a frequency of \(2.0\;{s^{ - 1}}\). The moment of inertia of the coil about its axis of rotation is (in \(kg - {m^2}\))
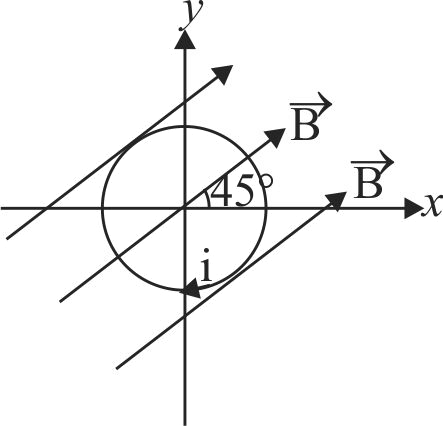
363052 A circular loop of radius \(R\) carries 1\(A\) of current in clockwise direction lie on \(x-y\) plane. The torque experienced by this loop due to a uniform magnetic field whose strength \(B_{o}\) making an angle \(45^{\circ}\) with \(\mathrm{x}\)-axis in \(\mathrm{x}-\mathrm{y}\) plane is
363049 A circular coil of 16 turns and radius 10\(cm\) carrying a current of 0.75\(A\) rests with its plane normal to an external field of magnitude \(5.0 \times {10^{ - 2}}\;T\). The coil is free to turn about an axis in its plane perpendicular to the field direction. When the coil is turned slightly and released, it oscillates about its stable equilibrium with a frequency of \(2.0\;{s^{ - 1}}\). The moment of inertia of the coil about its axis of rotation is (in \(kg - {m^2}\))
363052 A circular loop of radius \(R\) carries 1\(A\) of current in clockwise direction lie on \(x-y\) plane. The torque experienced by this loop due to a uniform magnetic field whose strength \(B_{o}\) making an angle \(45^{\circ}\) with \(\mathrm{x}\)-axis in \(\mathrm{x}-\mathrm{y}\) plane is
363049 A circular coil of 16 turns and radius 10\(cm\) carrying a current of 0.75\(A\) rests with its plane normal to an external field of magnitude \(5.0 \times {10^{ - 2}}\;T\). The coil is free to turn about an axis in its plane perpendicular to the field direction. When the coil is turned slightly and released, it oscillates about its stable equilibrium with a frequency of \(2.0\;{s^{ - 1}}\). The moment of inertia of the coil about its axis of rotation is (in \(kg - {m^2}\))
363052 A circular loop of radius \(R\) carries 1\(A\) of current in clockwise direction lie on \(x-y\) plane. The torque experienced by this loop due to a uniform magnetic field whose strength \(B_{o}\) making an angle \(45^{\circ}\) with \(\mathrm{x}\)-axis in \(\mathrm{x}-\mathrm{y}\) plane is
363049 A circular coil of 16 turns and radius 10\(cm\) carrying a current of 0.75\(A\) rests with its plane normal to an external field of magnitude \(5.0 \times {10^{ - 2}}\;T\). The coil is free to turn about an axis in its plane perpendicular to the field direction. When the coil is turned slightly and released, it oscillates about its stable equilibrium with a frequency of \(2.0\;{s^{ - 1}}\). The moment of inertia of the coil about its axis of rotation is (in \(kg - {m^2}\))
363052 A circular loop of radius \(R\) carries 1\(A\) of current in clockwise direction lie on \(x-y\) plane. The torque experienced by this loop due to a uniform magnetic field whose strength \(B_{o}\) making an angle \(45^{\circ}\) with \(\mathrm{x}\)-axis in \(\mathrm{x}-\mathrm{y}\) plane is
363049 A circular coil of 16 turns and radius 10\(cm\) carrying a current of 0.75\(A\) rests with its plane normal to an external field of magnitude \(5.0 \times {10^{ - 2}}\;T\). The coil is free to turn about an axis in its plane perpendicular to the field direction. When the coil is turned slightly and released, it oscillates about its stable equilibrium with a frequency of \(2.0\;{s^{ - 1}}\). The moment of inertia of the coil about its axis of rotation is (in \(kg - {m^2}\))
363052 A circular loop of radius \(R\) carries 1\(A\) of current in clockwise direction lie on \(x-y\) plane. The torque experienced by this loop due to a uniform magnetic field whose strength \(B_{o}\) making an angle \(45^{\circ}\) with \(\mathrm{x}\)-axis in \(\mathrm{x}-\mathrm{y}\) plane is