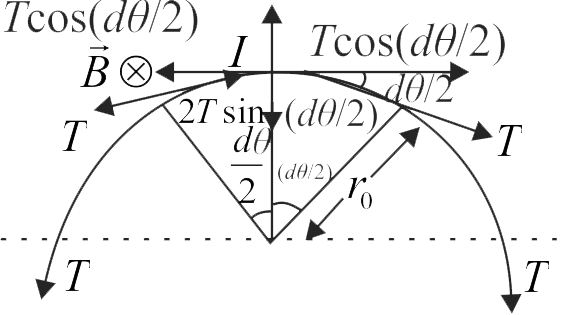
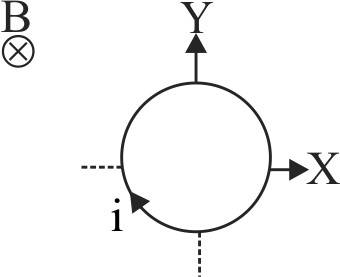
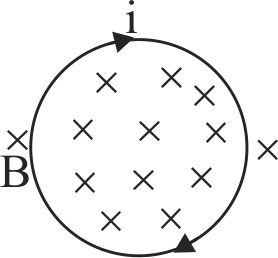
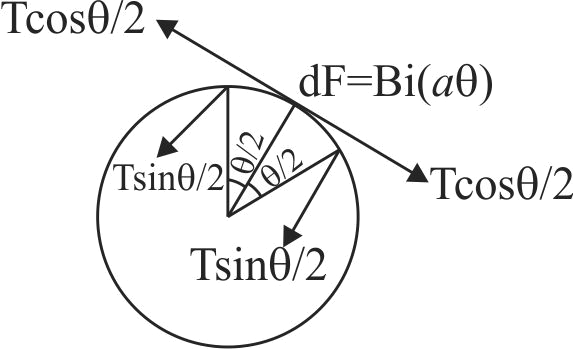
362754 A current \(I = 3\) A flows in a ring of radius \({r_0} = 20\;cm\) made of a very thin wire. The tensile strength of the wire is equal to \({T_s} = 1.5\;N\). The ring is placed in a magnetic field, which is perpendicular to the plane of the ring so that the forces tend to break the ring. The magnetic field at which the ring is broken would be
362757
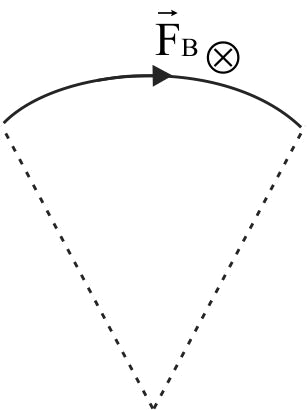
A current-carrying loop is placed in a uniform magnetic field pointing in negative \({z}\)-direction. Branch \({P Q R S}\) is a three quarter circle, while branch \({P S}\) is straight. If force on branch \({P S}\) is \({F}\), force on branch \({P Q R}\) is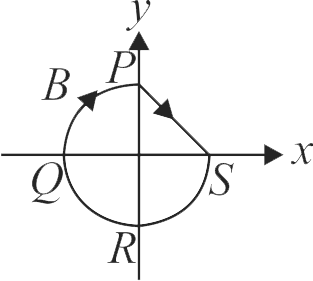
362754 A current \(I = 3\) A flows in a ring of radius \({r_0} = 20\;cm\) made of a very thin wire. The tensile strength of the wire is equal to \({T_s} = 1.5\;N\). The ring is placed in a magnetic field, which is perpendicular to the plane of the ring so that the forces tend to break the ring. The magnetic field at which the ring is broken would be
362757
A current-carrying loop is placed in a uniform magnetic field pointing in negative \({z}\)-direction. Branch \({P Q R S}\) is a three quarter circle, while branch \({P S}\) is straight. If force on branch \({P S}\) is \({F}\), force on branch \({P Q R}\) is
362754 A current \(I = 3\) A flows in a ring of radius \({r_0} = 20\;cm\) made of a very thin wire. The tensile strength of the wire is equal to \({T_s} = 1.5\;N\). The ring is placed in a magnetic field, which is perpendicular to the plane of the ring so that the forces tend to break the ring. The magnetic field at which the ring is broken would be
362757
A current-carrying loop is placed in a uniform magnetic field pointing in negative \({z}\)-direction. Branch \({P Q R S}\) is a three quarter circle, while branch \({P S}\) is straight. If force on branch \({P S}\) is \({F}\), force on branch \({P Q R}\) is
362754 A current \(I = 3\) A flows in a ring of radius \({r_0} = 20\;cm\) made of a very thin wire. The tensile strength of the wire is equal to \({T_s} = 1.5\;N\). The ring is placed in a magnetic field, which is perpendicular to the plane of the ring so that the forces tend to break the ring. The magnetic field at which the ring is broken would be
362757
A current-carrying loop is placed in a uniform magnetic field pointing in negative \({z}\)-direction. Branch \({P Q R S}\) is a three quarter circle, while branch \({P S}\) is straight. If force on branch \({P S}\) is \({F}\), force on branch \({P Q R}\) is
362754 A current \(I = 3\) A flows in a ring of radius \({r_0} = 20\;cm\) made of a very thin wire. The tensile strength of the wire is equal to \({T_s} = 1.5\;N\). The ring is placed in a magnetic field, which is perpendicular to the plane of the ring so that the forces tend to break the ring. The magnetic field at which the ring is broken would be
362757
A current-carrying loop is placed in a uniform magnetic field pointing in negative \({z}\)-direction. Branch \({P Q R S}\) is a three quarter circle, while branch \({P S}\) is straight. If force on branch \({P S}\) is \({F}\), force on branch \({P Q R}\) is