362742 A metal wire of mass \(m\) slides without friction on two horizontal rails placed at a distance \(l\) apart. The track lies in a uniform vertical magnetic field \(B\). A constant current i flows along the rails across the wire to the other rail. The acceleration of the wire is
362743
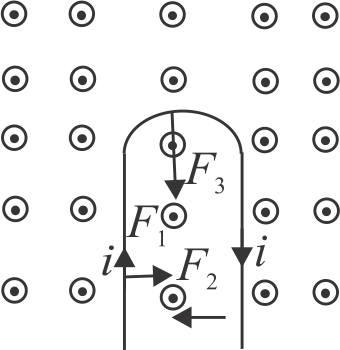
A rigid wire consists of a semicircular portion of radius \(R\) and two straight sections. The wire is partially immersed in a perpendicular magnetic field \(\vec{B}=B_{0} \hat{j}\) as shown in figure. The magnetic force on the wire if it has a current \(i\) is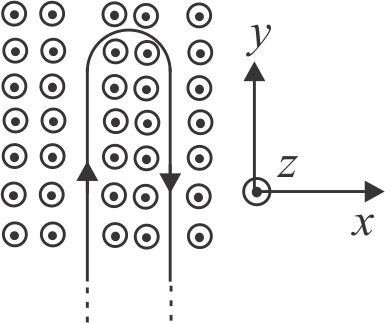
362742 A metal wire of mass \(m\) slides without friction on two horizontal rails placed at a distance \(l\) apart. The track lies in a uniform vertical magnetic field \(B\). A constant current i flows along the rails across the wire to the other rail. The acceleration of the wire is
362743
A rigid wire consists of a semicircular portion of radius \(R\) and two straight sections. The wire is partially immersed in a perpendicular magnetic field \(\vec{B}=B_{0} \hat{j}\) as shown in figure. The magnetic force on the wire if it has a current \(i\) is
362742 A metal wire of mass \(m\) slides without friction on two horizontal rails placed at a distance \(l\) apart. The track lies in a uniform vertical magnetic field \(B\). A constant current i flows along the rails across the wire to the other rail. The acceleration of the wire is
362743
A rigid wire consists of a semicircular portion of radius \(R\) and two straight sections. The wire is partially immersed in a perpendicular magnetic field \(\vec{B}=B_{0} \hat{j}\) as shown in figure. The magnetic force on the wire if it has a current \(i\) is
362742 A metal wire of mass \(m\) slides without friction on two horizontal rails placed at a distance \(l\) apart. The track lies in a uniform vertical magnetic field \(B\). A constant current i flows along the rails across the wire to the other rail. The acceleration of the wire is
362743
A rigid wire consists of a semicircular portion of radius \(R\) and two straight sections. The wire is partially immersed in a perpendicular magnetic field \(\vec{B}=B_{0} \hat{j}\) as shown in figure. The magnetic force on the wire if it has a current \(i\) is