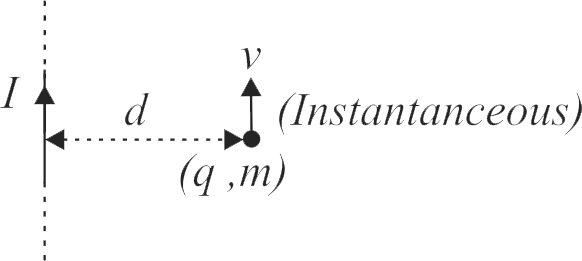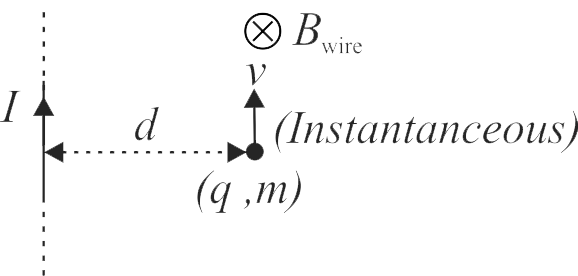362659
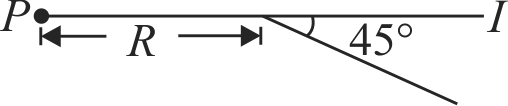
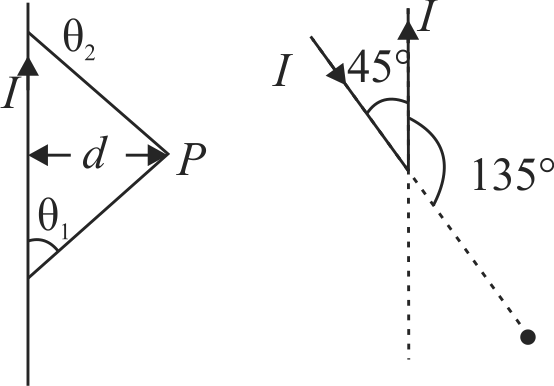
As shown in figure, two infinitely long, identical wires are bent by \(90^{\circ}\) and placed in such a way that the segments \(LP\) and \(QM\) are along \(x\)-axis, while segments \(PS\) and \(QN\) are parallel to \(y\)-axis. If \(OP = OQ = 5\;cm\), and the magnitude of the magnetic field at \(O\) is \({10^{ - 5}}\;T,\) and the two wires carry equal currents (see figure), the magnitude of current in each wire is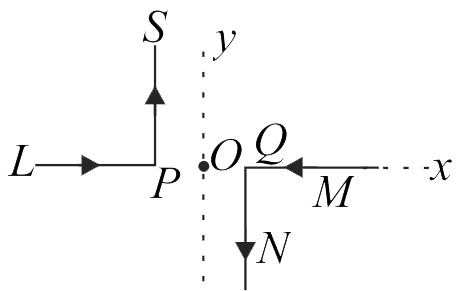
362659
As shown in figure, two infinitely long, identical wires are bent by \(90^{\circ}\) and placed in such a way that the segments \(LP\) and \(QM\) are along \(x\)-axis, while segments \(PS\) and \(QN\) are parallel to \(y\)-axis. If \(OP = OQ = 5\;cm\), and the magnitude of the magnetic field at \(O\) is \({10^{ - 5}}\;T,\) and the two wires carry equal currents (see figure), the magnitude of current in each wire is
362659
As shown in figure, two infinitely long, identical wires are bent by \(90^{\circ}\) and placed in such a way that the segments \(LP\) and \(QM\) are along \(x\)-axis, while segments \(PS\) and \(QN\) are parallel to \(y\)-axis. If \(OP = OQ = 5\;cm\), and the magnitude of the magnetic field at \(O\) is \({10^{ - 5}}\;T,\) and the two wires carry equal currents (see figure), the magnitude of current in each wire is
362659
As shown in figure, two infinitely long, identical wires are bent by \(90^{\circ}\) and placed in such a way that the segments \(LP\) and \(QM\) are along \(x\)-axis, while segments \(PS\) and \(QN\) are parallel to \(y\)-axis. If \(OP = OQ = 5\;cm\), and the magnitude of the magnetic field at \(O\) is \({10^{ - 5}}\;T,\) and the two wires carry equal currents (see figure), the magnitude of current in each wire is