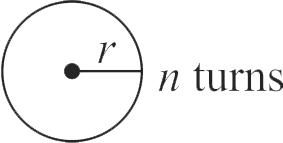
362580 A long conducting wire having a current \(I\) flowing through it. It is bent into a circular coil of \(N\) turns. Then it is bent into a circular coil of \(n\) turns. The magnetic field is calculated at the centre of coils in both the cases. The ratio of the magnetic field in first case to that of second case is
362580 A long conducting wire having a current \(I\) flowing through it. It is bent into a circular coil of \(N\) turns. Then it is bent into a circular coil of \(n\) turns. The magnetic field is calculated at the centre of coils in both the cases. The ratio of the magnetic field in first case to that of second case is
362580 A long conducting wire having a current \(I\) flowing through it. It is bent into a circular coil of \(N\) turns. Then it is bent into a circular coil of \(n\) turns. The magnetic field is calculated at the centre of coils in both the cases. The ratio of the magnetic field in first case to that of second case is
362580 A long conducting wire having a current \(I\) flowing through it. It is bent into a circular coil of \(N\) turns. Then it is bent into a circular coil of \(n\) turns. The magnetic field is calculated at the centre of coils in both the cases. The ratio of the magnetic field in first case to that of second case is