361330
A thin plate of area \(2\;\,{m^2}\) is moving between two fixed parallel surfaces as shown in the figure. The liquid present on both sides of the plate is same and has coefficient of viscosity \(\eta=0.01\) poise. Find the external force require to make the plate to move with constant velocity \(2\;m/s\).
361332
A metal plate of area \(0.2\;{m^2}\) is connected to a \(0.01\;kg\) of mass via a string that passes over an ideal pulley.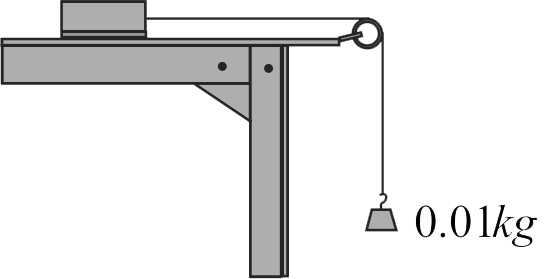
A liquid with a film thickness of \(0.4\,\,mm\) is placed between the plate and the table as shown in figure above. When released, the plate moves to the right with a constant speed of \(0.08\,\,m{s^{ - 1}}.\) The coefficient of viscosity of the liquid is \(n \times {10^{ - 3}}Pa - s.\) Find \(n\).
(Take \(g = 10\;m{\rm{/}}{s^2}\))
361330
A thin plate of area \(2\;\,{m^2}\) is moving between two fixed parallel surfaces as shown in the figure. The liquid present on both sides of the plate is same and has coefficient of viscosity \(\eta=0.01\) poise. Find the external force require to make the plate to move with constant velocity \(2\;m/s\).
361332
A metal plate of area \(0.2\;{m^2}\) is connected to a \(0.01\;kg\) of mass via a string that passes over an ideal pulley.
A liquid with a film thickness of \(0.4\,\,mm\) is placed between the plate and the table as shown in figure above. When released, the plate moves to the right with a constant speed of \(0.08\,\,m{s^{ - 1}}.\) The coefficient of viscosity of the liquid is \(n \times {10^{ - 3}}Pa - s.\) Find \(n\).
(Take \(g = 10\;m{\rm{/}}{s^2}\))
361330
A thin plate of area \(2\;\,{m^2}\) is moving between two fixed parallel surfaces as shown in the figure. The liquid present on both sides of the plate is same and has coefficient of viscosity \(\eta=0.01\) poise. Find the external force require to make the plate to move with constant velocity \(2\;m/s\).
361332
A metal plate of area \(0.2\;{m^2}\) is connected to a \(0.01\;kg\) of mass via a string that passes over an ideal pulley.
A liquid with a film thickness of \(0.4\,\,mm\) is placed between the plate and the table as shown in figure above. When released, the plate moves to the right with a constant speed of \(0.08\,\,m{s^{ - 1}}.\) The coefficient of viscosity of the liquid is \(n \times {10^{ - 3}}Pa - s.\) Find \(n\).
(Take \(g = 10\;m{\rm{/}}{s^2}\))
361330
A thin plate of area \(2\;\,{m^2}\) is moving between two fixed parallel surfaces as shown in the figure. The liquid present on both sides of the plate is same and has coefficient of viscosity \(\eta=0.01\) poise. Find the external force require to make the plate to move with constant velocity \(2\;m/s\).
361332
A metal plate of area \(0.2\;{m^2}\) is connected to a \(0.01\;kg\) of mass via a string that passes over an ideal pulley.
A liquid with a film thickness of \(0.4\,\,mm\) is placed between the plate and the table as shown in figure above. When released, the plate moves to the right with a constant speed of \(0.08\,\,m{s^{ - 1}}.\) The coefficient of viscosity of the liquid is \(n \times {10^{ - 3}}Pa - s.\) Find \(n\).
(Take \(g = 10\;m{\rm{/}}{s^2}\))
