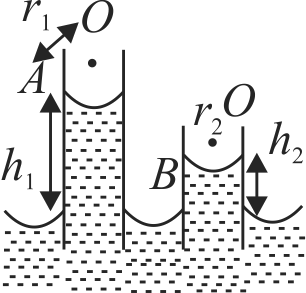
361161 When one end of the capillary is dipped in water, the height of water column is ' \(h\) '. The upward force of 105 dyne due to surface tension is balanced by the force due to the weight of water column. The inner circumference of the capillary is ( surface tension of water \( = 7 \times {10^{ - 2}}\;N/m\) )
361161 When one end of the capillary is dipped in water, the height of water column is ' \(h\) '. The upward force of 105 dyne due to surface tension is balanced by the force due to the weight of water column. The inner circumference of the capillary is ( surface tension of water \( = 7 \times {10^{ - 2}}\;N/m\) )
361161 When one end of the capillary is dipped in water, the height of water column is ' \(h\) '. The upward force of 105 dyne due to surface tension is balanced by the force due to the weight of water column. The inner circumference of the capillary is ( surface tension of water \( = 7 \times {10^{ - 2}}\;N/m\) )
361161 When one end of the capillary is dipped in water, the height of water column is ' \(h\) '. The upward force of 105 dyne due to surface tension is balanced by the force due to the weight of water column. The inner circumference of the capillary is ( surface tension of water \( = 7 \times {10^{ - 2}}\;N/m\) )