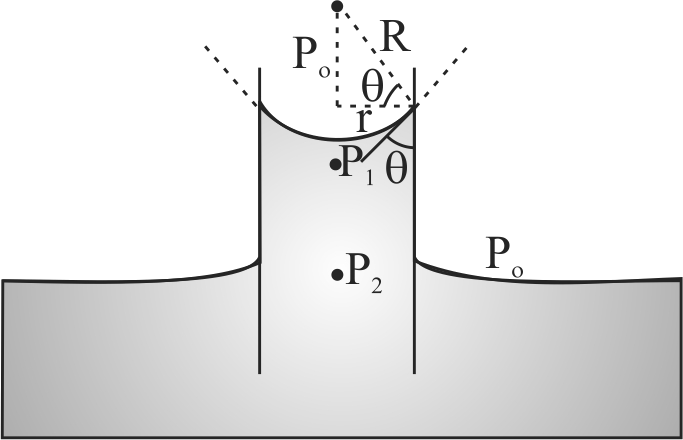
361147 A long capillary tube of radius \(0.2\,\;mm\) is placed vertically inside a beaker of water. The surface tension of water is \(7 \times {10^{ - 2}}\;N/m\) and the angle of contact between glass and water is zero, if the tube is pushed into water so that only 5.0 \(cm\) of its length is above the surface, then determine the angle of contact between the liquid and glass surface.
361148 Water rises to a height of \(100\,\;cm\) in a capillary tube and mercury falls to a depth of \(3.42\,\;cm\) in the same capillary tube. If the density of mercury is \(13.6\;g/c{m^3}\) and the angles of contact for mercury and water are \(135^{\circ}\) and \(0^{\circ}\) respectively, the ratio of the surface tension for water and mercury is
361147 A long capillary tube of radius \(0.2\,\;mm\) is placed vertically inside a beaker of water. The surface tension of water is \(7 \times {10^{ - 2}}\;N/m\) and the angle of contact between glass and water is zero, if the tube is pushed into water so that only 5.0 \(cm\) of its length is above the surface, then determine the angle of contact between the liquid and glass surface.
361148 Water rises to a height of \(100\,\;cm\) in a capillary tube and mercury falls to a depth of \(3.42\,\;cm\) in the same capillary tube. If the density of mercury is \(13.6\;g/c{m^3}\) and the angles of contact for mercury and water are \(135^{\circ}\) and \(0^{\circ}\) respectively, the ratio of the surface tension for water and mercury is
361147 A long capillary tube of radius \(0.2\,\;mm\) is placed vertically inside a beaker of water. The surface tension of water is \(7 \times {10^{ - 2}}\;N/m\) and the angle of contact between glass and water is zero, if the tube is pushed into water so that only 5.0 \(cm\) of its length is above the surface, then determine the angle of contact between the liquid and glass surface.
361148 Water rises to a height of \(100\,\;cm\) in a capillary tube and mercury falls to a depth of \(3.42\,\;cm\) in the same capillary tube. If the density of mercury is \(13.6\;g/c{m^3}\) and the angles of contact for mercury and water are \(135^{\circ}\) and \(0^{\circ}\) respectively, the ratio of the surface tension for water and mercury is
361147 A long capillary tube of radius \(0.2\,\;mm\) is placed vertically inside a beaker of water. The surface tension of water is \(7 \times {10^{ - 2}}\;N/m\) and the angle of contact between glass and water is zero, if the tube is pushed into water so that only 5.0 \(cm\) of its length is above the surface, then determine the angle of contact between the liquid and glass surface.
361148 Water rises to a height of \(100\,\;cm\) in a capillary tube and mercury falls to a depth of \(3.42\,\;cm\) in the same capillary tube. If the density of mercury is \(13.6\;g/c{m^3}\) and the angles of contact for mercury and water are \(135^{\circ}\) and \(0^{\circ}\) respectively, the ratio of the surface tension for water and mercury is