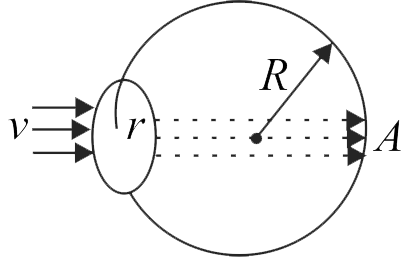
361242 A bubble having surface tension \(T\) and radius \(R\) is formed on ring of radius \(r(r \ll R)\). Air of density \(\rho\) is blown inside the tube with velocity \(v\) such that air molecule collides perpendicularly with the wall of the bubble and stops. The radius at which the bubble separates from the ring is \(\frac{{ZT}}{{\rho {v^2}}}.\) Find \(Z\).
361244 A capillary tube is immersed vertically in water and the height of the water column is \(x\). When this arrangement is taken into a mine of depth \(d\), the height of the water column is \(y\). If \(R\) is the radius of earth, the ratio \(\dfrac{x}{y}\) is :
361242 A bubble having surface tension \(T\) and radius \(R\) is formed on ring of radius \(r(r \ll R)\). Air of density \(\rho\) is blown inside the tube with velocity \(v\) such that air molecule collides perpendicularly with the wall of the bubble and stops. The radius at which the bubble separates from the ring is \(\frac{{ZT}}{{\rho {v^2}}}.\) Find \(Z\).
361244 A capillary tube is immersed vertically in water and the height of the water column is \(x\). When this arrangement is taken into a mine of depth \(d\), the height of the water column is \(y\). If \(R\) is the radius of earth, the ratio \(\dfrac{x}{y}\) is :
361242 A bubble having surface tension \(T\) and radius \(R\) is formed on ring of radius \(r(r \ll R)\). Air of density \(\rho\) is blown inside the tube with velocity \(v\) such that air molecule collides perpendicularly with the wall of the bubble and stops. The radius at which the bubble separates from the ring is \(\frac{{ZT}}{{\rho {v^2}}}.\) Find \(Z\).
361244 A capillary tube is immersed vertically in water and the height of the water column is \(x\). When this arrangement is taken into a mine of depth \(d\), the height of the water column is \(y\). If \(R\) is the radius of earth, the ratio \(\dfrac{x}{y}\) is :
361242 A bubble having surface tension \(T\) and radius \(R\) is formed on ring of radius \(r(r \ll R)\). Air of density \(\rho\) is blown inside the tube with velocity \(v\) such that air molecule collides perpendicularly with the wall of the bubble and stops. The radius at which the bubble separates from the ring is \(\frac{{ZT}}{{\rho {v^2}}}.\) Find \(Z\).
361244 A capillary tube is immersed vertically in water and the height of the water column is \(x\). When this arrangement is taken into a mine of depth \(d\), the height of the water column is \(y\). If \(R\) is the radius of earth, the ratio \(\dfrac{x}{y}\) is :
361242 A bubble having surface tension \(T\) and radius \(R\) is formed on ring of radius \(r(r \ll R)\). Air of density \(\rho\) is blown inside the tube with velocity \(v\) such that air molecule collides perpendicularly with the wall of the bubble and stops. The radius at which the bubble separates from the ring is \(\frac{{ZT}}{{\rho {v^2}}}.\) Find \(Z\).
361244 A capillary tube is immersed vertically in water and the height of the water column is \(x\). When this arrangement is taken into a mine of depth \(d\), the height of the water column is \(y\). If \(R\) is the radius of earth, the ratio \(\dfrac{x}{y}\) is :