361014 The volume of the hollow portion of a sphere is \(\dfrac{3}{4}\) of the external volume of the sphere. If it floats in a liquid of relative density \(\dfrac{3}{2}\), half of its external volume immersed, the relative density of the material of the solid is:
361017
The weight indicated on a balance is \(X\) when a beaker of water is placed on it. A solid object has weight \(Y\) in air and displaces weight \(Z\) of water when completely immersed.The given diagram shows the object suspended from a light string and completely immersed in the beaker of water. what is the balance reading in the given arrangement ?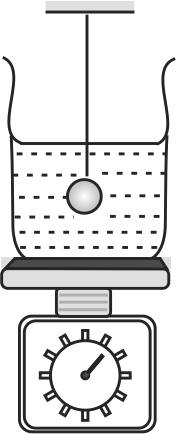
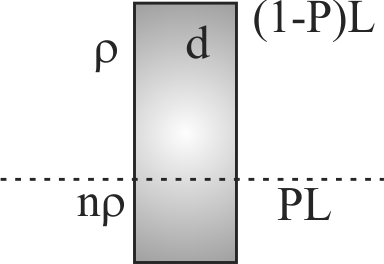
361018 Two non-mixing liquid of densities \(\rho\) and \(n \rho(\mathrm{n}>1)\) are put in a container. The height of each liquid is \(h\). A solid cylinder of length \(L\) and density \(d\) is put in this container. The cylinder floats with its axis vertical and length \(\mathrm{pL}(\mathrm{p} < 1)\) in the denser liquid. The density \(d\) is equal to.
361014 The volume of the hollow portion of a sphere is \(\dfrac{3}{4}\) of the external volume of the sphere. If it floats in a liquid of relative density \(\dfrac{3}{2}\), half of its external volume immersed, the relative density of the material of the solid is:
361017
The weight indicated on a balance is \(X\) when a beaker of water is placed on it. A solid object has weight \(Y\) in air and displaces weight \(Z\) of water when completely immersed.The given diagram shows the object suspended from a light string and completely immersed in the beaker of water. what is the balance reading in the given arrangement ?
361018 Two non-mixing liquid of densities \(\rho\) and \(n \rho(\mathrm{n}>1)\) are put in a container. The height of each liquid is \(h\). A solid cylinder of length \(L\) and density \(d\) is put in this container. The cylinder floats with its axis vertical and length \(\mathrm{pL}(\mathrm{p} < 1)\) in the denser liquid. The density \(d\) is equal to.
361014 The volume of the hollow portion of a sphere is \(\dfrac{3}{4}\) of the external volume of the sphere. If it floats in a liquid of relative density \(\dfrac{3}{2}\), half of its external volume immersed, the relative density of the material of the solid is:
361017
The weight indicated on a balance is \(X\) when a beaker of water is placed on it. A solid object has weight \(Y\) in air and displaces weight \(Z\) of water when completely immersed.The given diagram shows the object suspended from a light string and completely immersed in the beaker of water. what is the balance reading in the given arrangement ?
361018 Two non-mixing liquid of densities \(\rho\) and \(n \rho(\mathrm{n}>1)\) are put in a container. The height of each liquid is \(h\). A solid cylinder of length \(L\) and density \(d\) is put in this container. The cylinder floats with its axis vertical and length \(\mathrm{pL}(\mathrm{p} < 1)\) in the denser liquid. The density \(d\) is equal to.
361014 The volume of the hollow portion of a sphere is \(\dfrac{3}{4}\) of the external volume of the sphere. If it floats in a liquid of relative density \(\dfrac{3}{2}\), half of its external volume immersed, the relative density of the material of the solid is:
361017
The weight indicated on a balance is \(X\) when a beaker of water is placed on it. A solid object has weight \(Y\) in air and displaces weight \(Z\) of water when completely immersed.The given diagram shows the object suspended from a light string and completely immersed in the beaker of water. what is the balance reading in the given arrangement ?
361018 Two non-mixing liquid of densities \(\rho\) and \(n \rho(\mathrm{n}>1)\) are put in a container. The height of each liquid is \(h\). A solid cylinder of length \(L\) and density \(d\) is put in this container. The cylinder floats with its axis vertical and length \(\mathrm{pL}(\mathrm{p} < 1)\) in the denser liquid. The density \(d\) is equal to.
361014 The volume of the hollow portion of a sphere is \(\dfrac{3}{4}\) of the external volume of the sphere. If it floats in a liquid of relative density \(\dfrac{3}{2}\), half of its external volume immersed, the relative density of the material of the solid is:
361017
The weight indicated on a balance is \(X\) when a beaker of water is placed on it. A solid object has weight \(Y\) in air and displaces weight \(Z\) of water when completely immersed.The given diagram shows the object suspended from a light string and completely immersed in the beaker of water. what is the balance reading in the given arrangement ?
361018 Two non-mixing liquid of densities \(\rho\) and \(n \rho(\mathrm{n}>1)\) are put in a container. The height of each liquid is \(h\). A solid cylinder of length \(L\) and density \(d\) is put in this container. The cylinder floats with its axis vertical and length \(\mathrm{pL}(\mathrm{p} < 1)\) in the denser liquid. The density \(d\) is equal to.