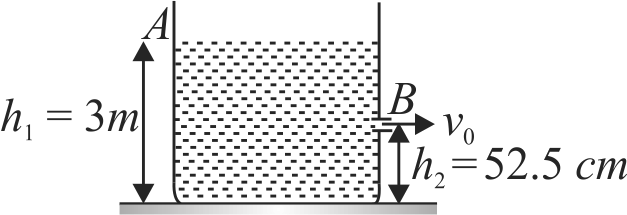
360882 Water is filled in a tank to a height of \(3\,m\). An orifice is made at a height \(52.5\,cm\) from bottom of tank. The ratio of the cross - sectional area of the orifice and the tank is 0.1 . The square of the speed of the liquid coming out from the orifice is (take, \(g = 10\;m{s^{ - 2}}\) )
360884 A large open tank containing water has two holes to its wall. A square hole of side a is made at a depth \(y\) and a circular hole of radius \(r\) is made at a depth \(16y\) from the surface of water. If equal amount of water comes out through both the holes per second, then the relation between \(r\) and \(a\) will be
360882 Water is filled in a tank to a height of \(3\,m\). An orifice is made at a height \(52.5\,cm\) from bottom of tank. The ratio of the cross - sectional area of the orifice and the tank is 0.1 . The square of the speed of the liquid coming out from the orifice is (take, \(g = 10\;m{s^{ - 2}}\) )
360884 A large open tank containing water has two holes to its wall. A square hole of side a is made at a depth \(y\) and a circular hole of radius \(r\) is made at a depth \(16y\) from the surface of water. If equal amount of water comes out through both the holes per second, then the relation between \(r\) and \(a\) will be
360882 Water is filled in a tank to a height of \(3\,m\). An orifice is made at a height \(52.5\,cm\) from bottom of tank. The ratio of the cross - sectional area of the orifice and the tank is 0.1 . The square of the speed of the liquid coming out from the orifice is (take, \(g = 10\;m{s^{ - 2}}\) )
360884 A large open tank containing water has two holes to its wall. A square hole of side a is made at a depth \(y\) and a circular hole of radius \(r\) is made at a depth \(16y\) from the surface of water. If equal amount of water comes out through both the holes per second, then the relation between \(r\) and \(a\) will be
360882 Water is filled in a tank to a height of \(3\,m\). An orifice is made at a height \(52.5\,cm\) from bottom of tank. The ratio of the cross - sectional area of the orifice and the tank is 0.1 . The square of the speed of the liquid coming out from the orifice is (take, \(g = 10\;m{s^{ - 2}}\) )
360884 A large open tank containing water has two holes to its wall. A square hole of side a is made at a depth \(y\) and a circular hole of radius \(r\) is made at a depth \(16y\) from the surface of water. If equal amount of water comes out through both the holes per second, then the relation between \(r\) and \(a\) will be