360817
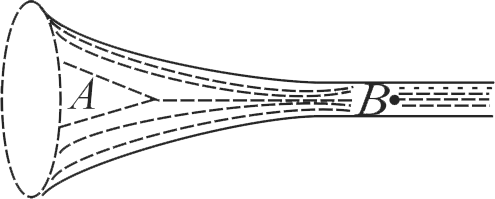
The figure shows a liquid of given density flowing steadily in horizontal tube of varying cross- section. Cross sectional areas at \(A\) is \(1.5\;c{m^2}\), and \(B\) is \(25\;m{m^2}\), if the speed of liquid at \(B\) is \(60\;cm/s\) then \(\left( {{P_A} - {P_B}} \right)\) is (Given: \({{P_A}}\) and \({{P_B}}\) are liquid pressures at \(A\) and \(B\) points. Density \(\rho = 1000\;kg\;{m^{ - 3}},A\) and \(B\) are on the axis of tube)
360817

The figure shows a liquid of given density flowing steadily in horizontal tube of varying cross- section. Cross sectional areas at \(A\) is \(1.5\;c{m^2}\), and \(B\) is \(25\;m{m^2}\), if the speed of liquid at \(B\) is \(60\;cm/s\) then \(\left( {{P_A} - {P_B}} \right)\) is (Given: \({{P_A}}\) and \({{P_B}}\) are liquid pressures at \(A\) and \(B\) points. Density \(\rho = 1000\;kg\;{m^{ - 3}},A\) and \(B\) are on the axis of tube)
360817

The figure shows a liquid of given density flowing steadily in horizontal tube of varying cross- section. Cross sectional areas at \(A\) is \(1.5\;c{m^2}\), and \(B\) is \(25\;m{m^2}\), if the speed of liquid at \(B\) is \(60\;cm/s\) then \(\left( {{P_A} - {P_B}} \right)\) is (Given: \({{P_A}}\) and \({{P_B}}\) are liquid pressures at \(A\) and \(B\) points. Density \(\rho = 1000\;kg\;{m^{ - 3}},A\) and \(B\) are on the axis of tube)
360817

The figure shows a liquid of given density flowing steadily in horizontal tube of varying cross- section. Cross sectional areas at \(A\) is \(1.5\;c{m^2}\), and \(B\) is \(25\;m{m^2}\), if the speed of liquid at \(B\) is \(60\;cm/s\) then \(\left( {{P_A} - {P_B}} \right)\) is (Given: \({{P_A}}\) and \({{P_B}}\) are liquid pressures at \(A\) and \(B\) points. Density \(\rho = 1000\;kg\;{m^{ - 3}},A\) and \(B\) are on the axis of tube)
