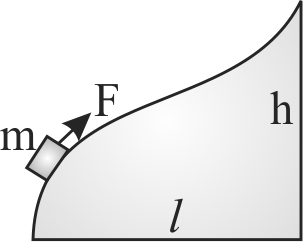
355843 A body of mass \(m\) starts moving from rest along \(x\)-axis so that its velocity varies as \(v=a \sqrt{s}\) where \(a\) is a constant and \(\mathrm{s}\) is the distance covered by the body. The total work done by all the forces acting on the body in the first \(t\) seconds after the start of the motion is:
355844 A proton is kept at rest. A positively charged particle is released from rest at a distance \(d\) in its field. Consider two experiments; one in which the charged particle is also a proton and in another, a positron. In the same time \(t\), the work done on the two moving charged particles is
355846
The work done on a particle of mass \(m\) by a force, \(K\left[\dfrac{x}{\left(x^{2}+y^{2}\right)^{3 / 2}} \hat{i}+\dfrac{y}{\left(x^{2}+y^{2}\right)^{3 / 2}} \hat{j}\right]\). being a constant of appropriate dimensions),
when the particle is taken from the point \((a, 0)\) to the point \((0, a)\) along a circular path of radius \(a\) about the origin in the \(x-y\) plane is
355843 A body of mass \(m\) starts moving from rest along \(x\)-axis so that its velocity varies as \(v=a \sqrt{s}\) where \(a\) is a constant and \(\mathrm{s}\) is the distance covered by the body. The total work done by all the forces acting on the body in the first \(t\) seconds after the start of the motion is:
355844 A proton is kept at rest. A positively charged particle is released from rest at a distance \(d\) in its field. Consider two experiments; one in which the charged particle is also a proton and in another, a positron. In the same time \(t\), the work done on the two moving charged particles is
355846
The work done on a particle of mass \(m\) by a force, \(K\left[\dfrac{x}{\left(x^{2}+y^{2}\right)^{3 / 2}} \hat{i}+\dfrac{y}{\left(x^{2}+y^{2}\right)^{3 / 2}} \hat{j}\right]\). being a constant of appropriate dimensions),
when the particle is taken from the point \((a, 0)\) to the point \((0, a)\) along a circular path of radius \(a\) about the origin in the \(x-y\) plane is
355843 A body of mass \(m\) starts moving from rest along \(x\)-axis so that its velocity varies as \(v=a \sqrt{s}\) where \(a\) is a constant and \(\mathrm{s}\) is the distance covered by the body. The total work done by all the forces acting on the body in the first \(t\) seconds after the start of the motion is:
355844 A proton is kept at rest. A positively charged particle is released from rest at a distance \(d\) in its field. Consider two experiments; one in which the charged particle is also a proton and in another, a positron. In the same time \(t\), the work done on the two moving charged particles is
355846
The work done on a particle of mass \(m\) by a force, \(K\left[\dfrac{x}{\left(x^{2}+y^{2}\right)^{3 / 2}} \hat{i}+\dfrac{y}{\left(x^{2}+y^{2}\right)^{3 / 2}} \hat{j}\right]\). being a constant of appropriate dimensions),
when the particle is taken from the point \((a, 0)\) to the point \((0, a)\) along a circular path of radius \(a\) about the origin in the \(x-y\) plane is
355843 A body of mass \(m\) starts moving from rest along \(x\)-axis so that its velocity varies as \(v=a \sqrt{s}\) where \(a\) is a constant and \(\mathrm{s}\) is the distance covered by the body. The total work done by all the forces acting on the body in the first \(t\) seconds after the start of the motion is:
355844 A proton is kept at rest. A positively charged particle is released from rest at a distance \(d\) in its field. Consider two experiments; one in which the charged particle is also a proton and in another, a positron. In the same time \(t\), the work done on the two moving charged particles is
355846
The work done on a particle of mass \(m\) by a force, \(K\left[\dfrac{x}{\left(x^{2}+y^{2}\right)^{3 / 2}} \hat{i}+\dfrac{y}{\left(x^{2}+y^{2}\right)^{3 / 2}} \hat{j}\right]\). being a constant of appropriate dimensions),
when the particle is taken from the point \((a, 0)\) to the point \((0, a)\) along a circular path of radius \(a\) about the origin in the \(x-y\) plane is