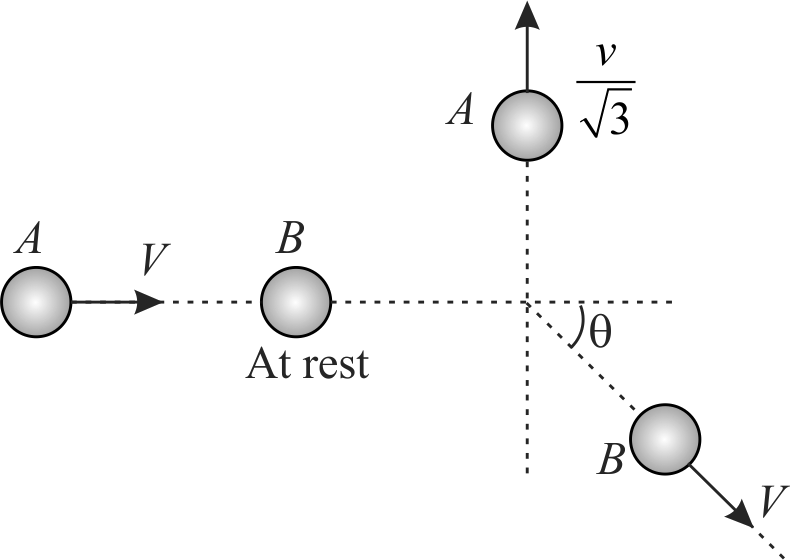
355333 A mass ' \(m\) ' moves with a velocity \(v\) and collides inelastically with another identical mass. After collision the \({1^{st}}\) mass moves with velocity \(\dfrac{v}{\sqrt{3}}\) in a direction perpendicular to the intial direction of motion. Find the speed of the \({2^{nd}}\) mass after collision.
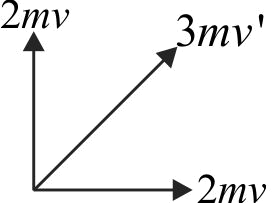
355334 A particle of mass \(m\) moving in the \(x\) direction with speed \(2 v\) is hit by another particle of mass 2 \(m\) moving in the \(y\) direction with speed \(v\). If the collision is perfectly inelastic, the percentage loss in the energy during the collision is close to
355333 A mass ' \(m\) ' moves with a velocity \(v\) and collides inelastically with another identical mass. After collision the \({1^{st}}\) mass moves with velocity \(\dfrac{v}{\sqrt{3}}\) in a direction perpendicular to the intial direction of motion. Find the speed of the \({2^{nd}}\) mass after collision.
355334 A particle of mass \(m\) moving in the \(x\) direction with speed \(2 v\) is hit by another particle of mass 2 \(m\) moving in the \(y\) direction with speed \(v\). If the collision is perfectly inelastic, the percentage loss in the energy during the collision is close to
355333 A mass ' \(m\) ' moves with a velocity \(v\) and collides inelastically with another identical mass. After collision the \({1^{st}}\) mass moves with velocity \(\dfrac{v}{\sqrt{3}}\) in a direction perpendicular to the intial direction of motion. Find the speed of the \({2^{nd}}\) mass after collision.
355334 A particle of mass \(m\) moving in the \(x\) direction with speed \(2 v\) is hit by another particle of mass 2 \(m\) moving in the \(y\) direction with speed \(v\). If the collision is perfectly inelastic, the percentage loss in the energy during the collision is close to
355333 A mass ' \(m\) ' moves with a velocity \(v\) and collides inelastically with another identical mass. After collision the \({1^{st}}\) mass moves with velocity \(\dfrac{v}{\sqrt{3}}\) in a direction perpendicular to the intial direction of motion. Find the speed of the \({2^{nd}}\) mass after collision.
355334 A particle of mass \(m\) moving in the \(x\) direction with speed \(2 v\) is hit by another particle of mass 2 \(m\) moving in the \(y\) direction with speed \(v\). If the collision is perfectly inelastic, the percentage loss in the energy during the collision is close to