355305
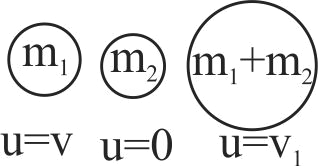
A block of mass \(m\) rests on a rough horizontal surface (Coefficient of friction is \(\mu\) ). When a bullet of mass \(m\)/2 strikes horizontally, and get embedded in it, the block moves a distance d before coming to rest. The initial velocity of the bullet is \(k \sqrt{2 \mu g d}\), then the value of \(k\) is
355305
A block of mass \(m\) rests on a rough horizontal surface (Coefficient of friction is \(\mu\) ). When a bullet of mass \(m\)/2 strikes horizontally, and get embedded in it, the block moves a distance d before coming to rest. The initial velocity of the bullet is \(k \sqrt{2 \mu g d}\), then the value of \(k\) is
355305
A block of mass \(m\) rests on a rough horizontal surface (Coefficient of friction is \(\mu\) ). When a bullet of mass \(m\)/2 strikes horizontally, and get embedded in it, the block moves a distance d before coming to rest. The initial velocity of the bullet is \(k \sqrt{2 \mu g d}\), then the value of \(k\) is
355305
A block of mass \(m\) rests on a rough horizontal surface (Coefficient of friction is \(\mu\) ). When a bullet of mass \(m\)/2 strikes horizontally, and get embedded in it, the block moves a distance d before coming to rest. The initial velocity of the bullet is \(k \sqrt{2 \mu g d}\), then the value of \(k\) is