355205 Two particles masses \(m_{1}\) and \(m_{2}\) in projectile motion have velocities \(\vec{v}_{1}\) and \(\vec{v}_{2}\) respectively at time \(t=0\). They collide at time \(t_{0}\). Their velocities become \(\vec{v}_{1}^{\prime}\) and \(\vec{v}_{2}^{\prime}\) at time \(2 t_{0}\) while still moving in air. The value of \(\mid\left(m_{1} \vec{v}_{1}^{\prime}+m_{2} \vec{v}_{2}^{\prime}-\left(m_{1} \vec{v}_{1}+m_{2} \vec{v}_{2}\right) \mid\right.\) is
355207
Two astronauts, \(A\) and \(B\), both with mass of \(60\;kg\), are moving along a straight line in the same direction in a "weightless" spaceship. Relative to the spaceship the speed of \(A\) is \(2\;m{\rm{/}}s\) and that of \(B\) is \(1\;m{\rm{/}}s\). A is carrying a bag of mass \(5\;kg\) with him. To avoid collision with \(B, A\) throws the bag with a speed \(v\) relative to the spaceship towards \(B\) and \(B\) catches it. Find the minimum value of \(v\).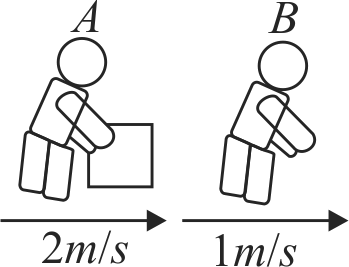
355208
For a system to follow the law of conservation of linear momentum during a collision, the condition is
(i) total external force acting on the system is zero.
(ii) total external force acting on the system is finite and time of collision is negligible.
(iii) total internal force acting on the system is zero.
355205 Two particles masses \(m_{1}\) and \(m_{2}\) in projectile motion have velocities \(\vec{v}_{1}\) and \(\vec{v}_{2}\) respectively at time \(t=0\). They collide at time \(t_{0}\). Their velocities become \(\vec{v}_{1}^{\prime}\) and \(\vec{v}_{2}^{\prime}\) at time \(2 t_{0}\) while still moving in air. The value of \(\mid\left(m_{1} \vec{v}_{1}^{\prime}+m_{2} \vec{v}_{2}^{\prime}-\left(m_{1} \vec{v}_{1}+m_{2} \vec{v}_{2}\right) \mid\right.\) is
355207
Two astronauts, \(A\) and \(B\), both with mass of \(60\;kg\), are moving along a straight line in the same direction in a "weightless" spaceship. Relative to the spaceship the speed of \(A\) is \(2\;m{\rm{/}}s\) and that of \(B\) is \(1\;m{\rm{/}}s\). A is carrying a bag of mass \(5\;kg\) with him. To avoid collision with \(B, A\) throws the bag with a speed \(v\) relative to the spaceship towards \(B\) and \(B\) catches it. Find the minimum value of \(v\).
355208
For a system to follow the law of conservation of linear momentum during a collision, the condition is
(i) total external force acting on the system is zero.
(ii) total external force acting on the system is finite and time of collision is negligible.
(iii) total internal force acting on the system is zero.
355205 Two particles masses \(m_{1}\) and \(m_{2}\) in projectile motion have velocities \(\vec{v}_{1}\) and \(\vec{v}_{2}\) respectively at time \(t=0\). They collide at time \(t_{0}\). Their velocities become \(\vec{v}_{1}^{\prime}\) and \(\vec{v}_{2}^{\prime}\) at time \(2 t_{0}\) while still moving in air. The value of \(\mid\left(m_{1} \vec{v}_{1}^{\prime}+m_{2} \vec{v}_{2}^{\prime}-\left(m_{1} \vec{v}_{1}+m_{2} \vec{v}_{2}\right) \mid\right.\) is
355207
Two astronauts, \(A\) and \(B\), both with mass of \(60\;kg\), are moving along a straight line in the same direction in a "weightless" spaceship. Relative to the spaceship the speed of \(A\) is \(2\;m{\rm{/}}s\) and that of \(B\) is \(1\;m{\rm{/}}s\). A is carrying a bag of mass \(5\;kg\) with him. To avoid collision with \(B, A\) throws the bag with a speed \(v\) relative to the spaceship towards \(B\) and \(B\) catches it. Find the minimum value of \(v\).
355208
For a system to follow the law of conservation of linear momentum during a collision, the condition is
(i) total external force acting on the system is zero.
(ii) total external force acting on the system is finite and time of collision is negligible.
(iii) total internal force acting on the system is zero.
355205 Two particles masses \(m_{1}\) and \(m_{2}\) in projectile motion have velocities \(\vec{v}_{1}\) and \(\vec{v}_{2}\) respectively at time \(t=0\). They collide at time \(t_{0}\). Their velocities become \(\vec{v}_{1}^{\prime}\) and \(\vec{v}_{2}^{\prime}\) at time \(2 t_{0}\) while still moving in air. The value of \(\mid\left(m_{1} \vec{v}_{1}^{\prime}+m_{2} \vec{v}_{2}^{\prime}-\left(m_{1} \vec{v}_{1}+m_{2} \vec{v}_{2}\right) \mid\right.\) is
355207
Two astronauts, \(A\) and \(B\), both with mass of \(60\;kg\), are moving along a straight line in the same direction in a "weightless" spaceship. Relative to the spaceship the speed of \(A\) is \(2\;m{\rm{/}}s\) and that of \(B\) is \(1\;m{\rm{/}}s\). A is carrying a bag of mass \(5\;kg\) with him. To avoid collision with \(B, A\) throws the bag with a speed \(v\) relative to the spaceship towards \(B\) and \(B\) catches it. Find the minimum value of \(v\).
355208
For a system to follow the law of conservation of linear momentum during a collision, the condition is
(i) total external force acting on the system is zero.
(ii) total external force acting on the system is finite and time of collision is negligible.
(iii) total internal force acting on the system is zero.
355205 Two particles masses \(m_{1}\) and \(m_{2}\) in projectile motion have velocities \(\vec{v}_{1}\) and \(\vec{v}_{2}\) respectively at time \(t=0\). They collide at time \(t_{0}\). Their velocities become \(\vec{v}_{1}^{\prime}\) and \(\vec{v}_{2}^{\prime}\) at time \(2 t_{0}\) while still moving in air. The value of \(\mid\left(m_{1} \vec{v}_{1}^{\prime}+m_{2} \vec{v}_{2}^{\prime}-\left(m_{1} \vec{v}_{1}+m_{2} \vec{v}_{2}\right) \mid\right.\) is
355207
Two astronauts, \(A\) and \(B\), both with mass of \(60\;kg\), are moving along a straight line in the same direction in a "weightless" spaceship. Relative to the spaceship the speed of \(A\) is \(2\;m{\rm{/}}s\) and that of \(B\) is \(1\;m{\rm{/}}s\). A is carrying a bag of mass \(5\;kg\) with him. To avoid collision with \(B, A\) throws the bag with a speed \(v\) relative to the spaceship towards \(B\) and \(B\) catches it. Find the minimum value of \(v\).
355208
For a system to follow the law of conservation of linear momentum during a collision, the condition is
(i) total external force acting on the system is zero.
(ii) total external force acting on the system is finite and time of collision is negligible.
(iii) total internal force acting on the system is zero.
