355187
A guitar string of length \(L\) is stretched between two fixed points \(P\) and \(Q\) and made to vibrate transversely as shown in the figure. Two particles \(A\) and \(B\) on the string are separated by a distance \(s\). The maximum kinetic energies of \(A\) and \(B\) are \(K_{A}\) and \(K_{B}\) respectively. Which of the following gives the correct phase difference and maximum kinetic energies of the particles?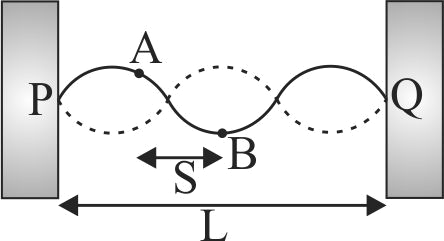
\[\begin{array}{*{20}{c}}
{{\text{phase}}\,{\text{difference}}}&{{\text{Maximum}}\,{\text{kinetic}}\,{\text{energy}}}
\end{array}\]
355187
A guitar string of length \(L\) is stretched between two fixed points \(P\) and \(Q\) and made to vibrate transversely as shown in the figure. Two particles \(A\) and \(B\) on the string are separated by a distance \(s\). The maximum kinetic energies of \(A\) and \(B\) are \(K_{A}\) and \(K_{B}\) respectively. Which of the following gives the correct phase difference and maximum kinetic energies of the particles?
\[\begin{array}{*{20}{c}}
{{\text{phase}}\,{\text{difference}}}&{{\text{Maximum}}\,{\text{kinetic}}\,{\text{energy}}}
\end{array}\]
355187
A guitar string of length \(L\) is stretched between two fixed points \(P\) and \(Q\) and made to vibrate transversely as shown in the figure. Two particles \(A\) and \(B\) on the string are separated by a distance \(s\). The maximum kinetic energies of \(A\) and \(B\) are \(K_{A}\) and \(K_{B}\) respectively. Which of the following gives the correct phase difference and maximum kinetic energies of the particles?
\[\begin{array}{*{20}{c}}
{{\text{phase}}\,{\text{difference}}}&{{\text{Maximum}}\,{\text{kinetic}}\,{\text{energy}}}
\end{array}\]
355187
A guitar string of length \(L\) is stretched between two fixed points \(P\) and \(Q\) and made to vibrate transversely as shown in the figure. Two particles \(A\) and \(B\) on the string are separated by a distance \(s\). The maximum kinetic energies of \(A\) and \(B\) are \(K_{A}\) and \(K_{B}\) respectively. Which of the following gives the correct phase difference and maximum kinetic energies of the particles?
\[\begin{array}{*{20}{c}}
{{\text{phase}}\,{\text{difference}}}&{{\text{Maximum}}\,{\text{kinetic}}\,{\text{energy}}}
\end{array}\]
