355117 Fundamental frequency of sonometer wire is \(n\). If the length, tension and diameter of wire are tripled, the new fundamental frequency is

355118 A surface of area \(S\) is placed perpendicular to the direction of travel of a plane wave. The energy per unit time intercepted by the surface is \(E\) when the amplitude of the wave is \(A\). The area of the surface is reduced to \(1/2\;S\) and the amplitude of the wave is increased to \(2\;A\). What is the energy per unit time intercepted by this smaller surface?

355119 A standing wave having 3 nodes and 2 antinodes is formed between two atoms having a distance \(1.21\mathop A\limits^o \) between them. The wavelength of the standing wave is :

355120 What could be a correct expression for the speed of ocean waves in terms of its wavelength \(\lambda\), the depth \(h\) of the ocean, the density \(\rho\) of sea water, and the acceleration of free fall \(g\) ?

355121 The \((x, y)\) coordinates of the corners of a squareplate are \(\left( {0,0} \right)\), \(\left( {L,0} \right)\), \(\left( {L,L} \right)\), and \(\left( {0,L} \right)\). The edges of the plates are clamped and transverse standing waves are set - up in it. If \(u\left( {x,y} \right)\) denotes the displacement of the plate at the point \(\left( {x,y} \right)\) at some instant of time, the possible expression for \(u\) is \(\left( {u\,{\text{ = }}\,{\text{positive}}\,{\text{constant}}} \right)\)

355117 Fundamental frequency of sonometer wire is \(n\). If the length, tension and diameter of wire are tripled, the new fundamental frequency is

355118 A surface of area \(S\) is placed perpendicular to the direction of travel of a plane wave. The energy per unit time intercepted by the surface is \(E\) when the amplitude of the wave is \(A\). The area of the surface is reduced to \(1/2\;S\) and the amplitude of the wave is increased to \(2\;A\). What is the energy per unit time intercepted by this smaller surface?

355119 A standing wave having 3 nodes and 2 antinodes is formed between two atoms having a distance \(1.21\mathop A\limits^o \) between them. The wavelength of the standing wave is :

355120 What could be a correct expression for the speed of ocean waves in terms of its wavelength \(\lambda\), the depth \(h\) of the ocean, the density \(\rho\) of sea water, and the acceleration of free fall \(g\) ?

355121 The \((x, y)\) coordinates of the corners of a squareplate are \(\left( {0,0} \right)\), \(\left( {L,0} \right)\), \(\left( {L,L} \right)\), and \(\left( {0,L} \right)\). The edges of the plates are clamped and transverse standing waves are set - up in it. If \(u\left( {x,y} \right)\) denotes the displacement of the plate at the point \(\left( {x,y} \right)\) at some instant of time, the possible expression for \(u\) is \(\left( {u\,{\text{ = }}\,{\text{positive}}\,{\text{constant}}} \right)\)

355117 Fundamental frequency of sonometer wire is \(n\). If the length, tension and diameter of wire are tripled, the new fundamental frequency is

355118 A surface of area \(S\) is placed perpendicular to the direction of travel of a plane wave. The energy per unit time intercepted by the surface is \(E\) when the amplitude of the wave is \(A\). The area of the surface is reduced to \(1/2\;S\) and the amplitude of the wave is increased to \(2\;A\). What is the energy per unit time intercepted by this smaller surface?

355119 A standing wave having 3 nodes and 2 antinodes is formed between two atoms having a distance \(1.21\mathop A\limits^o \) between them. The wavelength of the standing wave is :

355120 What could be a correct expression for the speed of ocean waves in terms of its wavelength \(\lambda\), the depth \(h\) of the ocean, the density \(\rho\) of sea water, and the acceleration of free fall \(g\) ?

355121 The \((x, y)\) coordinates of the corners of a squareplate are \(\left( {0,0} \right)\), \(\left( {L,0} \right)\), \(\left( {L,L} \right)\), and \(\left( {0,L} \right)\). The edges of the plates are clamped and transverse standing waves are set - up in it. If \(u\left( {x,y} \right)\) denotes the displacement of the plate at the point \(\left( {x,y} \right)\) at some instant of time, the possible expression for \(u\) is \(\left( {u\,{\text{ = }}\,{\text{positive}}\,{\text{constant}}} \right)\)

355117 Fundamental frequency of sonometer wire is \(n\). If the length, tension and diameter of wire are tripled, the new fundamental frequency is

355118 A surface of area \(S\) is placed perpendicular to the direction of travel of a plane wave. The energy per unit time intercepted by the surface is \(E\) when the amplitude of the wave is \(A\). The area of the surface is reduced to \(1/2\;S\) and the amplitude of the wave is increased to \(2\;A\). What is the energy per unit time intercepted by this smaller surface?

355119 A standing wave having 3 nodes and 2 antinodes is formed between two atoms having a distance \(1.21\mathop A\limits^o \) between them. The wavelength of the standing wave is :

355120 What could be a correct expression for the speed of ocean waves in terms of its wavelength \(\lambda\), the depth \(h\) of the ocean, the density \(\rho\) of sea water, and the acceleration of free fall \(g\) ?

355121 The \((x, y)\) coordinates of the corners of a squareplate are \(\left( {0,0} \right)\), \(\left( {L,0} \right)\), \(\left( {L,L} \right)\), and \(\left( {0,L} \right)\). The edges of the plates are clamped and transverse standing waves are set - up in it. If \(u\left( {x,y} \right)\) denotes the displacement of the plate at the point \(\left( {x,y} \right)\) at some instant of time, the possible expression for \(u\) is \(\left( {u\,{\text{ = }}\,{\text{positive}}\,{\text{constant}}} \right)\)

355117 Fundamental frequency of sonometer wire is \(n\). If the length, tension and diameter of wire are tripled, the new fundamental frequency is

355118 A surface of area \(S\) is placed perpendicular to the direction of travel of a plane wave. The energy per unit time intercepted by the surface is \(E\) when the amplitude of the wave is \(A\). The area of the surface is reduced to \(1/2\;S\) and the amplitude of the wave is increased to \(2\;A\). What is the energy per unit time intercepted by this smaller surface?

355119 A standing wave having 3 nodes and 2 antinodes is formed between two atoms having a distance \(1.21\mathop A\limits^o \) between them. The wavelength of the standing wave is :

355120 What could be a correct expression for the speed of ocean waves in terms of its wavelength \(\lambda\), the depth \(h\) of the ocean, the density \(\rho\) of sea water, and the acceleration of free fall \(g\) ?

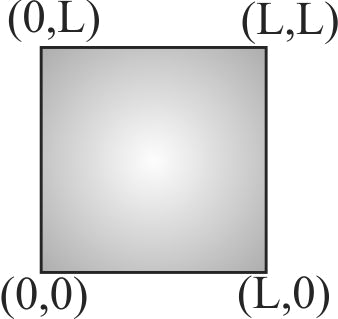
355121 The \((x, y)\) coordinates of the corners of a squareplate are \(\left( {0,0} \right)\), \(\left( {L,0} \right)\), \(\left( {L,L} \right)\), and \(\left( {0,L} \right)\). The edges of the plates are clamped and transverse standing waves are set - up in it. If \(u\left( {x,y} \right)\) denotes the displacement of the plate at the point \(\left( {x,y} \right)\) at some instant of time, the possible expression for \(u\) is \(\left( {u\,{\text{ = }}\,{\text{positive}}\,{\text{constant}}} \right)\)