Explanation:
Let us make the following table
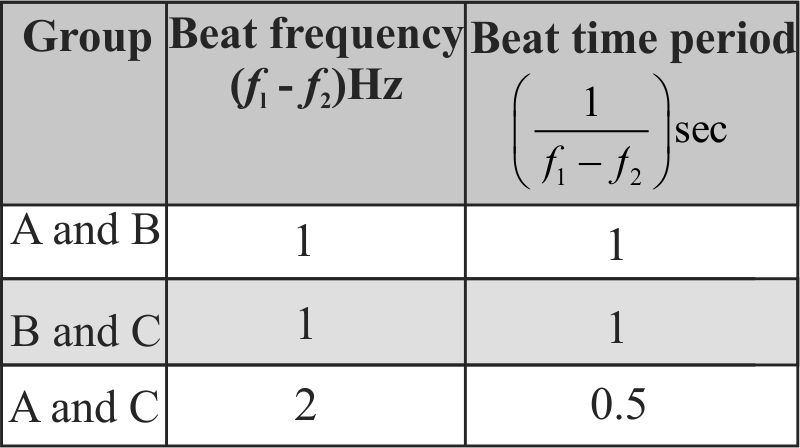
Beat time period for \(A\) and \(B\) is \(1\,s\). It implies that if \(A\) and \(B\) are in phase at time \(t=0\), they are again in phase after \(1\,s\). Same is the case with \(B\) and \(C\). But beat time period for\(A\) and \(C\) is \(0.5\;s\).
Therefore, beat time period for all together \(A\), \(B\) and \(C\) will be \(1\,s\). Because if, at \(t = 0,\;A,\;B\,{\text{and}}\,C\) all are in phase then after \(1\,s\). (\(A\) and \(B\)) and (\(B\) and \(C\) ) will again be in phase for the first time while (\(A\) and \(C\)) will be in phase for the second time. Or we can say all \(A\), \(B\) and \(C\) are again in phase after \(1\,s\).
\(\therefore \quad\) Beat time period \(T_{b}=1 s\)
or \(\quad\)
Beat frequency \({f_b} = \frac{1}{{{T_b}}} = 1\;Hz\)