354863 A flexible steel cable of total length ' \(L\) ' and mass per unit length \(\mu\) hangs vertically (under it's own weight) from a support at upper end. If a small transverse pulse starts to move down in the wire from its support. The ratio of acceleration of the pulse at distance \(\dfrac{L}{4}\) from the support end to the acceleration of the of the pulse at distance \(\dfrac{L}{2}\) from the support is
354865
A long, heavy, flexible rope with mass per unit length \(\mu\) is under a constant tension \(T\). A sudden movement causes a circular loop, of radius \(R\) to form at one end of the rope. The loop rolls along the rope with speed \(v\) as shown in the figure. Find the kinetic energy (in \(J\)) of this circular pulse. \(\left[ {\mu = 5 \times {{10}^{ - 2}}kg{m^{ - 1}},R = \frac{1}{{8\pi }}m,T = 16\;N} \right]\)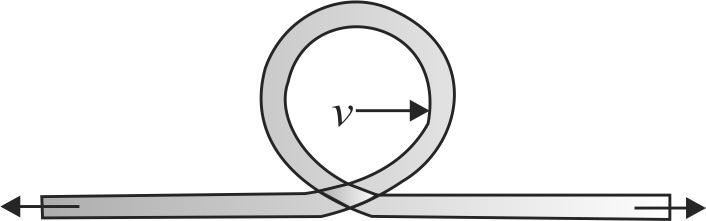
354863 A flexible steel cable of total length ' \(L\) ' and mass per unit length \(\mu\) hangs vertically (under it's own weight) from a support at upper end. If a small transverse pulse starts to move down in the wire from its support. The ratio of acceleration of the pulse at distance \(\dfrac{L}{4}\) from the support end to the acceleration of the of the pulse at distance \(\dfrac{L}{2}\) from the support is
354865
A long, heavy, flexible rope with mass per unit length \(\mu\) is under a constant tension \(T\). A sudden movement causes a circular loop, of radius \(R\) to form at one end of the rope. The loop rolls along the rope with speed \(v\) as shown in the figure. Find the kinetic energy (in \(J\)) of this circular pulse. \(\left[ {\mu = 5 \times {{10}^{ - 2}}kg{m^{ - 1}},R = \frac{1}{{8\pi }}m,T = 16\;N} \right]\)
354863 A flexible steel cable of total length ' \(L\) ' and mass per unit length \(\mu\) hangs vertically (under it's own weight) from a support at upper end. If a small transverse pulse starts to move down in the wire from its support. The ratio of acceleration of the pulse at distance \(\dfrac{L}{4}\) from the support end to the acceleration of the of the pulse at distance \(\dfrac{L}{2}\) from the support is
354865
A long, heavy, flexible rope with mass per unit length \(\mu\) is under a constant tension \(T\). A sudden movement causes a circular loop, of radius \(R\) to form at one end of the rope. The loop rolls along the rope with speed \(v\) as shown in the figure. Find the kinetic energy (in \(J\)) of this circular pulse. \(\left[ {\mu = 5 \times {{10}^{ - 2}}kg{m^{ - 1}},R = \frac{1}{{8\pi }}m,T = 16\;N} \right]\)
354863 A flexible steel cable of total length ' \(L\) ' and mass per unit length \(\mu\) hangs vertically (under it's own weight) from a support at upper end. If a small transverse pulse starts to move down in the wire from its support. The ratio of acceleration of the pulse at distance \(\dfrac{L}{4}\) from the support end to the acceleration of the of the pulse at distance \(\dfrac{L}{2}\) from the support is
354865
A long, heavy, flexible rope with mass per unit length \(\mu\) is under a constant tension \(T\). A sudden movement causes a circular loop, of radius \(R\) to form at one end of the rope. The loop rolls along the rope with speed \(v\) as shown in the figure. Find the kinetic energy (in \(J\)) of this circular pulse. \(\left[ {\mu = 5 \times {{10}^{ - 2}}kg{m^{ - 1}},R = \frac{1}{{8\pi }}m,T = 16\;N} \right]\)