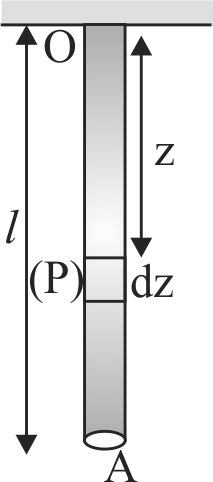
354858 A rope hangs from a rigid support. A pulse is set by jiggling the bottom end. We want to design a rope in which velocity \(v\) of pulse is independent of \(z\), the distance of the pulse from fixed end of the rope. If the rope is very long the desired function for mass per unit length \(\mu (z)\) in terms of \(\mu_{0}\) [mass per unit length of the rope at the top \((z = 0)\)] is given by
354861
A circular loop of string rotates about its axis on a frictionless horizontal plane at a uniform rate so that the tangential speed of any particle of the string is \(v\). If a small transverse disturbance is produced at a point of the loop, speed (relative to the string) of this disturbance travel on the string is \(x v\) then \(x\) is
354858 A rope hangs from a rigid support. A pulse is set by jiggling the bottom end. We want to design a rope in which velocity \(v\) of pulse is independent of \(z\), the distance of the pulse from fixed end of the rope. If the rope is very long the desired function for mass per unit length \(\mu (z)\) in terms of \(\mu_{0}\) [mass per unit length of the rope at the top \((z = 0)\)] is given by
354861
A circular loop of string rotates about its axis on a frictionless horizontal plane at a uniform rate so that the tangential speed of any particle of the string is \(v\). If a small transverse disturbance is produced at a point of the loop, speed (relative to the string) of this disturbance travel on the string is \(x v\) then \(x\) is
354858 A rope hangs from a rigid support. A pulse is set by jiggling the bottom end. We want to design a rope in which velocity \(v\) of pulse is independent of \(z\), the distance of the pulse from fixed end of the rope. If the rope is very long the desired function for mass per unit length \(\mu (z)\) in terms of \(\mu_{0}\) [mass per unit length of the rope at the top \((z = 0)\)] is given by
354861
A circular loop of string rotates about its axis on a frictionless horizontal plane at a uniform rate so that the tangential speed of any particle of the string is \(v\). If a small transverse disturbance is produced at a point of the loop, speed (relative to the string) of this disturbance travel on the string is \(x v\) then \(x\) is
354858 A rope hangs from a rigid support. A pulse is set by jiggling the bottom end. We want to design a rope in which velocity \(v\) of pulse is independent of \(z\), the distance of the pulse from fixed end of the rope. If the rope is very long the desired function for mass per unit length \(\mu (z)\) in terms of \(\mu_{0}\) [mass per unit length of the rope at the top \((z = 0)\)] is given by
354861
A circular loop of string rotates about its axis on a frictionless horizontal plane at a uniform rate so that the tangential speed of any particle of the string is \(v\). If a small transverse disturbance is produced at a point of the loop, speed (relative to the string) of this disturbance travel on the string is \(x v\) then \(x\) is