359942
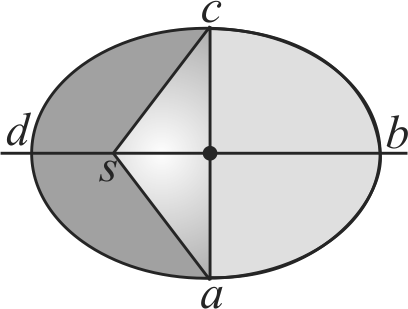
Figure shows elliptical path abcd of a planet around the sun S such that the area of triangle csa is \(\dfrac{1}{4}\) the area of the ellipse . (See figure) With \(db\) as the semi major axis, and \(ca\) as the semi minor axis. If \(t_{1}\) is the time taken for planet to go over path \(abc\) and \(t_{2}\) for path taken over \(cda\) then :
359942
Figure shows elliptical path abcd of a planet around the sun S such that the area of triangle csa is \(\dfrac{1}{4}\) the area of the ellipse . (See figure) With \(db\) as the semi major axis, and \(ca\) as the semi minor axis. If \(t_{1}\) is the time taken for planet to go over path \(abc\) and \(t_{2}\) for path taken over \(cda\) then :
359942
Figure shows elliptical path abcd of a planet around the sun S such that the area of triangle csa is \(\dfrac{1}{4}\) the area of the ellipse . (See figure) With \(db\) as the semi major axis, and \(ca\) as the semi minor axis. If \(t_{1}\) is the time taken for planet to go over path \(abc\) and \(t_{2}\) for path taken over \(cda\) then :
359942
Figure shows elliptical path abcd of a planet around the sun S such that the area of triangle csa is \(\dfrac{1}{4}\) the area of the ellipse . (See figure) With \(db\) as the semi major axis, and \(ca\) as the semi minor axis. If \(t_{1}\) is the time taken for planet to go over path \(abc\) and \(t_{2}\) for path taken over \(cda\) then :
359942
Figure shows elliptical path abcd of a planet around the sun S such that the area of triangle csa is \(\dfrac{1}{4}\) the area of the ellipse . (See figure) With \(db\) as the semi major axis, and \(ca\) as the semi minor axis. If \(t_{1}\) is the time taken for planet to go over path \(abc\) and \(t_{2}\) for path taken over \(cda\) then :