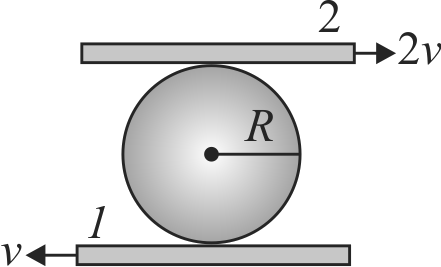
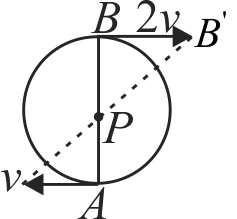
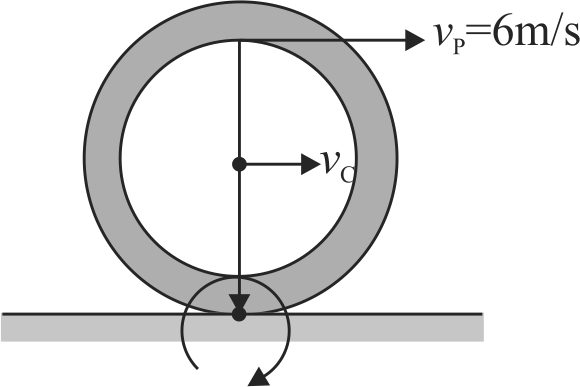
366054 A sphere is rolling without slipping on a fixed horizonal plane surface. In the figure, \(\mathrm{A}\) is the point of contanct, \(\mathrm{B}\) is the center of the sphere and \(\mathrm{C}\) is its topmost point. Then:
(i) \({\overrightarrow V _C} - {\overrightarrow V _A} = 2\left( {{{\overrightarrow V }_B} - {{\overrightarrow V }_C}} \right)\)
(ii) \({\overrightarrow V _C} - {\overrightarrow V _B} = {\overrightarrow V _B} - {\overrightarrow V _A}\)
(iii) \(\left| {{{\overrightarrow V }_C} - {{\overrightarrow V }_A}} \right| = 2\left| {{{\overrightarrow V }_B} - {{\overrightarrow V }_C}} \right|\)
(iv) \(\left| {{{\overrightarrow V }_C} - {{\overrightarrow V }_A}} \right| = 4\left| {{{\overrightarrow V }_B}} \right|\)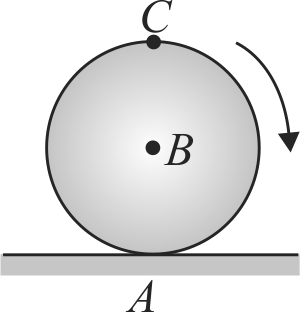

366055 An automobile engine develops \(100\;kW\) when rotating at a speed of \(1800\,\,rev/\min .\) What torque does it deliver?

366056 A cylinder is rolling without sliding over two horizontal planks (surfaces) 1 and 2. If the velocities of the surfaces 1 and 2 are \(-v \hat{i}\) and \(2 v \hat{i}\) respectively, find the instantaneous centre of the cyclinder?

366057 A cotton reel rolls without sliding such that the point \(P\) of the string has velocity \(v = 6\;m/s\). If \(r = 10\;cm\) and \(R = 20\;cm\) then the velocity of its centre \(C\) is

366054 A sphere is rolling without slipping on a fixed horizonal plane surface. In the figure, \(\mathrm{A}\) is the point of contanct, \(\mathrm{B}\) is the center of the sphere and \(\mathrm{C}\) is its topmost point. Then:
(i) \({\overrightarrow V _C} - {\overrightarrow V _A} = 2\left( {{{\overrightarrow V }_B} - {{\overrightarrow V }_C}} \right)\)
(ii) \({\overrightarrow V _C} - {\overrightarrow V _B} = {\overrightarrow V _B} - {\overrightarrow V _A}\)
(iii) \(\left| {{{\overrightarrow V }_C} - {{\overrightarrow V }_A}} \right| = 2\left| {{{\overrightarrow V }_B} - {{\overrightarrow V }_C}} \right|\)
(iv) \(\left| {{{\overrightarrow V }_C} - {{\overrightarrow V }_A}} \right| = 4\left| {{{\overrightarrow V }_B}} \right|\)

366055 An automobile engine develops \(100\;kW\) when rotating at a speed of \(1800\,\,rev/\min .\) What torque does it deliver?

366056 A cylinder is rolling without sliding over two horizontal planks (surfaces) 1 and 2. If the velocities of the surfaces 1 and 2 are \(-v \hat{i}\) and \(2 v \hat{i}\) respectively, find the instantaneous centre of the cyclinder?

366057 A cotton reel rolls without sliding such that the point \(P\) of the string has velocity \(v = 6\;m/s\). If \(r = 10\;cm\) and \(R = 20\;cm\) then the velocity of its centre \(C\) is

366054 A sphere is rolling without slipping on a fixed horizonal plane surface. In the figure, \(\mathrm{A}\) is the point of contanct, \(\mathrm{B}\) is the center of the sphere and \(\mathrm{C}\) is its topmost point. Then:
(i) \({\overrightarrow V _C} - {\overrightarrow V _A} = 2\left( {{{\overrightarrow V }_B} - {{\overrightarrow V }_C}} \right)\)
(ii) \({\overrightarrow V _C} - {\overrightarrow V _B} = {\overrightarrow V _B} - {\overrightarrow V _A}\)
(iii) \(\left| {{{\overrightarrow V }_C} - {{\overrightarrow V }_A}} \right| = 2\left| {{{\overrightarrow V }_B} - {{\overrightarrow V }_C}} \right|\)
(iv) \(\left| {{{\overrightarrow V }_C} - {{\overrightarrow V }_A}} \right| = 4\left| {{{\overrightarrow V }_B}} \right|\)

366055 An automobile engine develops \(100\;kW\) when rotating at a speed of \(1800\,\,rev/\min .\) What torque does it deliver?

366056 A cylinder is rolling without sliding over two horizontal planks (surfaces) 1 and 2. If the velocities of the surfaces 1 and 2 are \(-v \hat{i}\) and \(2 v \hat{i}\) respectively, find the instantaneous centre of the cyclinder?

366057 A cotton reel rolls without sliding such that the point \(P\) of the string has velocity \(v = 6\;m/s\). If \(r = 10\;cm\) and \(R = 20\;cm\) then the velocity of its centre \(C\) is

366054 A sphere is rolling without slipping on a fixed horizonal plane surface. In the figure, \(\mathrm{A}\) is the point of contanct, \(\mathrm{B}\) is the center of the sphere and \(\mathrm{C}\) is its topmost point. Then:
(i) \({\overrightarrow V _C} - {\overrightarrow V _A} = 2\left( {{{\overrightarrow V }_B} - {{\overrightarrow V }_C}} \right)\)
(ii) \({\overrightarrow V _C} - {\overrightarrow V _B} = {\overrightarrow V _B} - {\overrightarrow V _A}\)
(iii) \(\left| {{{\overrightarrow V }_C} - {{\overrightarrow V }_A}} \right| = 2\left| {{{\overrightarrow V }_B} - {{\overrightarrow V }_C}} \right|\)
(iv) \(\left| {{{\overrightarrow V }_C} - {{\overrightarrow V }_A}} \right| = 4\left| {{{\overrightarrow V }_B}} \right|\)

366055 An automobile engine develops \(100\;kW\) when rotating at a speed of \(1800\,\,rev/\min .\) What torque does it deliver?

366056 A cylinder is rolling without sliding over two horizontal planks (surfaces) 1 and 2. If the velocities of the surfaces 1 and 2 are \(-v \hat{i}\) and \(2 v \hat{i}\) respectively, find the instantaneous centre of the cyclinder?

366057 A cotton reel rolls without sliding such that the point \(P\) of the string has velocity \(v = 6\;m/s\). If \(r = 10\;cm\) and \(R = 20\;cm\) then the velocity of its centre \(C\) is