365926 A thin uniform rectangular plate of mass \(2\;kg\) is placed in \(x-y\) plane as shown in figure.
The moment of inertia about \(X\)-axis is \({I_x} = 0.2\,kg\,{m^2}\) and moment of ineria about \(y\)-axis is \({I_x} = 0.3\,kg{\mkern 1mu} {m^2}\). The radius of gyration of the plate about the axis passing through O and per perpendicular to the plane of the plate is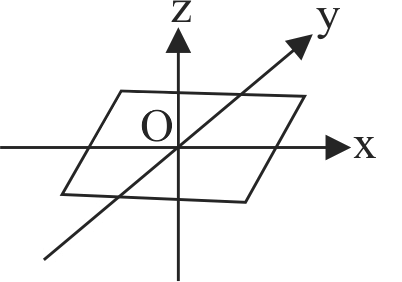

365927 Let \(M\) be the mass and \(L\) be the length of a thin uniform rod. In first case, axis of rotation is passing through centre and perpendicular to the length of the rod. In second case, axis of rotation is passing through one end and perpendicular to the length of the rod. The ratio of radius of gyration in first case to second case is

365928 The moment of inertia of a uniform rod of length \(2 l\) and mass \(m\) about an axis \(x x^{\prime}\) passing through its centre and inclined at an angle \(\alpha\) is

365929 Match the column I (rotation of different bodies) with column II (their moment of inertia) and select the correct answer from the codes given below.
Column I
Column II
A
Circular ring of radius \(R\) having axis perpendicular to the plane and passing through centre
P
\(M{R^2}/2\)
B
Thin circular ring of radius \(R\) having axis passing through its diameter
Q
\(M{L^2}/3\)
C
Thin rod of length \(L\) about an axis passing through end point
R
\(M{R^2}\)
D
Circular disc of radius \(R\) about an axis perpendicular to the disc and touching the edge.
S
\(3M{R^2}/2\)

365926 A thin uniform rectangular plate of mass \(2\;kg\) is placed in \(x-y\) plane as shown in figure.
The moment of inertia about \(X\)-axis is \({I_x} = 0.2\,kg\,{m^2}\) and moment of ineria about \(y\)-axis is \({I_x} = 0.3\,kg{\mkern 1mu} {m^2}\). The radius of gyration of the plate about the axis passing through O and per perpendicular to the plane of the plate is

365927 Let \(M\) be the mass and \(L\) be the length of a thin uniform rod. In first case, axis of rotation is passing through centre and perpendicular to the length of the rod. In second case, axis of rotation is passing through one end and perpendicular to the length of the rod. The ratio of radius of gyration in first case to second case is

365928 The moment of inertia of a uniform rod of length \(2 l\) and mass \(m\) about an axis \(x x^{\prime}\) passing through its centre and inclined at an angle \(\alpha\) is

365929 Match the column I (rotation of different bodies) with column II (their moment of inertia) and select the correct answer from the codes given below.
Column I
Column II
A
Circular ring of radius \(R\) having axis perpendicular to the plane and passing through centre
P
\(M{R^2}/2\)
B
Thin circular ring of radius \(R\) having axis passing through its diameter
Q
\(M{L^2}/3\)
C
Thin rod of length \(L\) about an axis passing through end point
R
\(M{R^2}\)
D
Circular disc of radius \(R\) about an axis perpendicular to the disc and touching the edge.
S
\(3M{R^2}/2\)

365926 A thin uniform rectangular plate of mass \(2\;kg\) is placed in \(x-y\) plane as shown in figure.
The moment of inertia about \(X\)-axis is \({I_x} = 0.2\,kg\,{m^2}\) and moment of ineria about \(y\)-axis is \({I_x} = 0.3\,kg{\mkern 1mu} {m^2}\). The radius of gyration of the plate about the axis passing through O and per perpendicular to the plane of the plate is

365927 Let \(M\) be the mass and \(L\) be the length of a thin uniform rod. In first case, axis of rotation is passing through centre and perpendicular to the length of the rod. In second case, axis of rotation is passing through one end and perpendicular to the length of the rod. The ratio of radius of gyration in first case to second case is

365928 The moment of inertia of a uniform rod of length \(2 l\) and mass \(m\) about an axis \(x x^{\prime}\) passing through its centre and inclined at an angle \(\alpha\) is

365929 Match the column I (rotation of different bodies) with column II (their moment of inertia) and select the correct answer from the codes given below.
Column I
Column II
A
Circular ring of radius \(R\) having axis perpendicular to the plane and passing through centre
P
\(M{R^2}/2\)
B
Thin circular ring of radius \(R\) having axis passing through its diameter
Q
\(M{L^2}/3\)
C
Thin rod of length \(L\) about an axis passing through end point
R
\(M{R^2}\)
D
Circular disc of radius \(R\) about an axis perpendicular to the disc and touching the edge.
S
\(3M{R^2}/2\)

365926 A thin uniform rectangular plate of mass \(2\;kg\) is placed in \(x-y\) plane as shown in figure.
The moment of inertia about \(X\)-axis is \({I_x} = 0.2\,kg\,{m^2}\) and moment of ineria about \(y\)-axis is \({I_x} = 0.3\,kg{\mkern 1mu} {m^2}\). The radius of gyration of the plate about the axis passing through O and per perpendicular to the plane of the plate is

365927 Let \(M\) be the mass and \(L\) be the length of a thin uniform rod. In first case, axis of rotation is passing through centre and perpendicular to the length of the rod. In second case, axis of rotation is passing through one end and perpendicular to the length of the rod. The ratio of radius of gyration in first case to second case is

365928 The moment of inertia of a uniform rod of length \(2 l\) and mass \(m\) about an axis \(x x^{\prime}\) passing through its centre and inclined at an angle \(\alpha\) is

365929 Match the column I (rotation of different bodies) with column II (their moment of inertia) and select the correct answer from the codes given below.
Column I
Column II
A
Circular ring of radius \(R\) having axis perpendicular to the plane and passing through centre
P
\(M{R^2}/2\)
B
Thin circular ring of radius \(R\) having axis passing through its diameter
Q
\(M{L^2}/3\)
C
Thin rod of length \(L\) about an axis passing through end point
R
\(M{R^2}\)
D
Circular disc of radius \(R\) about an axis perpendicular to the disc and touching the edge.
S
\(3M{R^2}/2\)