365732 Three particles of masses \(1\;kg\), \(\frac{3}{2}\;kg\), and \(2\;kg\) are located at the vertices of an equilateral triangle of side \(a\). Here \(1\;kg\) is placed at origin and \(\dfrac{3}{2} k g\) on the \(x\)-axis. The \(x, y\) coordinates of the centre of mass are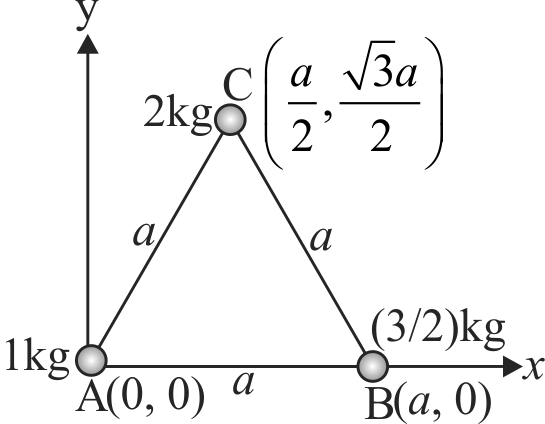

365733 Two masses of 6 and 2 unit are at positions \((6 \hat{i}-7 \hat{j})\) and \((2 \hat{i}+5 \hat{j}-8 \hat{k})\), respectively. The coordinates of the centre of mass are

365734 Assertion :
The centre of mass of system of \(n\) particles is the average of the position of all particles, weighted according to their masses.
Reason :
The position of the centre of mass of a system is independent of co-ordinate system.

365735 The centre of mass of a system of three particles of masses \(1\;g\), \(2\;g\), and \(3\;g\) is taken as the origin of a coordinate system. The position vector of a fourth particle of mass \(4\;g\) such that the centre of mass of the four particle system lies at the point \((1,2,3)\) is \(\alpha(\hat{i}+2 \hat{j}+3 \hat{k})\), where \(\alpha\) is a constant. The value of \(\alpha\) is

365732 Three particles of masses \(1\;kg\), \(\frac{3}{2}\;kg\), and \(2\;kg\) are located at the vertices of an equilateral triangle of side \(a\). Here \(1\;kg\) is placed at origin and \(\dfrac{3}{2} k g\) on the \(x\)-axis. The \(x, y\) coordinates of the centre of mass are

365733 Two masses of 6 and 2 unit are at positions \((6 \hat{i}-7 \hat{j})\) and \((2 \hat{i}+5 \hat{j}-8 \hat{k})\), respectively. The coordinates of the centre of mass are

365734 Assertion :
The centre of mass of system of \(n\) particles is the average of the position of all particles, weighted according to their masses.
Reason :
The position of the centre of mass of a system is independent of co-ordinate system.

365735 The centre of mass of a system of three particles of masses \(1\;g\), \(2\;g\), and \(3\;g\) is taken as the origin of a coordinate system. The position vector of a fourth particle of mass \(4\;g\) such that the centre of mass of the four particle system lies at the point \((1,2,3)\) is \(\alpha(\hat{i}+2 \hat{j}+3 \hat{k})\), where \(\alpha\) is a constant. The value of \(\alpha\) is

365732 Three particles of masses \(1\;kg\), \(\frac{3}{2}\;kg\), and \(2\;kg\) are located at the vertices of an equilateral triangle of side \(a\). Here \(1\;kg\) is placed at origin and \(\dfrac{3}{2} k g\) on the \(x\)-axis. The \(x, y\) coordinates of the centre of mass are

365733 Two masses of 6 and 2 unit are at positions \((6 \hat{i}-7 \hat{j})\) and \((2 \hat{i}+5 \hat{j}-8 \hat{k})\), respectively. The coordinates of the centre of mass are

365734 Assertion :
The centre of mass of system of \(n\) particles is the average of the position of all particles, weighted according to their masses.
Reason :
The position of the centre of mass of a system is independent of co-ordinate system.

365735 The centre of mass of a system of three particles of masses \(1\;g\), \(2\;g\), and \(3\;g\) is taken as the origin of a coordinate system. The position vector of a fourth particle of mass \(4\;g\) such that the centre of mass of the four particle system lies at the point \((1,2,3)\) is \(\alpha(\hat{i}+2 \hat{j}+3 \hat{k})\), where \(\alpha\) is a constant. The value of \(\alpha\) is

365732 Three particles of masses \(1\;kg\), \(\frac{3}{2}\;kg\), and \(2\;kg\) are located at the vertices of an equilateral triangle of side \(a\). Here \(1\;kg\) is placed at origin and \(\dfrac{3}{2} k g\) on the \(x\)-axis. The \(x, y\) coordinates of the centre of mass are

365733 Two masses of 6 and 2 unit are at positions \((6 \hat{i}-7 \hat{j})\) and \((2 \hat{i}+5 \hat{j}-8 \hat{k})\), respectively. The coordinates of the centre of mass are

365734 Assertion :
The centre of mass of system of \(n\) particles is the average of the position of all particles, weighted according to their masses.
Reason :
The position of the centre of mass of a system is independent of co-ordinate system.

365735 The centre of mass of a system of three particles of masses \(1\;g\), \(2\;g\), and \(3\;g\) is taken as the origin of a coordinate system. The position vector of a fourth particle of mass \(4\;g\) such that the centre of mass of the four particle system lies at the point \((1,2,3)\) is \(\alpha(\hat{i}+2 \hat{j}+3 \hat{k})\), where \(\alpha\) is a constant. The value of \(\alpha\) is