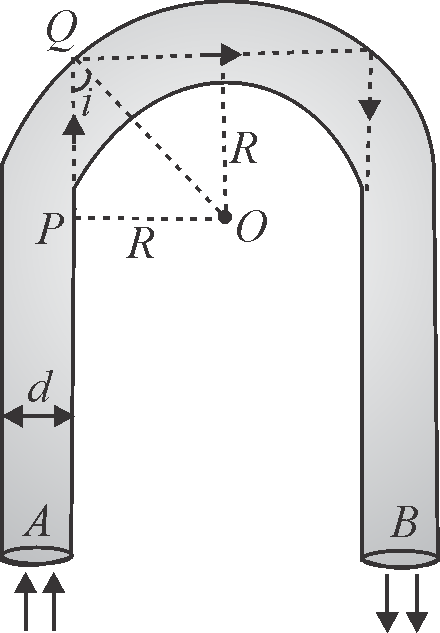
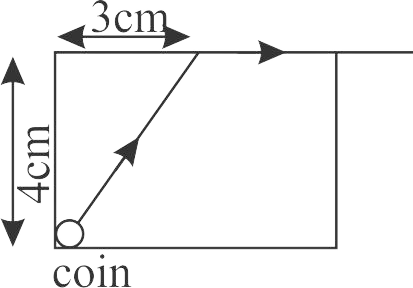
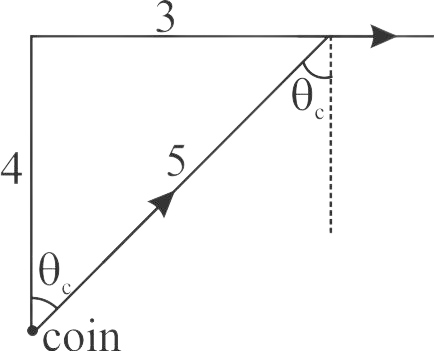
364932 A rod made of glass \({(\mu=1.5)}\) and of square cross-section is bent into the shape shown in figure. A parallel beam of light falls perpendicularly on the plane flat surface \({d}\). Referring to the diagram, \({d}\) is the width of a side and \({R}\) the radius of inner semi-circle. Find the maximum value of ratio \({(d / R)}\) so that all light entering the glass through surface \({A}\) emerge from the glass through \({B}\).

364933 A small coin is resting on the bottom of a beaker filled with a liquid. A ray of light from the coin travels up to the surface of the liquid and moves along its surface (see figure).
How fast is the light travelling in the liquid?

364934 A cut diamond sparkles because of its

364935 Wavelength of given light waves in air and in a medium are \(6000\;\mathop A\limits^ \circ \) and \(4000\;\mathop A\limits^ \circ \) respectively. The critical angle is

364932 A rod made of glass \({(\mu=1.5)}\) and of square cross-section is bent into the shape shown in figure. A parallel beam of light falls perpendicularly on the plane flat surface \({d}\). Referring to the diagram, \({d}\) is the width of a side and \({R}\) the radius of inner semi-circle. Find the maximum value of ratio \({(d / R)}\) so that all light entering the glass through surface \({A}\) emerge from the glass through \({B}\).

364933 A small coin is resting on the bottom of a beaker filled with a liquid. A ray of light from the coin travels up to the surface of the liquid and moves along its surface (see figure).
How fast is the light travelling in the liquid?

364934 A cut diamond sparkles because of its

364935 Wavelength of given light waves in air and in a medium are \(6000\;\mathop A\limits^ \circ \) and \(4000\;\mathop A\limits^ \circ \) respectively. The critical angle is

364932 A rod made of glass \({(\mu=1.5)}\) and of square cross-section is bent into the shape shown in figure. A parallel beam of light falls perpendicularly on the plane flat surface \({d}\). Referring to the diagram, \({d}\) is the width of a side and \({R}\) the radius of inner semi-circle. Find the maximum value of ratio \({(d / R)}\) so that all light entering the glass through surface \({A}\) emerge from the glass through \({B}\).

364933 A small coin is resting on the bottom of a beaker filled with a liquid. A ray of light from the coin travels up to the surface of the liquid and moves along its surface (see figure).
How fast is the light travelling in the liquid?

364934 A cut diamond sparkles because of its

364935 Wavelength of given light waves in air and in a medium are \(6000\;\mathop A\limits^ \circ \) and \(4000\;\mathop A\limits^ \circ \) respectively. The critical angle is

364932 A rod made of glass \({(\mu=1.5)}\) and of square cross-section is bent into the shape shown in figure. A parallel beam of light falls perpendicularly on the plane flat surface \({d}\). Referring to the diagram, \({d}\) is the width of a side and \({R}\) the radius of inner semi-circle. Find the maximum value of ratio \({(d / R)}\) so that all light entering the glass through surface \({A}\) emerge from the glass through \({B}\).

364933 A small coin is resting on the bottom of a beaker filled with a liquid. A ray of light from the coin travels up to the surface of the liquid and moves along its surface (see figure).
How fast is the light travelling in the liquid?

364934 A cut diamond sparkles because of its

364935 Wavelength of given light waves in air and in a medium are \(6000\;\mathop A\limits^ \circ \) and \(4000\;\mathop A\limits^ \circ \) respectively. The critical angle is