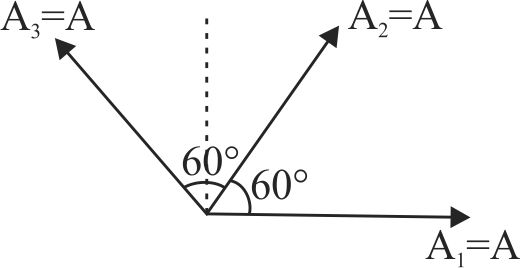
364511 Three simple harmonic motions, of equal amplitudes \(A\) and equal time periods, along the same line combine. The phase of the second motion is \(60^{\circ}\) ahead of the first and phase of the third motion is \(60^{\circ}\) ahead of the second. The amplitude of resultant motion is ____.

364512 The displacement \(x\) of a particle executing a certain periodic motion is given by \(y=25 \sin (4 t) \cos ^{2}(5 t)\). This expression may be considered to be the superposition of \(n\) independent harmonic motions. What is the value of \(n\) ?

364513 Two linear simple harmonic motions of equal amplitude and frequency are imposed on a particle along \(x\) and \(y\) axis respectively. The initial phase difference between them is \(\dfrac{\pi}{2}\). The resultant path followed by the particle is:

364514 A particle is executing two different simple harmonic motions, mutually perpendicular, of different amplitudes and having phase difference of \(\pi / 2\). The path of the particle will be

364515 Two SHMs \(r_{1}=A \sin \omega t\) and \(r_{2}=B \sin \omega t\) are superposed on a particle. \(r_{1}\) and \(r_{2}\) are along the directions which make \(37^{0}\) with each other. Choose the correct statement

364511 Three simple harmonic motions, of equal amplitudes \(A\) and equal time periods, along the same line combine. The phase of the second motion is \(60^{\circ}\) ahead of the first and phase of the third motion is \(60^{\circ}\) ahead of the second. The amplitude of resultant motion is ____.

364512 The displacement \(x\) of a particle executing a certain periodic motion is given by \(y=25 \sin (4 t) \cos ^{2}(5 t)\). This expression may be considered to be the superposition of \(n\) independent harmonic motions. What is the value of \(n\) ?

364513 Two linear simple harmonic motions of equal amplitude and frequency are imposed on a particle along \(x\) and \(y\) axis respectively. The initial phase difference between them is \(\dfrac{\pi}{2}\). The resultant path followed by the particle is:

364514 A particle is executing two different simple harmonic motions, mutually perpendicular, of different amplitudes and having phase difference of \(\pi / 2\). The path of the particle will be

364515 Two SHMs \(r_{1}=A \sin \omega t\) and \(r_{2}=B \sin \omega t\) are superposed on a particle. \(r_{1}\) and \(r_{2}\) are along the directions which make \(37^{0}\) with each other. Choose the correct statement

364511 Three simple harmonic motions, of equal amplitudes \(A\) and equal time periods, along the same line combine. The phase of the second motion is \(60^{\circ}\) ahead of the first and phase of the third motion is \(60^{\circ}\) ahead of the second. The amplitude of resultant motion is ____.

364512 The displacement \(x\) of a particle executing a certain periodic motion is given by \(y=25 \sin (4 t) \cos ^{2}(5 t)\). This expression may be considered to be the superposition of \(n\) independent harmonic motions. What is the value of \(n\) ?

364513 Two linear simple harmonic motions of equal amplitude and frequency are imposed on a particle along \(x\) and \(y\) axis respectively. The initial phase difference between them is \(\dfrac{\pi}{2}\). The resultant path followed by the particle is:

364514 A particle is executing two different simple harmonic motions, mutually perpendicular, of different amplitudes and having phase difference of \(\pi / 2\). The path of the particle will be

364515 Two SHMs \(r_{1}=A \sin \omega t\) and \(r_{2}=B \sin \omega t\) are superposed on a particle. \(r_{1}\) and \(r_{2}\) are along the directions which make \(37^{0}\) with each other. Choose the correct statement

364511 Three simple harmonic motions, of equal amplitudes \(A\) and equal time periods, along the same line combine. The phase of the second motion is \(60^{\circ}\) ahead of the first and phase of the third motion is \(60^{\circ}\) ahead of the second. The amplitude of resultant motion is ____.

364512 The displacement \(x\) of a particle executing a certain periodic motion is given by \(y=25 \sin (4 t) \cos ^{2}(5 t)\). This expression may be considered to be the superposition of \(n\) independent harmonic motions. What is the value of \(n\) ?

364513 Two linear simple harmonic motions of equal amplitude and frequency are imposed on a particle along \(x\) and \(y\) axis respectively. The initial phase difference between them is \(\dfrac{\pi}{2}\). The resultant path followed by the particle is:

364514 A particle is executing two different simple harmonic motions, mutually perpendicular, of different amplitudes and having phase difference of \(\pi / 2\). The path of the particle will be

364515 Two SHMs \(r_{1}=A \sin \omega t\) and \(r_{2}=B \sin \omega t\) are superposed on a particle. \(r_{1}\) and \(r_{2}\) are along the directions which make \(37^{0}\) with each other. Choose the correct statement

364511 Three simple harmonic motions, of equal amplitudes \(A\) and equal time periods, along the same line combine. The phase of the second motion is \(60^{\circ}\) ahead of the first and phase of the third motion is \(60^{\circ}\) ahead of the second. The amplitude of resultant motion is ____.

364512 The displacement \(x\) of a particle executing a certain periodic motion is given by \(y=25 \sin (4 t) \cos ^{2}(5 t)\). This expression may be considered to be the superposition of \(n\) independent harmonic motions. What is the value of \(n\) ?

364513 Two linear simple harmonic motions of equal amplitude and frequency are imposed on a particle along \(x\) and \(y\) axis respectively. The initial phase difference between them is \(\dfrac{\pi}{2}\). The resultant path followed by the particle is:

364514 A particle is executing two different simple harmonic motions, mutually perpendicular, of different amplitudes and having phase difference of \(\pi / 2\). The path of the particle will be

364515 Two SHMs \(r_{1}=A \sin \omega t\) and \(r_{2}=B \sin \omega t\) are superposed on a particle. \(r_{1}\) and \(r_{2}\) are along the directions which make \(37^{0}\) with each other. Choose the correct statement