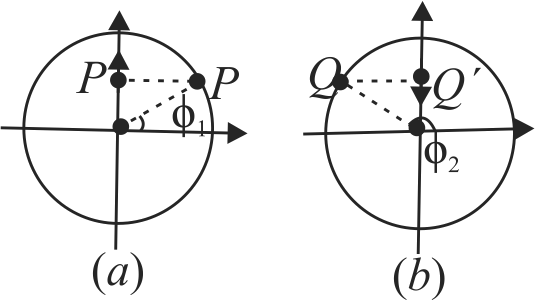
364211 Two particles execute SHM with same amplitudes and same angular frequency on same straight line with same mean position. Given that during oscillation they cross each other in opposite direction when at a distance \(A / 2\) from mean position. Find phase difference in the two \(SHMs\).
364214
Two simple harmonic motions exhibited by two particles \(A\) and \(B\) are given respectively by the following equations.
\({y_1} = a\sin \left[ {\omega t + \frac{\pi }{6}} \right]\)
\({y_2} = a\sin \left[ {\omega t + \frac{{3\pi }}{6}} \right]\)
Phase difference between them is
364211 Two particles execute SHM with same amplitudes and same angular frequency on same straight line with same mean position. Given that during oscillation they cross each other in opposite direction when at a distance \(A / 2\) from mean position. Find phase difference in the two \(SHMs\).
364214
Two simple harmonic motions exhibited by two particles \(A\) and \(B\) are given respectively by the following equations.
\({y_1} = a\sin \left[ {\omega t + \frac{\pi }{6}} \right]\)
\({y_2} = a\sin \left[ {\omega t + \frac{{3\pi }}{6}} \right]\)
Phase difference between them is
364211 Two particles execute SHM with same amplitudes and same angular frequency on same straight line with same mean position. Given that during oscillation they cross each other in opposite direction when at a distance \(A / 2\) from mean position. Find phase difference in the two \(SHMs\).
364214
Two simple harmonic motions exhibited by two particles \(A\) and \(B\) are given respectively by the following equations.
\({y_1} = a\sin \left[ {\omega t + \frac{\pi }{6}} \right]\)
\({y_2} = a\sin \left[ {\omega t + \frac{{3\pi }}{6}} \right]\)
Phase difference between them is
364211 Two particles execute SHM with same amplitudes and same angular frequency on same straight line with same mean position. Given that during oscillation they cross each other in opposite direction when at a distance \(A / 2\) from mean position. Find phase difference in the two \(SHMs\).
364214
Two simple harmonic motions exhibited by two particles \(A\) and \(B\) are given respectively by the following equations.
\({y_1} = a\sin \left[ {\omega t + \frac{\pi }{6}} \right]\)
\({y_2} = a\sin \left[ {\omega t + \frac{{3\pi }}{6}} \right]\)
Phase difference between them is
364211 Two particles execute SHM with same amplitudes and same angular frequency on same straight line with same mean position. Given that during oscillation they cross each other in opposite direction when at a distance \(A / 2\) from mean position. Find phase difference in the two \(SHMs\).
364214
Two simple harmonic motions exhibited by two particles \(A\) and \(B\) are given respectively by the following equations.
\({y_1} = a\sin \left[ {\omega t + \frac{\pi }{6}} \right]\)
\({y_2} = a\sin \left[ {\omega t + \frac{{3\pi }}{6}} \right]\)
Phase difference between them is