320481
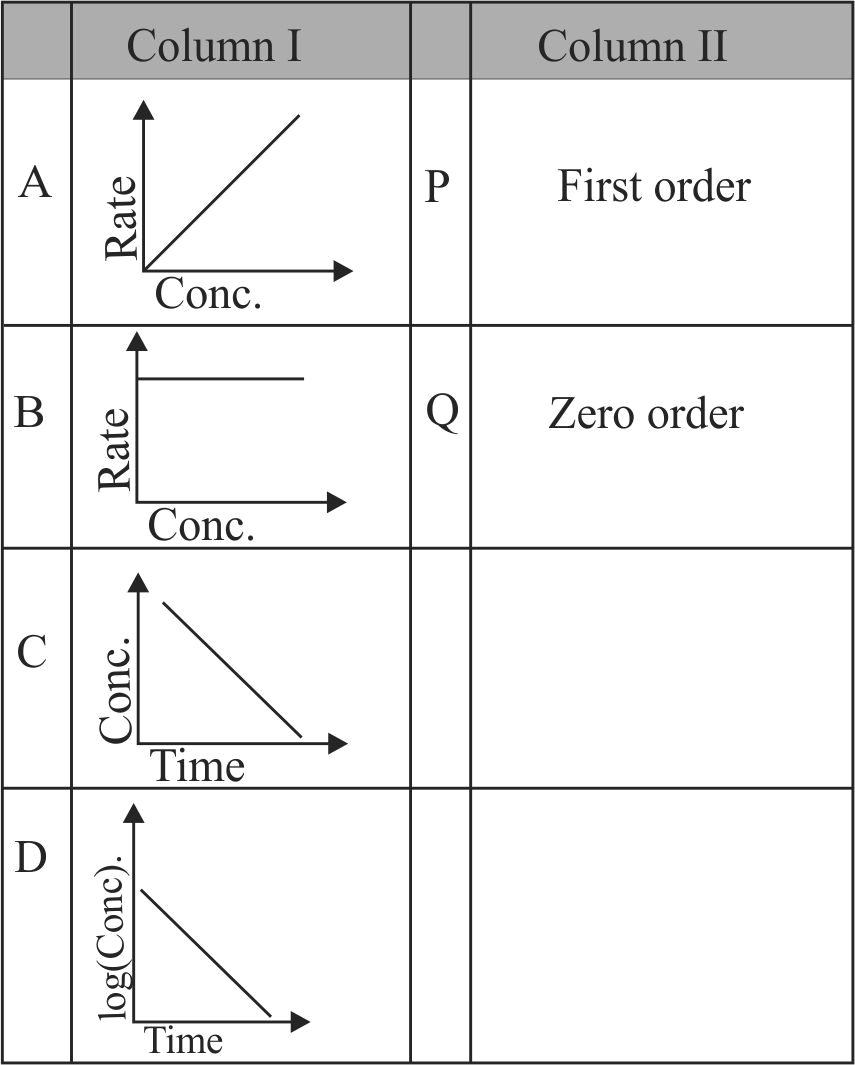
Match the terms of Column I with Column II and choose the correct option from the codes given below.
Column I
Column II
A
\(\begin{array}{l}\text { Inversion of } \\\text { cane sugar. }\end{array}\)
P
\(\begin{array}{l}\text { Zero order } \\\text { reaction }\end{array}\)
B
\(\begin{array}{l}\text { Decomposition } \\\text { of } \mathrm{N}_{2} \mathrm{O}\end{array}\)
Q
\(\begin{array}{l}\text { First order } \\\text { reaction }\end{array}\)
C
\(\begin{array}{l}\text { Thermal } \\\text { decomposition } \\\text { of HI on gold } \\\text { surface }\end{array}\)
R
\(\begin{array}{l}\text { Pseudo first } \\\text { order reaction }\end{array}\)
320482 Consider a reaction \({\text{aG + bH}} \to \) products. When concentration of both the reactants \(G\) and \(\mathrm{H}\) is doubled, the rate increases by eight times. However, when concentration of \(\mathrm{G}\) is doubled keeping the concentration of \(\mathrm{H}\) fixed, the rate is doubled. The overall order of the reaction is
320484 The rate constant (k) for the reaction, \({\rm{2\;A + B}} \to \) Product was found to be \({\rm{2}}{\rm{.5 \times 1}}{{\rm{0}}^{{\rm{ - 5}}}}\) lit \({\rm{mo}}{{\rm{l}}^{{\rm{ - 1}}}}{\rm{se}}{{\rm{c}}^{{\rm{ - 1}}}}\) after \({\rm{30}}\,{\rm{sec}}\) and \({\rm{2}}{\rm{.55 \times 1}}{{\rm{0}}^{{\rm{ - 5}}}}\) lit \({\rm{mo}}{{\rm{l}}^{{\rm{ - 1}}}}\,{\rm{se}}{{\rm{c}}^{{\rm{ - 1}}}}\,{\rm{after}}\,{\rm{50}}\,{\rm{sec}}\). The order of the reaction is
320481
Match the terms of Column I with Column II and choose the correct option from the codes given below.
Column I
Column II
A
\(\begin{array}{l}\text { Inversion of } \\\text { cane sugar. }\end{array}\)
P
\(\begin{array}{l}\text { Zero order } \\\text { reaction }\end{array}\)
B
\(\begin{array}{l}\text { Decomposition } \\\text { of } \mathrm{N}_{2} \mathrm{O}\end{array}\)
Q
\(\begin{array}{l}\text { First order } \\\text { reaction }\end{array}\)
C
\(\begin{array}{l}\text { Thermal } \\\text { decomposition } \\\text { of HI on gold } \\\text { surface }\end{array}\)
R
\(\begin{array}{l}\text { Pseudo first } \\\text { order reaction }\end{array}\)
320482 Consider a reaction \({\text{aG + bH}} \to \) products. When concentration of both the reactants \(G\) and \(\mathrm{H}\) is doubled, the rate increases by eight times. However, when concentration of \(\mathrm{G}\) is doubled keeping the concentration of \(\mathrm{H}\) fixed, the rate is doubled. The overall order of the reaction is
320484 The rate constant (k) for the reaction, \({\rm{2\;A + B}} \to \) Product was found to be \({\rm{2}}{\rm{.5 \times 1}}{{\rm{0}}^{{\rm{ - 5}}}}\) lit \({\rm{mo}}{{\rm{l}}^{{\rm{ - 1}}}}{\rm{se}}{{\rm{c}}^{{\rm{ - 1}}}}\) after \({\rm{30}}\,{\rm{sec}}\) and \({\rm{2}}{\rm{.55 \times 1}}{{\rm{0}}^{{\rm{ - 5}}}}\) lit \({\rm{mo}}{{\rm{l}}^{{\rm{ - 1}}}}\,{\rm{se}}{{\rm{c}}^{{\rm{ - 1}}}}\,{\rm{after}}\,{\rm{50}}\,{\rm{sec}}\). The order of the reaction is
320481
Match the terms of Column I with Column II and choose the correct option from the codes given below.
Column I
Column II
A
\(\begin{array}{l}\text { Inversion of } \\\text { cane sugar. }\end{array}\)
P
\(\begin{array}{l}\text { Zero order } \\\text { reaction }\end{array}\)
B
\(\begin{array}{l}\text { Decomposition } \\\text { of } \mathrm{N}_{2} \mathrm{O}\end{array}\)
Q
\(\begin{array}{l}\text { First order } \\\text { reaction }\end{array}\)
C
\(\begin{array}{l}\text { Thermal } \\\text { decomposition } \\\text { of HI on gold } \\\text { surface }\end{array}\)
R
\(\begin{array}{l}\text { Pseudo first } \\\text { order reaction }\end{array}\)
320482 Consider a reaction \({\text{aG + bH}} \to \) products. When concentration of both the reactants \(G\) and \(\mathrm{H}\) is doubled, the rate increases by eight times. However, when concentration of \(\mathrm{G}\) is doubled keeping the concentration of \(\mathrm{H}\) fixed, the rate is doubled. The overall order of the reaction is
320484 The rate constant (k) for the reaction, \({\rm{2\;A + B}} \to \) Product was found to be \({\rm{2}}{\rm{.5 \times 1}}{{\rm{0}}^{{\rm{ - 5}}}}\) lit \({\rm{mo}}{{\rm{l}}^{{\rm{ - 1}}}}{\rm{se}}{{\rm{c}}^{{\rm{ - 1}}}}\) after \({\rm{30}}\,{\rm{sec}}\) and \({\rm{2}}{\rm{.55 \times 1}}{{\rm{0}}^{{\rm{ - 5}}}}\) lit \({\rm{mo}}{{\rm{l}}^{{\rm{ - 1}}}}\,{\rm{se}}{{\rm{c}}^{{\rm{ - 1}}}}\,{\rm{after}}\,{\rm{50}}\,{\rm{sec}}\). The order of the reaction is
320481
Match the terms of Column I with Column II and choose the correct option from the codes given below.
Column I
Column II
A
\(\begin{array}{l}\text { Inversion of } \\\text { cane sugar. }\end{array}\)
P
\(\begin{array}{l}\text { Zero order } \\\text { reaction }\end{array}\)
B
\(\begin{array}{l}\text { Decomposition } \\\text { of } \mathrm{N}_{2} \mathrm{O}\end{array}\)
Q
\(\begin{array}{l}\text { First order } \\\text { reaction }\end{array}\)
C
\(\begin{array}{l}\text { Thermal } \\\text { decomposition } \\\text { of HI on gold } \\\text { surface }\end{array}\)
R
\(\begin{array}{l}\text { Pseudo first } \\\text { order reaction }\end{array}\)
320482 Consider a reaction \({\text{aG + bH}} \to \) products. When concentration of both the reactants \(G\) and \(\mathrm{H}\) is doubled, the rate increases by eight times. However, when concentration of \(\mathrm{G}\) is doubled keeping the concentration of \(\mathrm{H}\) fixed, the rate is doubled. The overall order of the reaction is
320484 The rate constant (k) for the reaction, \({\rm{2\;A + B}} \to \) Product was found to be \({\rm{2}}{\rm{.5 \times 1}}{{\rm{0}}^{{\rm{ - 5}}}}\) lit \({\rm{mo}}{{\rm{l}}^{{\rm{ - 1}}}}{\rm{se}}{{\rm{c}}^{{\rm{ - 1}}}}\) after \({\rm{30}}\,{\rm{sec}}\) and \({\rm{2}}{\rm{.55 \times 1}}{{\rm{0}}^{{\rm{ - 5}}}}\) lit \({\rm{mo}}{{\rm{l}}^{{\rm{ - 1}}}}\,{\rm{se}}{{\rm{c}}^{{\rm{ - 1}}}}\,{\rm{after}}\,{\rm{50}}\,{\rm{sec}}\). The order of the reaction is
320481
Match the terms of Column I with Column II and choose the correct option from the codes given below.
Column I
Column II
A
\(\begin{array}{l}\text { Inversion of } \\\text { cane sugar. }\end{array}\)
P
\(\begin{array}{l}\text { Zero order } \\\text { reaction }\end{array}\)
B
\(\begin{array}{l}\text { Decomposition } \\\text { of } \mathrm{N}_{2} \mathrm{O}\end{array}\)
Q
\(\begin{array}{l}\text { First order } \\\text { reaction }\end{array}\)
C
\(\begin{array}{l}\text { Thermal } \\\text { decomposition } \\\text { of HI on gold } \\\text { surface }\end{array}\)
R
\(\begin{array}{l}\text { Pseudo first } \\\text { order reaction }\end{array}\)
320482 Consider a reaction \({\text{aG + bH}} \to \) products. When concentration of both the reactants \(G\) and \(\mathrm{H}\) is doubled, the rate increases by eight times. However, when concentration of \(\mathrm{G}\) is doubled keeping the concentration of \(\mathrm{H}\) fixed, the rate is doubled. The overall order of the reaction is
320484 The rate constant (k) for the reaction, \({\rm{2\;A + B}} \to \) Product was found to be \({\rm{2}}{\rm{.5 \times 1}}{{\rm{0}}^{{\rm{ - 5}}}}\) lit \({\rm{mo}}{{\rm{l}}^{{\rm{ - 1}}}}{\rm{se}}{{\rm{c}}^{{\rm{ - 1}}}}\) after \({\rm{30}}\,{\rm{sec}}\) and \({\rm{2}}{\rm{.55 \times 1}}{{\rm{0}}^{{\rm{ - 5}}}}\) lit \({\rm{mo}}{{\rm{l}}^{{\rm{ - 1}}}}\,{\rm{se}}{{\rm{c}}^{{\rm{ - 1}}}}\,{\rm{after}}\,{\rm{50}}\,{\rm{sec}}\). The order of the reaction is