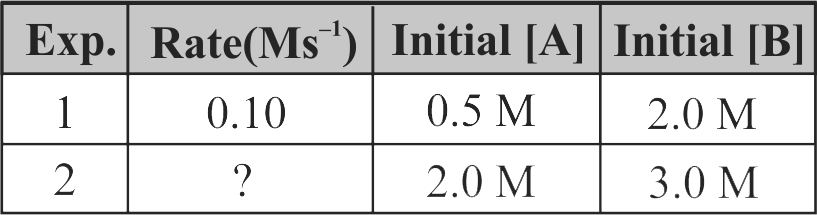
320184 \(\mathrm{A}+2 \mathrm{~B} \rightarrow \mathrm{C}\), the rate equation for this reaction is given as, Rate \(=\mathrm{k}[\mathrm{A}][\mathrm{B}]\).
If the concentration of \(\mathrm{A}\) is kept the same but that of \(\mathrm{B}\) is doubled what will happen to the rate itself?

320185 For the reaction, \({\rm{2\;}}{{\rm{N}}_{\rm{2}}}{{\rm{O}}_{\rm{5}}}{\rm{(g)}} \to {\rm{4N}}{{\rm{O}}_{\rm{2}}}{\rm{(g) + }}{{\rm{O}}_{\rm{2}}}{\rm{(g)}}\) rate of reaction and rate constant are \({\rm{1}}{\rm{.2 \times 1}}{{\rm{0}}^{{\rm{ - 4}}}}\) and \({\rm{4 \times 1}}{{\rm{0}}^{{\rm{ - 5}}}}{\rm{se}}{{\rm{c}}^{{\rm{ - 1}}}}\) respectively. The concentration of \({{\rm{N}}_{\rm{2}}}{{\rm{O}}_{\rm{5}}}\) at that time will be:

320186 Consider a chemical reaction involving compounds A and B , which is found to be second order in A and first order in B. At what rate, will the reaction occur in experiment 2 ?

320187 For a certain reaction, the rate \({\text{ = k [A}}{{\text{]}}^{\text{2}}}{\text{ [B]}}\), when the initial concentration of A is tripled keeping concentration of B constant, the initial rate would

320188 Select the rate law that corresponds to the data shown for the following reaction:
\(A+B \rightarrow C\)

320184 \(\mathrm{A}+2 \mathrm{~B} \rightarrow \mathrm{C}\), the rate equation for this reaction is given as, Rate \(=\mathrm{k}[\mathrm{A}][\mathrm{B}]\).
If the concentration of \(\mathrm{A}\) is kept the same but that of \(\mathrm{B}\) is doubled what will happen to the rate itself?

320185 For the reaction, \({\rm{2\;}}{{\rm{N}}_{\rm{2}}}{{\rm{O}}_{\rm{5}}}{\rm{(g)}} \to {\rm{4N}}{{\rm{O}}_{\rm{2}}}{\rm{(g) + }}{{\rm{O}}_{\rm{2}}}{\rm{(g)}}\) rate of reaction and rate constant are \({\rm{1}}{\rm{.2 \times 1}}{{\rm{0}}^{{\rm{ - 4}}}}\) and \({\rm{4 \times 1}}{{\rm{0}}^{{\rm{ - 5}}}}{\rm{se}}{{\rm{c}}^{{\rm{ - 1}}}}\) respectively. The concentration of \({{\rm{N}}_{\rm{2}}}{{\rm{O}}_{\rm{5}}}\) at that time will be:

320186 Consider a chemical reaction involving compounds A and B , which is found to be second order in A and first order in B. At what rate, will the reaction occur in experiment 2 ?

320187 For a certain reaction, the rate \({\text{ = k [A}}{{\text{]}}^{\text{2}}}{\text{ [B]}}\), when the initial concentration of A is tripled keeping concentration of B constant, the initial rate would

320188 Select the rate law that corresponds to the data shown for the following reaction:
\(A+B \rightarrow C\)

320184 \(\mathrm{A}+2 \mathrm{~B} \rightarrow \mathrm{C}\), the rate equation for this reaction is given as, Rate \(=\mathrm{k}[\mathrm{A}][\mathrm{B}]\).
If the concentration of \(\mathrm{A}\) is kept the same but that of \(\mathrm{B}\) is doubled what will happen to the rate itself?

320185 For the reaction, \({\rm{2\;}}{{\rm{N}}_{\rm{2}}}{{\rm{O}}_{\rm{5}}}{\rm{(g)}} \to {\rm{4N}}{{\rm{O}}_{\rm{2}}}{\rm{(g) + }}{{\rm{O}}_{\rm{2}}}{\rm{(g)}}\) rate of reaction and rate constant are \({\rm{1}}{\rm{.2 \times 1}}{{\rm{0}}^{{\rm{ - 4}}}}\) and \({\rm{4 \times 1}}{{\rm{0}}^{{\rm{ - 5}}}}{\rm{se}}{{\rm{c}}^{{\rm{ - 1}}}}\) respectively. The concentration of \({{\rm{N}}_{\rm{2}}}{{\rm{O}}_{\rm{5}}}\) at that time will be:

320186 Consider a chemical reaction involving compounds A and B , which is found to be second order in A and first order in B. At what rate, will the reaction occur in experiment 2 ?

320187 For a certain reaction, the rate \({\text{ = k [A}}{{\text{]}}^{\text{2}}}{\text{ [B]}}\), when the initial concentration of A is tripled keeping concentration of B constant, the initial rate would

320188 Select the rate law that corresponds to the data shown for the following reaction:
\(A+B \rightarrow C\)

320184 \(\mathrm{A}+2 \mathrm{~B} \rightarrow \mathrm{C}\), the rate equation for this reaction is given as, Rate \(=\mathrm{k}[\mathrm{A}][\mathrm{B}]\).
If the concentration of \(\mathrm{A}\) is kept the same but that of \(\mathrm{B}\) is doubled what will happen to the rate itself?

320185 For the reaction, \({\rm{2\;}}{{\rm{N}}_{\rm{2}}}{{\rm{O}}_{\rm{5}}}{\rm{(g)}} \to {\rm{4N}}{{\rm{O}}_{\rm{2}}}{\rm{(g) + }}{{\rm{O}}_{\rm{2}}}{\rm{(g)}}\) rate of reaction and rate constant are \({\rm{1}}{\rm{.2 \times 1}}{{\rm{0}}^{{\rm{ - 4}}}}\) and \({\rm{4 \times 1}}{{\rm{0}}^{{\rm{ - 5}}}}{\rm{se}}{{\rm{c}}^{{\rm{ - 1}}}}\) respectively. The concentration of \({{\rm{N}}_{\rm{2}}}{{\rm{O}}_{\rm{5}}}\) at that time will be:

320186 Consider a chemical reaction involving compounds A and B , which is found to be second order in A and first order in B. At what rate, will the reaction occur in experiment 2 ?

320187 For a certain reaction, the rate \({\text{ = k [A}}{{\text{]}}^{\text{2}}}{\text{ [B]}}\), when the initial concentration of A is tripled keeping concentration of B constant, the initial rate would

320188 Select the rate law that corresponds to the data shown for the following reaction:
\(A+B \rightarrow C\)

320184 \(\mathrm{A}+2 \mathrm{~B} \rightarrow \mathrm{C}\), the rate equation for this reaction is given as, Rate \(=\mathrm{k}[\mathrm{A}][\mathrm{B}]\).
If the concentration of \(\mathrm{A}\) is kept the same but that of \(\mathrm{B}\) is doubled what will happen to the rate itself?

320185 For the reaction, \({\rm{2\;}}{{\rm{N}}_{\rm{2}}}{{\rm{O}}_{\rm{5}}}{\rm{(g)}} \to {\rm{4N}}{{\rm{O}}_{\rm{2}}}{\rm{(g) + }}{{\rm{O}}_{\rm{2}}}{\rm{(g)}}\) rate of reaction and rate constant are \({\rm{1}}{\rm{.2 \times 1}}{{\rm{0}}^{{\rm{ - 4}}}}\) and \({\rm{4 \times 1}}{{\rm{0}}^{{\rm{ - 5}}}}{\rm{se}}{{\rm{c}}^{{\rm{ - 1}}}}\) respectively. The concentration of \({{\rm{N}}_{\rm{2}}}{{\rm{O}}_{\rm{5}}}\) at that time will be:

320186 Consider a chemical reaction involving compounds A and B , which is found to be second order in A and first order in B. At what rate, will the reaction occur in experiment 2 ?

320187 For a certain reaction, the rate \({\text{ = k [A}}{{\text{]}}^{\text{2}}}{\text{ [B]}}\), when the initial concentration of A is tripled keeping concentration of B constant, the initial rate would

320188 Select the rate law that corresponds to the data shown for the following reaction:
\(A+B \rightarrow C\)