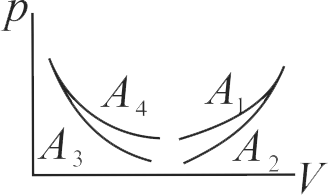
369341
The pressure-volume work for an ideal gas can be calculated by using the expression \({\rm{w = - }}\int_{{{\rm{V}}_{\rm{i}}}}^{{{\rm{v}}_{\rm{f}}}} {{{\rm{p}}_{{\rm{ex}}}}} {\rm{dV}}\). The work can also be calculated from the \(\mathrm{p \mathrm{~V}}\) - plot by using the area under the curve within the specified limits. When an ideal gas is compressed (A) reversibly or (B) irreversibly from volume \(\mathrm{\mathrm{V}_{i}}\) to \(\mathrm{\mathrm{V}_{f}}\) [Here, \(\mathrm{P_{e x}}\) at each stage is equal to \(\left[ {{\rm{V}}\left( {{{\rm{P}}_{{\rm{in }}}}{\rm{ + dp}}} \right)} \right]\). Choose the correct option.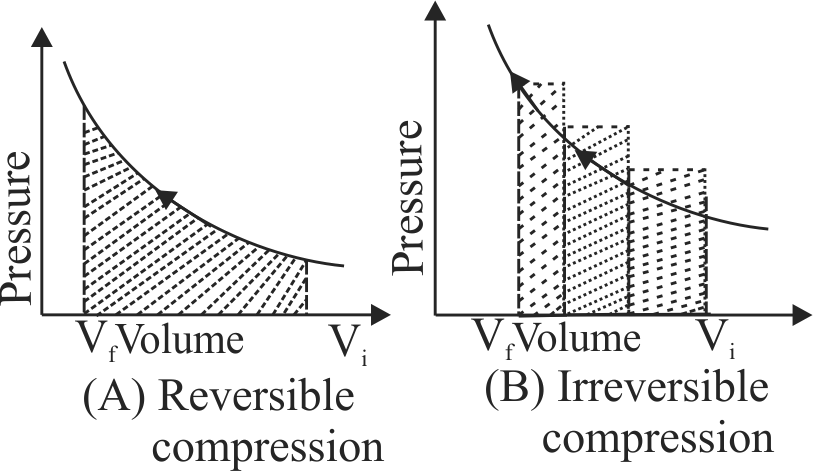
369342
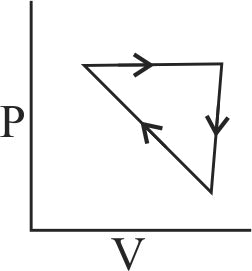
An ideal gas undergoes a cyclic pocess as shown in Figure
\(\Delta {{\text{U}}_{{\text{BC}}}}{\text{ = - 5}}{\mkern 1mu} {\text{k}}{\mkern 1mu} {\text{J}}{\mkern 1mu} {\text{mo}}{{\text{l}}^{{\text{ - 1}}}}{\text{,}}{\mkern 1mu} {{\text{q}}_{{\text{AB}}}}{\text{ = 2}}{\mkern 1mu} {\text{k}}{\mkern 1mu} {\text{J}}{\mkern 1mu} {\text{mo}}{{\text{l}}^{{\text{ - 1}}}}\)
\({{\text{W}}_{{\text{AB}}}}{\text{ = - 5}}\,{\text{k}}\,{\text{J}}\,{\text{mo}}{{\text{l}}^{{\text{ - 1}}}}{\text{,}}\,{{\text{W}}_{{\text{CA}}}}{\text{ = 3}}\,{\text{k}}\,{\text{J}}\,{\text{mo}}{{\text{l}}^{{\text{ - 1}}}}\)
Heat absorbed by the system during process CA is: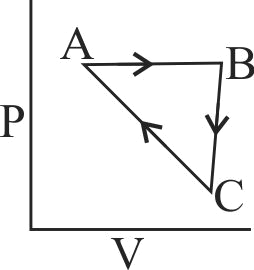
369341
The pressure-volume work for an ideal gas can be calculated by using the expression \({\rm{w = - }}\int_{{{\rm{V}}_{\rm{i}}}}^{{{\rm{v}}_{\rm{f}}}} {{{\rm{p}}_{{\rm{ex}}}}} {\rm{dV}}\). The work can also be calculated from the \(\mathrm{p \mathrm{~V}}\) - plot by using the area under the curve within the specified limits. When an ideal gas is compressed (A) reversibly or (B) irreversibly from volume \(\mathrm{\mathrm{V}_{i}}\) to \(\mathrm{\mathrm{V}_{f}}\) [Here, \(\mathrm{P_{e x}}\) at each stage is equal to \(\left[ {{\rm{V}}\left( {{{\rm{P}}_{{\rm{in }}}}{\rm{ + dp}}} \right)} \right]\). Choose the correct option.
369342
An ideal gas undergoes a cyclic pocess as shown in Figure
\(\Delta {{\text{U}}_{{\text{BC}}}}{\text{ = - 5}}{\mkern 1mu} {\text{k}}{\mkern 1mu} {\text{J}}{\mkern 1mu} {\text{mo}}{{\text{l}}^{{\text{ - 1}}}}{\text{,}}{\mkern 1mu} {{\text{q}}_{{\text{AB}}}}{\text{ = 2}}{\mkern 1mu} {\text{k}}{\mkern 1mu} {\text{J}}{\mkern 1mu} {\text{mo}}{{\text{l}}^{{\text{ - 1}}}}\)
\({{\text{W}}_{{\text{AB}}}}{\text{ = - 5}}\,{\text{k}}\,{\text{J}}\,{\text{mo}}{{\text{l}}^{{\text{ - 1}}}}{\text{,}}\,{{\text{W}}_{{\text{CA}}}}{\text{ = 3}}\,{\text{k}}\,{\text{J}}\,{\text{mo}}{{\text{l}}^{{\text{ - 1}}}}\)
Heat absorbed by the system during process CA is:
369341
The pressure-volume work for an ideal gas can be calculated by using the expression \({\rm{w = - }}\int_{{{\rm{V}}_{\rm{i}}}}^{{{\rm{v}}_{\rm{f}}}} {{{\rm{p}}_{{\rm{ex}}}}} {\rm{dV}}\). The work can also be calculated from the \(\mathrm{p \mathrm{~V}}\) - plot by using the area under the curve within the specified limits. When an ideal gas is compressed (A) reversibly or (B) irreversibly from volume \(\mathrm{\mathrm{V}_{i}}\) to \(\mathrm{\mathrm{V}_{f}}\) [Here, \(\mathrm{P_{e x}}\) at each stage is equal to \(\left[ {{\rm{V}}\left( {{{\rm{P}}_{{\rm{in }}}}{\rm{ + dp}}} \right)} \right]\). Choose the correct option.
369342
An ideal gas undergoes a cyclic pocess as shown in Figure
\(\Delta {{\text{U}}_{{\text{BC}}}}{\text{ = - 5}}{\mkern 1mu} {\text{k}}{\mkern 1mu} {\text{J}}{\mkern 1mu} {\text{mo}}{{\text{l}}^{{\text{ - 1}}}}{\text{,}}{\mkern 1mu} {{\text{q}}_{{\text{AB}}}}{\text{ = 2}}{\mkern 1mu} {\text{k}}{\mkern 1mu} {\text{J}}{\mkern 1mu} {\text{mo}}{{\text{l}}^{{\text{ - 1}}}}\)
\({{\text{W}}_{{\text{AB}}}}{\text{ = - 5}}\,{\text{k}}\,{\text{J}}\,{\text{mo}}{{\text{l}}^{{\text{ - 1}}}}{\text{,}}\,{{\text{W}}_{{\text{CA}}}}{\text{ = 3}}\,{\text{k}}\,{\text{J}}\,{\text{mo}}{{\text{l}}^{{\text{ - 1}}}}\)
Heat absorbed by the system during process CA is:
369341
The pressure-volume work for an ideal gas can be calculated by using the expression \({\rm{w = - }}\int_{{{\rm{V}}_{\rm{i}}}}^{{{\rm{v}}_{\rm{f}}}} {{{\rm{p}}_{{\rm{ex}}}}} {\rm{dV}}\). The work can also be calculated from the \(\mathrm{p \mathrm{~V}}\) - plot by using the area under the curve within the specified limits. When an ideal gas is compressed (A) reversibly or (B) irreversibly from volume \(\mathrm{\mathrm{V}_{i}}\) to \(\mathrm{\mathrm{V}_{f}}\) [Here, \(\mathrm{P_{e x}}\) at each stage is equal to \(\left[ {{\rm{V}}\left( {{{\rm{P}}_{{\rm{in }}}}{\rm{ + dp}}} \right)} \right]\). Choose the correct option.
369342
An ideal gas undergoes a cyclic pocess as shown in Figure
\(\Delta {{\text{U}}_{{\text{BC}}}}{\text{ = - 5}}{\mkern 1mu} {\text{k}}{\mkern 1mu} {\text{J}}{\mkern 1mu} {\text{mo}}{{\text{l}}^{{\text{ - 1}}}}{\text{,}}{\mkern 1mu} {{\text{q}}_{{\text{AB}}}}{\text{ = 2}}{\mkern 1mu} {\text{k}}{\mkern 1mu} {\text{J}}{\mkern 1mu} {\text{mo}}{{\text{l}}^{{\text{ - 1}}}}\)
\({{\text{W}}_{{\text{AB}}}}{\text{ = - 5}}\,{\text{k}}\,{\text{J}}\,{\text{mo}}{{\text{l}}^{{\text{ - 1}}}}{\text{,}}\,{{\text{W}}_{{\text{CA}}}}{\text{ = 3}}\,{\text{k}}\,{\text{J}}\,{\text{mo}}{{\text{l}}^{{\text{ - 1}}}}\)
Heat absorbed by the system during process CA is: